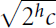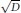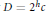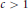Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Mollin, R. A.
and
Srinivasan, A.
2012.
Pell Equations: Non-Principal Lagrange Criteria and Central Norms.
Canadian Mathematical Bulletin,
Vol. 55,
Issue. 4,
p.
774.