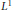Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Yun, Jong-Gug
2006.
Comparison Geometry With L1-Norms of Ricci Curvature.
Canadian Mathematical Bulletin,
Vol. 49,
Issue. 1,
p.
152.