Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-24T18:48:40.135Z Has data issue: false hasContentIssue false
  • English
  • Français

Maximal Sublattices of Finite Distributive Lattices. III: A Conjecture from the 1984 Banff Conference on Graphs and Order

Published online by Cambridge University Press:  20 November 2018

Jonathan David Farley
Jonathan David Farley*
Affiliation:
Institut für Algebra, Johannes Kepler Universität Linz, A-4040 Linz, Österreich, andCenter for International Security and Cooperation, Stanford University, Stanford, California 94305, USAandDepartment of Mathematics and Computer Science, The University of the West Indies, Mona, Kingston 7, Jamaica, andDepartment of Mathematics, California Institute of Technology, Pasadena, California 91125, USAe-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let $L$ be a finite distributive lattice. Let 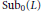$\text{Su}{{\text{b}}_{0}}(L)$ be the lattice

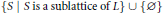$$\{S\,|\,S\,\text{is}\,\text{a sublattice of }L\}\cup \{\phi \}$$

and let 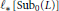${{\ell }_{*}}[\text{Su}{{\text{b}}_{0}}(L)]$ be the length of the shortest maximal chain in 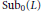$\text{Su}{{\text{b}}_{0}}(L)$. It is proved that if 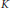$K$ and $L$ are non-trivial finite distributive lattices, then

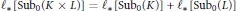$${{\ell }_{*}}[\text{Su}{{\text{b}}_{0}}(K\times L)]={{\ell }_{*}}[\text{Su}{{\text{b}}_{0}}(K)]+{{\ell }_{*}}[\text{Su}{{\text{b}}_{0}}(L)]$$

A conjecture from the 1984 Banff Conference on Graphs and Order is thus proved.

Keywords

06D0506D5006A07(distributive) latticemaximal sublattice(partially) ordered set
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 54 , Issue 2 , 01 June 2011 , pp. 277 - 282
Copyright
Copyright © Canadian Mathematical Society 2011

References

[1] Adams, M. E., Dwinger, P., and Schmid, J., Maximal sublattices of finite distributive lattices. Algebra Universalis 36(1996), no. 4, 488–504.Google Scholar
[2] Birkhoff, G., Lattice Theory. Third edition. American Mathematical Society Colloquium Publications 25, American Mathematical Society, Providence, RI, 1967.Google Scholar
[3] Chen, C. C., Koh, K. M., and Lee, S. C., On the grading numbers of direct products of chains. Discrete Math. 49(1984), no. 1, 21–26.Google Scholar
[4] Davey, B. A. and Priestley, H. A., Introduction to Lattices and Order. Second edition. Cambridge University Press, Cambridge, 2002.Google Scholar
[5] Hashimoto, J., Ideal theory for lattices. Math. Japon. 2(1952), 149–186.Google Scholar
[6] Rival, I., Maximal sublattices of finite distributive lattices. Proc. Amer. Math. Soc. 37(1973), 417–420.Google Scholar
[7] Rival, I., Maximal sublattices of finite distributive lattices. II. Proc. Amer. Math. Soc. 44(1974), 263–268.Google Scholar
[8] Rival, I. (ed.), Graphs and Order. D. Reidel, Dordrecht, 1985.Google Scholar