Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Guralnick, Robert M.
1994.
Invertible preservers and algebraic groups.
Linear Algebra and its Applications,
Vol. 212-213,
Issue. ,
p.
249.
Guralnick, Robert M.
and
Li, Chi-Kwong
1997.
Invertible preservers and algebraic groups III: preservers of unitary similarity (congruence) invariants and overgroups of some unitary subgroups∗.
Linear and Multilinear Algebra,
Vol. 43,
Issue. 1-3,
p.
257.
Li, Chi-Kwong
and
Pierce, Stephen
2001.
Linear Preserver Problems.
The American Mathematical Monthly,
Vol. 108,
Issue. 7,
p.
591.
Bai, Zhaofang
and
Hou, Jinchuan
2002.
Linear maps and additive maps that preserve operators annihilated by a polynomial.
Journal of Mathematical Analysis and Applications,
Vol. 271,
Issue. 1,
p.
139.
Bai, Zhao Fang
and
Hou, Jin Chuan
2005.
Additive Maps Preserving Nilpotent Operators or Spectral Radius.
Acta Mathematica Sinica, English Series,
Vol. 21,
Issue. 5,
p.
1167.
Guterman, A. E.
and
Kuzma, B.
2009.
Preserving Zeros of a Polynomial.
Communications in Algebra,
Vol. 37,
Issue. 11,
p.
4038.
Lim, Ming-Huat
2009.
Linear rank one preservers between spaces of matrices with zero trace.
Linear Algebra and its Applications,
Vol. 430,
Issue. 11-12,
p.
2982.
Fošner, A.
Kuzma, B.
Kuzma, T.
and
Sze, N.-S.
2011.
Maps preserving matrix pairs with zero Jordan product.
Linear and Multilinear Algebra,
Vol. 59,
Issue. 5,
p.
507.
Słowik, Roksana
2013.
Bijective maps of infinite triangular and unitriangular matrices preserving commutators.
Linear and Multilinear Algebra,
Vol. 61,
Issue. 8,
p.
1028.
Hołubowski, W.
and
Stepanov, A. V.
2017.
Bijections preserving commutators and automorphisms of unitriangular groups.
Linear and Multilinear Algebra,
Vol. 65,
Issue. 1,
p.
24.
Costara, Constantin
2020.
Linear maps preserving the polynomial numerical radius of matrices.
Linear Algebra and its Applications,
Vol. 595,
Issue. ,
p.
63.
Kok, Wai Keong
Teoh, L.E.
and
Ng, W.S.
2021.
Rank 2 preservers on symmetric matrices with zero trace.
ITM Web of Conferences,
Vol. 36,
Issue. ,
p.
03002.
Franca, Willian
and
Alves, Magno
2022.
Linear transformations preserving algebraic elements of degree 2.
Linear and Multilinear Algebra,
Vol. 70,
Issue. 19,
p.
4191.
Borisov, Nikita
Julius, Hayden
and
Sikora, Martha
2022.
On maps preserving square roots of idempotent and rank-one nilpotent matrices.
Journal of Algebra and Its Applications,
Vol. 21,
Issue. 06,
Huang, Huajun
and
Tsai, Ming-Cheng
2023.
Matrix Theory - Classics and Advances.
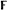 of characteristic zero. For A ∊ Mn, denote by
of characteristic zero. For A ∊ Mn, denote by 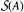 the collection of all matrices in Mn that are similar to A. In this paper we characterize those invertible linear operators ϕ on Mn that satisfy
the collection of all matrices in Mn that are similar to A. In this paper we characterize those invertible linear operators ϕ on Mn that satisfy 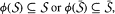 , where
, where 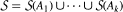 for some given A1,..., Ak ∊ Mn and
for some given A1,..., Ak ∊ Mn and 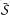 denotes the (Zariski) closure of S. Our theorem covers a result of Howard on linear operators mapping the set of matrices annihilated by a given polynomial into itself, and extends a result of Chan and Lim on linear operators commuting with the function f(x) = xk for a given positive integer k ≥ 2. The possibility of weakening the invertibility assumption in our theorem is considered, a partial answer to a conjecture of Howard is given, and some extensions of our result to arbitrary fields are discussed.
denotes the (Zariski) closure of S. Our theorem covers a result of Howard on linear operators mapping the set of matrices annihilated by a given polynomial into itself, and extends a result of Chan and Lim on linear operators commuting with the function f(x) = xk for a given positive integer k ≥ 2. The possibility of weakening the invertibility assumption in our theorem is considered, a partial answer to a conjecture of Howard is given, and some extensions of our result to arbitrary fields are discussed.

