Hostname: page-component-cd9895bd7-gbm5v Total loading time: 0 Render date: 2024-12-26T23:06:35.467Z Has data issue: false hasContentIssue false
  • English
  • Français

Left orderable surgeries of double twist knots II

Part of: Low-dimensional topology Ordered structures

Published online by Cambridge University Press:  27 August 2020

Vu The Khoi ,
Masakazu Teragaito  and
Anh T. Tran
Vu The Khoi
Affiliation:
Institute of Mathematics, VAST, 18 Hoang Quoc Viet, Hanoi10307, Vietnam e-mail: [email protected]
Masakazu Teragaito
Affiliation:
Department of Mathematics and Mathematics Education, Hiroshima University, 1-1-1 Kagamiyama, Higashi-Hiroshima, Hiroshima7398524, Japan e-mail: [email protected]
Anh T. Tran*
Affiliation:
Department of Mathematical Sciences, The University of Texas at Dallas, Richardson, TX, 75080-3021, USA
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

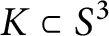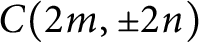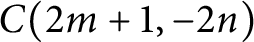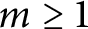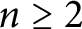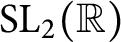A slope r is called a left orderable slope of a knot $K \subset S^3$ if the 3-manifold obtained by r-surgery along K has left orderable fundamental group. Consider double twist knots $C(2m, \pm 2n)$ and $C(2m+1, -2n)$ in the Conway notation, where $m \ge 1$ and $n \ge 2$ are integers. By using continuous families of hyperbolic ${\mathrm {SL}}_2(\mathbb {R})$ -representations of knot groups, it was shown in [8, 16] that any slope in 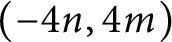$(-4n, 4m)$ (resp. 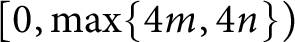$ [0, \max \{4m, 4n\})$ ) is a left orderable slope of 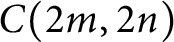$C(2m, 2n)$ (resp. 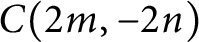$C(2m, - 2n)$ ) and in [6] that any slope in 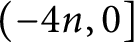$(-4n,0]$ is a left orderable slope of $C(2m+1,-2n)$ . However, the proofs of these results are incomplete, since the continuity of the families of representations was not proved. In this paper, we complete these proofs, and, moreover, we show that any slope in $(-4n, 4m)$ is a left orderable slope of $C(2m+1,-2n)$ detected by hyperbolic ${\mathrm {SL}}_2(\mathbb {R})$ -representations of the knot group.

Keywords

Dehn surgeryleft orderableL-spaceRiley polynomialtwo-bridge knot

MSC classification

Secondary: 06F15: Ordered groups 57M27: Invariants of knots and 3-manifolds 57M25: Knots and links in $S^3$
Type
Article
Information
Canadian Mathematical Bulletin , Volume 64 , Issue 3 , September 2021 , pp. 624 - 637
Check for updates
Copyright
© Canadian Mathematical Society 2020

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

The first author has been partially supported by the Vietnam National Foundation for Science and Technology Development (NAFOSTED) under grant number 101.04-2015.20. The second author has been partially supported by JSPS KAKENHI Grant Number JP20K03587. The third author has been partially supported by a grant from the Simons Foundation (354595).

References

Bergman, G., Right orderable groups that are not locally indicable . Pacific J. Math. 147(1991), no. 2, 243–248.CrossRefGoogle Scholar
Boyer, S., Gordon, C., and Watson, L., On L-spaces and left-orderable fundamental groups . Math. Ann. 356(2013), no. 4, 1213–1245. https://doi.org/10.1007/s00208-012-0852-7 CrossRefGoogle Scholar
Boyer, S., Rolfsen, D., and Wiest, B., Orderable 3-manifold groups . Ann. Inst. Fourier (Grenoble) 55(2005), no. 1, 243–288.CrossRefGoogle Scholar
Culler, M. and Dunfield, N., Orderability and Dehn filling . Geom. Topol. 22(2018), no. 3, 1405–1457. https://doi.org/10.2140/gt.2018.22.1405 CrossRefGoogle Scholar
Gao, X., Orderability of homology spheres obtained by Dehn filling. Preprint, 2018. arXiv:1810.11202 Google Scholar
Gao, X., Slope of orderable Dehn filling of two-bridge knots. Preprint, 2019. arXiv:1912.07468 Google Scholar
Hakamata, R. and Teragaito, M., Left-orderable fundamental groups and Dehn surgery on genus one 2-bridge knots . Algebr. Geom. Topol. 14(2014), no. 4, 2125–2148. https://doi.org/10.2140/agt.2014.14.2125 CrossRefGoogle Scholar
Hakamata, R. and Teragaito, M., Left-orderable fundamental group and Dehn surgery on the knot 52 . Canad. Math. Bull. 57(2014), no. 2, 310–317. https://doi.org/10.4153/CMB-2013-030-2 CrossRefGoogle Scholar
Hatcher, A. and Thurston, W., Incompressible surfaces in 2-bridge knot complements . Invent. Math. 79(1985), no. 2, 225–246. https://doi.org/10.1007/BF01388971 CrossRefGoogle Scholar
Hoste, J. and Shanahan, P., A formula for the A-polynomial of twist knots . J. Knot Theory Ramif. 13(2004), no. 2, 193–209. https://doi.org/10.1142/S0218216504003081 CrossRefGoogle Scholar
Khoi, V., A cut-and-paste method for computing the Seifert volumes . Math. Ann. 326(2003), no. 4, 759–801. https://doi.org/10.1007/s00208-003-0438-5 CrossRefGoogle Scholar
Petersen, K., A-polynomials of a family of two-bridge knots . New York J. Math. 21(2015), 847–881.Google Scholar
Riley, R., Nonabelian representations of 2-bridge knot groups . Quart. J. Math. Oxford Ser. (2) 35(1984), no. 138, 191–208. https://doi.org/10.1093/qmath/35.2.191 CrossRefGoogle Scholar
Tran, A., Left orderable surgeries of double twist knots . J. Math. Soc. Japan. Preprint, 2019. arXiv:1911.03798 Google Scholar
Tran, A., On left-orderable fundamental groups and Dehn surgeries on knots . J. Math. Soc. Jpn. 67(2015), no. 1, 319–338. https://doi.org/10.2969/jmsj/06710319 CrossRefGoogle Scholar