Hostname: page-component-66644f4456-xp6jp Total loading time: 0 Render date: 2025-02-13T07:41:02.571Z Has data issue: true hasContentIssue false
  • English
  • Français

Involutions Fixing Fn ∪ ﹛Indecomposable﹜

Published online by Cambridge University Press:  20 November 2018

Pedro L. Q. Pergher
Pedro L. Q. Pergher*
Affiliation:
Departamento de Matemática, Universidade Federal de São Carlos, Caixa Postal 676, São Carlos, SP 13565- 905, Brazil e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

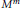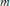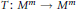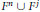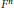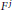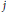Let ${{M}^{m}}$ be an $m$-dimensional, closed and smooth manifold, equipped with a smooth involution $T\,:\,{{M}^{m}}\,\to \,{{M}^{m}}$ whose fixed point set has the form ${{F}^{n}}\,\bigcup \,{{F}^{j}}$, where ${{F}^{n}}$ and ${{F}^{j}}$ are submanifolds with dimensions $n$ and $j$, 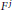${{F}^{j}}$ is indecomposable and 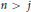$n\,>\,j$. Write 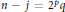$n\,-\,j\,={{2}^{p}}q$, where 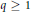$q\,\ge \,1$ is odd and 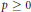$p\,\ge \,0$, and set 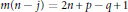$m(n\,-\,j)\,=\,2n\,+\,p\,-q\,+\,1$ if $p\,\le \,q\,+\,1$ and 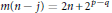$m(n\,-\,j)\,=\,2n\,+\,{{2}^{p-q}}$ if 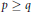$p\,\ge \,q$. In this paper we show that 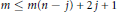$m\,\le \,m(n-j)+2j+1$. 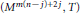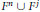Further, we show that this bound is almost best possible, by exhibiting examples $({{M}^{m(n-j)+2j}},\,T)$ where the fixed point set of $T$ has the form ${{F}^{n}}\,\bigcup \,{{F}^{j}}$ described above, for every 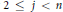$2\,\le \,j\,<\,n$ and 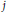$j$ not of the form 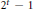${{2}^{t}}\,-\,1$ (for $j\,=\,0$ and 2, it has been previously shown that 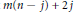$m(n\,-\,j)\,+\,2j$ is the best possible bound). The existence of these bounds is guaranteed by the famous 5/2-theorem of J. Boardman, which establishes that under the above hypotheses $m\,\le \,\frac{5}{2}\,n$.

Keywords

57R85involutionprojective space bundleindecomposable manifoldsplitting principleStiefel– Whitney classcharacteristic number
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 55 , Issue 1 , 01 March 2012 , pp. 164 - 171
Copyright
Copyright © Canadian Mathematical Society 2012

References

[1] Boardman, J. M., On manifolds with involution. Bull. Amer. Math. Soc. 73(1967), 136–138. doi:10.1090/S0002-9904-1967-11683-5Google Scholar
[2] Borel, A., Sur la cohomologie des espaces fibrés principaux et des espaces homogènes de groupes de Lie compacts. Ann. of Math. 57(1953), 115–207. doi:10.2307/1969728Google Scholar
[3] Borel, A. and Hirzebruch, F., Characteristic classes and homogeneous spaces. I. Amer. J. Math. 80(1958), 458–538. doi:10.2307/2372795Google Scholar
[4] Conner, P. E. and Floyd, E. E., Differentiable Periodic Maps. Ergebnisse der Mathematik und ihrer Grenzgebiete 33, Springer-Verlag, Berlin, 1964.Google Scholar
[5] Kelton, S. M., Involutions fixing ℝP j ⋃ Fn . Topology Appl. 142(2004), no. 1-3, 197–203. doi:10.1016/j.topol.2004.02.001Google Scholar
[6] Kelton, S. M., Involutions fixing ℝP j ⋃ Fn, II. Topology Appl. 149(2005), no. 1-3, 217–226. doi:10.1016/j.topol.2004.09.011Google Scholar
[7] Kosniowski, C. and Stong, R. E., Involutions and characteristic numbers. Topology 17(1978), no. 4, 309–330. doi:10.1016/0040-9383(78)90001-0Google Scholar
[8] Pergher, P. L. Q., Bounds on the dimension of manifolds with certain Z 2 fixed sets. Mat. Contemp. 13(1997), 269–275.Google Scholar
[9] Pergher, P. L. Q. and Stong, R. E., Involutions fixing ﹛point﹜ ⋃ Fn . Transform. Groups 6(2001), no. 1, 78–85. doi:10.1007/BF01236063Google Scholar
[10] Pergher, P. L. Q. and Figueira, F. G., Dimensions of fixed point sets of involutions. Arch. Math. (Basel) 87(2006), no. 3, 280–288.Google Scholar
[11] Pergher, P. L. Q. and Figueira, F. G., Involutions fixing Fn ⋃ F2 . Topology Appl. 153(2006), no. 14, 2499–2507. doi:10.1016/j.topol.2005.10.003Google Scholar
[12] Pergher, P. L. Q. and Figueira, F. G., Two commuting involutions fixing Fn ⋃ Fn–1 . Geom. Dedicata 117(2006), 181–193. doi:10.1007/s10711-005-9021-4Google Scholar
[13] Pergher, P. L. Q. and Figueira, F. G., Bounds on the dimension of manifolds with involution fixing Fn ⋃ F2 . Glasg. Math. J. 50(2008), no. 3, 595–604.Google Scholar
[14] Royster, D. C., Involutions fixing the disjoint union of two projective spaces. Indiana Univ. Math. J. 29(1980), no. 2, 267–276. doi:10.1512/iumj.1980.29.29018Google Scholar
[15] Thom, R., Quelques propriétés globales des variétés différentiables. Comment. Math. Helv. 28(1954), 17–86. doi:10.1007/BF02566923Google Scholar