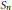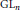Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Woo, Alexander
and
Wyser, Benjamin J.
2015.
Combinatorial Results on (1, 2, 1, 2)-Avoiding GL(p, ℂ) × GL(q, ℂ)-Orbit Closures on GL(p+q, ℂ)/B.
International Mathematics Research Notices,
Vol. 2015,
Issue. 24,
p.
13148.
Woo, Alexander
Wyser, Benjamin
and
Yong, Alexander
2018.
Governing singularities of symmetric orbit closures.
Algebra & Number Theory,
Vol. 12,
Issue. 1,
p.
173.
Gao, Yibo
and
Wang, Anthony
2023.
Consecutive patterns in Coxeter groups.
Journal of Algebra,
Vol. 634,
Issue. ,
p.
650.