Hostname: page-component-f554764f5-sl7kg Total loading time: 0 Render date: 2025-04-08T18:11:43.178Z Has data issue: false hasContentIssue false
  • English
  • Français

Inclusion Relations for New Function Spaces on Riemann Surfaces

Published online by Cambridge University Press:  20 November 2018

Rauno Aulaskari  and
Jouni Rättyä
Rauno Aulaskari
Affiliation:
University of Eastern Finland, Department of Physics and Mathematics, 80101 Joensuu, Finland e-mail: [email protected]@uef.fi
Jouni Rättyä
Affiliation:
University of Eastern Finland, Department of Physics and Mathematics, 80101 Joensuu, Finland e-mail: [email protected]@uef.fi
Rights & Permissions [Opens in a new window]

Abstract.

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

We introduce and study some new function spaces on Riemann surfaces. For certain parameter values these spaces coincide with the classical Dirichlet space, 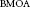$\text{BMOA}$, or the recently defined 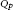${{\text{Q}}_{p}}$ space. We establish inclusion relations that generalize earlier known inclusions between the above-mentioned spaces.

Keywords

0F3530H2530H30Bloch spaceBMOAQpGreen's functionhyperbolic Riemann surface
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 56 , Issue 3 , 01 September 2013 , pp. 466 - 476
Copyright
Copyright © Canadian Mathematical Society 2013

References

[1] Aulaskari, R. and Chen, H., Area inequality and Qp norm. J. Funct. Anal. 221 (2005), no. 1, 1–24. http://dx.doi.org/10.1016/j.jfa.2004.12.007 Google Scholar
[2] Aulaskari, R., He, Y., Ristioja, J., and Zhao, R., Qp spaces on Riemann surfaces. Canad. J. Math. 50 (1998), no. 3, 449–464. http://dx.doi.org/10.4153/CJM-1998-024-4 Google Scholar
[3] Aulaskari, R. and Lappan, P., A criterion for a rotation automorphic function to be normal. Bull. Inst. Math. Acad. Sinica 15 (1987), no. 1, 73–79.Google Scholar
[4] Aulaskari, R., Lappan, P., Xiao, J., and Zhao, R., BMOA(R;m) and capacity density Bloch spaces on hyperbolic Riemann surfaces. Results Math. 29 (1996), no. 3–4, 203–226.Google Scholar
[5] Kobayashi, S., Range sets and BMO norms of analytic functions. Canad. J. Math. 36 (1984), no. 4, 747–755. http://dx.doi.org/10.4153/CJM-1984-042-6 Google Scholar
[6] Kobayashi, S. and Suita, N., Area integrals and Hp norms of analytic functions. Complex Variables Theory Appl. 5 (1986), no. 2–4, 181–188. http://dx.doi.org/10.1080/17476938608814138 Google Scholar
[7] Metzger, T. A., On BMOA for Riemann surfaces. Canad. J. Math. 33 (1981), no. 5, 1255–1260. http://dx.doi.org/10.4153/CJM-1981-094-6 Google Scholar
[8] Minda, D., Bloch and normal functions on general planar regions. In: Holomorphic functions and moduli, Vol. I (Berkeley, CA, 1986), Math. Sci. Res. Inst. Publ., 10, Springer, New York, 1988), pp. 101–110.Google Scholar
[9] Rubel, L. and Timoney, R., An extremal property of the Bloch space. Proc. Amer. Math. Soc. 75 (1979), no. 1, 45–49. http://dx.doi.org/10.1090/S0002-9939-1979-0529210-9 Google Scholar
[10] Stoll, M., A characterization of Hardy-Orlicz spaces on planar domains. Proc. Amer. Math. Soc. 117 (1993), no. 4, 1031–1038. http://dx.doi.org/10.1090/S0002-9939-1993-1124151-8 Google Scholar
[11] Tsuji, M., Potential theory in modern function theory. Maruzen Co., Ltd., Tokyo, 1959).Google Scholar
[12] Zhao, R., An exponential decay characterization of BMOA on Riemann surfaces. Arch. Math. (Basel) 79 (2002), no. 1, 61–66. http://dx.doi.org/10.1007/s00013-002-8285-2 Google Scholar
[13] Zhao, R., The characteristics of BMOA on Riemann surfaces. Kodai Math. J. 15 (1992), no. 2, 221–229. http://dx.doi.org/10.2996/kmj/1138039598 Google Scholar