Hostname: page-component-586b7cd67f-2plfb Total loading time: 0 Render date: 2024-11-28T00:01:10.193Z Has data issue: false hasContentIssue false
  • English
  • Français

Homogeneous Einstein Manifolds with Vanishing 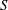$S$ Curvature

Part of: Global differential geometry

Published online by Cambridge University Press:  22 February 2019

Libing Huang  and
Zhongmin Shen
Libing Huang
Affiliation:
School of Mathematical Sciences and LPMC, Nankai University, Tianjin 300071, P. R. China Email: [email protected]
Zhongmin Shen
Affiliation:
Department of Mathematical Sciences, Indiana University-Purdue University, Indianapolis, IN 46202-3216, USA Email: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Infinitely many new Einstein Finsler metrics are constructed on several homogeneous spaces. By imposing certain conditions on the homogeneous spaces, it is shown that the Ricci constant condition becomes an ordinary differential equation. The regular solutions of this equation lead to a two parameter family of Einstein Finsler metrics with vanishing 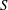$S$ curvature.

Keywords

homogeneous Finsler spaceEinstein manifoldS curvature

MSC classification

Primary: 53C30: Homogeneous manifolds
Secondary: 53C60: Finsler spaces and generalizations (areal metrics)
Type
Article
Information
Canadian Mathematical Bulletin , Volume 62 , Issue 3 , September 2019 , pp. 525 - 537
Copyright
© Canadian Mathematical Society 2019 

Footnotes

The first author is supported by NSFC Grant (no. 11301283, 11571185) and the Fundamental Research Funds for the Central Universities. The second author is supported by NSFC Grant (no. 11671352). This work was done during the first author’s visit to IUPUI.

References

Akbar-Zadeh, H., Generalized Einstein manifolds . J. Geom. Phys. 17(1995), 342–380. https://doi.org/10.1016/0393-0440(94)00052-2.Google Scholar
Arvanitoyeorgos, A., Homogeneous Einstein metrics on Stiefel manifolds . Comment. Math. Univ. Carolin. 37(1996), no. 3, 627–634.Google Scholar
Bao, D., Robles, C., and Shen, Z., Zermelo navigation on Riemannian manifolds . J. Differential Geom. 66(2004), 377–435.Google Scholar
Besse, A., Einstein manifolds . Ergebnisse der Mathematik und ihrer Grenzgebiete (3), 10, Springer-Verlag, Berlin, 1987.Google Scholar
Böhm, C., Wang, M., and Ziller, W., A variational approach for compact homogeneous Einstein manifolds . Geom. Func. Anal. 14(2004), no. 4, 681–733. https://doi.org/10.1007/s00039-004-0471-x.Google Scholar
Bryant, R., Some remarks on Finsler manifolds of constant flag curvature . Houston J. Math. 28(2002), no. 2, 221–262.Google Scholar
Huang, L., On the fundamental equations of homogeneous Finsler spaces . Differential Geom. Appl. 40(2015), 187–208. https://doi.org/10.1016/j.difgeo.2014.12.009.Google Scholar
Huang, L. and Mo, X., Homogeneous Einstein Finsler metrics on (4n + 3)-dimensional spheres . Canad. Math. Bull., to appear. https://doi.org/10.4153/S0008439518000139.Google Scholar
Jensen, G. R., Einstein metrics on principal fibre bundles . J. Differential Geom. 8(1973), 599–614.Google Scholar
Li, B. and Shen, Z., Ricci curvature tensor and non-Riemannian quantities . Canad. Math. Bull. 58(2015), no. 3, 530–537. https://doi.org/10.4153/CMB-2014-063-4.Google Scholar
Wang, H., Huang, L., and Deng, S., Homogeneous Einstein-Randers metrics on spheres . Nonlinear Anal. 74(2011), 6295–6301. https://doi.org/10.1016/j.na.2011.06.008.Google Scholar
Yan, Z. and Deng, S., Homogeneous Einstein (𝛼, 𝛽) metrics on compact simple Lie groups and spheres . Nonlinear Anal. 148(2017), 147–160. https://doi.org/10.1016/j.na.2016.09.016.Google Scholar