Hostname: page-component-cd9895bd7-dk4vv Total loading time: 0 Render date: 2024-12-27T11:35:12.501Z Has data issue: false hasContentIssue false
  • English
  • Français

The Gibbs Phenomenon for [S, αn] Means and [T, αn] Means

Published online by Cambridge University Press:  20 November 2018

V. Swaminathan
V. Swaminathan*
Affiliation:
Department of Mathematics, University of Kerala, Kariavattom, Trivandrum, India
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

The Gibbs phenomenon of the Fourier series 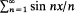for different summability methods has been investigated by various authors. In this note, we study the same for the [S, αn] method of summability introduced by Meir and Sharma [3]. The corresponding result for the [T, αn] method of summability due to Powell [5] can be worked out in exactly the same way.

The elements cnk of the [S, αn] matrix are defined by the relations:

1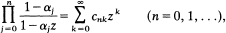

where 0<αn<1. The [S, αn] matrix is regular if and only if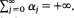

Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 19 , Issue 2 , 01 June 1976 , pp. 209 - 211
Copyright
Copyright © Canadian Mathematical Society 1976

References

1. Ishiguro, K., Zur Gibbschen Erscheinung für das Kreisverfahren. Math. Z. 76, 288-294 (1961).Google Scholar
2. Ishiguro, K., Über das Sα-Verfahren bei Fourier-Reihen. Math. Z. 80, 4–11 (1962).10.1007/BF01162364CrossRefGoogle Scholar
3. Meir, A. and Sharma, A., A generalization of the Sa-summation method. Proc. Camb. Phil. Soc. 67, 61–66 (1970).10.1017/S0305004100057091CrossRefGoogle Scholar
4. Miracle, C. L., The Gibbs phenomenon for Taylor means and for [F, dn] means. Can. J. Math. 12, 660–673 (1960).10.4153/CJM-1960-059-2CrossRefGoogle Scholar
5. Powell, R. E., The T(rn) summability transform. J. Analyse Math. 20, 289–304 (1967).10.1007/BF02786677CrossRefGoogle Scholar