Article contents
Galois Groups of Even Sextic Polynomials
Published online by Cambridge University Press: 12 December 2019
Abstract
Let 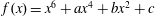 $f(x)=x^{6}+ax^{4}+bx^{2}+c$ be an irreducible sextic polynomial with coefficients from a field
$f(x)=x^{6}+ax^{4}+bx^{2}+c$ be an irreducible sextic polynomial with coefficients from a field 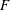 $F$ of characteristic
$F$ of characteristic 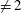 $\neq 2$, and let
$\neq 2$, and let 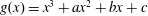 $g(x)=x^{3}+ax^{2}+bx+c$. We show how to identify the conjugacy class in
$g(x)=x^{3}+ax^{2}+bx+c$. We show how to identify the conjugacy class in 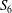 $S_{6}$ of the Galois group of
$S_{6}$ of the Galois group of  $f$ over
$f$ over 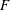 $F$ using only the discriminants of
$F$ using only the discriminants of 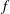 $f$ and
$f$ and  $g$ and the reducibility of a related sextic polynomial. We demonstrate that our method is useful for producing one-parameter families of even sextic polynomials with a specified Galois group.
$g$ and the reducibility of a related sextic polynomial. We demonstrate that our method is useful for producing one-parameter families of even sextic polynomials with a specified Galois group.
Keywords
MSC classification
- Type
- Article
- Information
- Copyright
- © Canadian Mathematical Society 2019
References
- 6
- Cited by



