Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-25T18:53:37.969Z Has data issue: false hasContentIssue false
  • English
  • Français

Functions Universal for all Translation Operators in Several Complex Variables

Published online by Cambridge University Press:  20 November 2018

Frédéric Bayart  and
Paul M Gauthier
Frédéric Bayart
Affiliation:
Université Clermont Auvergne, CNRS, LMBP, F-63000 Clermont-Ferrand, France. e-mail: [email protected]
Paul M Gauthier
Affiliation:
Département demathématiques et de statistique, Université deMontréal, CP-6128A Centreville, Montréal, QC, H3C3J7, Canada. e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

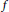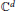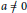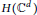We prove the existence of a (in fact many) holomorphic function $f$ in ${{\mathbb{C}}^{d}}$ such that, for any $a\ne 0$, its translations $f(\cdot +na)$ are dense in $H({{\mathbb{C}}^{d}})$.

Keywords

47A1632E20hypercyclic operatortranslation operator
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 60 , Issue 3 , 01 September 2017 , pp. 462 - 469
Copyright
Copyright © Canadian Mathematical Society 2017

References

[1] Bayart, E, Common hypercyclic vectors for high dimensional families of operators. Int. Math. Res. Not. IMRN 2016, no. 21, 6512–6552. http://dx.doi.Org/10.1093/imrn/rnv354 Google Scholar
[2] Bayart, E and Matheron, E., Dynamics of linear operators. Cambridge Tracts in Mathematics, 179, Cambridge University Press, Cambridge, 2009. http://dx.doi.Org/10.1017/CBO9780511581113 Google Scholar
[3] Birkhoff, G. D., Demonstration d'un theoreme elementaire sur les fonctions entieres. C. R. Acad. Sci. Paris 189(1929), 473–475. Google Scholar
[4] Costakis, G. and Sambarino, M., Genericity of wild holomorphic functions and common hypercyclic vectors. Adv. Math. 182(2004), 278–306. http://dx.doi.Org/10.1016/S0001-8708(03)00079-3 Google Scholar
[5] Grosse-Erdmann, K.-G. and Peris, A., Linear chaos. Universitext, Springer, London 2011. http://dx.doi.Org/10.1007/978-1-4471-2170-1 Google Scholar
[6] Stout, E. L., Polynomial convexity. Progress in Mathematics, 261, Birkhauser Boston, Inc., Boston, MA, 2007. Google Scholar
[7] Tsirivas, N., Common hypercyclic functions for translation operators with large gaps. J. Funct. Anal. 272(2017), 2726–2751. http://dx.doi.Org/10.1016/j.jfa.2O16.11.010 Google Scholar
[8] Tsirivas, N., Common hypercyclic functions for translation operators with large gaps. II. arxiv:1412.1963Google Scholar
[9] Tsirivas, N., Existence of common hypercyclic vectors for translation operators. arxiv:1411.7815Google Scholar