Hostname: page-component-cd9895bd7-gvvz8 Total loading time: 0 Render date: 2024-12-25T17:41:04.944Z Has data issue: false hasContentIssue false
  • English
  • Français

Equivariant Forms: Structure and Geometry

Published online by Cambridge University Press:  20 November 2018

Abdelkrim Elbasraoui  and
Abdellah Sebbar
Abdelkrim Elbasraoui
Affiliation:
Centre de recherches mathématiques, Université de Montréal, Montréal, QC H3C 3J7 e-mail: [email protected] CICMA, Concordia University, Montréal, QC H3G 1M8 e-mail: [email protected]
Abdellah Sebbar
Affiliation:
Department of Mathematics and Statistics, University of Ottawa, Ottawa, ON K1N 6N5
Rights & Permissions [Opens in a new window]

Abstract.

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

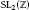In this paper we study the notion of equivariant forms introduced in the authors' previous works. In particular, we completely classify all the equivariant forms for a subgroup of $\text{S}{{\text{L}}_{2\left( \mathbb{Z} \right)}}$ by means of the cross-ratio, weight 2 modular forms, quasimodular forms, as well as differential forms of a Riemann surface and sections of a canonical line bundle.

Keywords

11F11equivariant formsmodular formsSchwarz derivativecross-ratiodifferential forms
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 56 , Issue 3 , 01 September 2013 , pp. 520 - 533
Copyright
Copyright © Canadian Mathematical Society 2013

References

[1] Brady, M., Meromorphic solutions of a system of functional equations involving the modular group. Proc. Amer. Math. Soc. 30 (1971), 271–277. http://dx.doi.org/10.1090/S0002-9939-1971-0280712-5 Google Scholar
[2] El basraoui, A. and Sebbar, A., Rational equivariant forms. Int. J. Number Theory, to appear. http://dx.doi.org/10.1142/S1793042112500571 Google Scholar
[3] Heins, M., On the pseudo-periods of the Weierstrass zeta functions. II. Nagoya Math. J. 30 (1967), 113–119.Google Scholar
[4] Kaneko, M. and Zagier, D., A generalized Jacobi theta function and quasimodular forms. In: The moduli space of curves (Texel Island, 1994), Progr. Math., 129, Birkh¨auser Boston, Boston, MA, 1995, pp. 165–172.Google Scholar
[5] Knopp, M. and Mason, G., Generalized modular forms. J. Number Theory 99 (2003), no. 1, 1–28. http://dx.doi.org/10.1016/S0022-314X(02)00065-3 Google Scholar
[6] Sebbar, A. and Sebbar, A., Equivariant functions and integrals of elliptic functions. GeoDedicata, m., to appear. http://dx.doi.org/10.1007/S10711-011-9688-7 Google Scholar
[7] Smart, J. R., On meromorphic functions commuting with elements of a function group. Proc. Amer. Math. Soc. 33 (1972), 343–348. http://dx.doi.org/10.1090/S0002-9939-1972-0293086-1 Google Scholar