No CrossRef data available.
Article contents
Equivalent Presentations of Modules Over Prüfer Domains
Published online by Cambridge University Press: 20 November 2018
Abstract
Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.
If 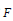 $F$ and
$F$ and 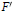 ${F}'$ are free
${F}'$ are free 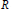 $R$-modules, then
$R$-modules, then 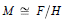 $M\cong F/H$ and
$M\cong F/H$ and 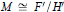 $M\,\cong \,{F}'\,/\,{H}'$ are viewed as equivalent presentations of the
$M\,\cong \,{F}'\,/\,{H}'$ are viewed as equivalent presentations of the 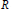 $R$-module
$R$-module 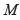 $M$ if there is an isomorphism
$M$ if there is an isomorphism 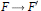 $F\,\to \,{F}'$ which carries the submodule
$F\,\to \,{F}'$ which carries the submodule 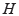 $H$ onto
$H$ onto 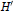 ${H}'$. We study when presentations of modules of projective dimension 1 over Prüfer domains of finite character are necessarily equivalent.
${H}'$. We study when presentations of modules of projective dimension 1 over Prüfer domains of finite character are necessarily equivalent.
Keywords
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 1998
References
1.
Brewer, J. and Klingler, L., Pole assignability and the invariant factor theorem in Prüfer domains and Dedekind domains. J.Algebra 114 (1987), 536–545.Google Scholar
2.
Cohen, J. and Gluck, H., Stacked bases for modules over principal ideal domains. J. Algebra 14 (1970), 493–505.Google Scholar
3.
Erdőos, J., Torsion-free factor groups of free abelian groups and a classification of torsion-free abelian groups. Publ. Math. Debrecen 5 (1957), 172–184.Google Scholar
5.
Fuchs, L., Note on modules of projective dimension one. In: Abelian Group Theory, Gordon and Breach Science Publishers, New York etc., 1986.Google Scholar
6.
Heitmann, R. C. and Levy, L. S., 1 1/2 and 2 generator ideals in Prüfer domains. RockyMountain J. Math. 5 (1975), 361–373.Google Scholar
7.
Hill, P. and Megibben, C., Generalizations of the stacked bases theorem. Trans.Amer.Math. Soc. 312 (1989), 377–402.Google Scholar
8.
Kaplansky, I., Modules over Dedekind rings and valuation rings. Trans. Amer.Math. Soc. 72(1952), 327–340.Google Scholar
9.
Levy, L. S., Invariant factor theorem for Prüfer domains of finite character. J. Algebra 106 (1987), 259–264.Google Scholar


