Article contents
A dichotomy for topological full groups
Published online by Cambridge University Press: 15 September 2022
Abstract
Given a minimal action
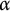 $\alpha $
of a countable group on the Cantor set, we show that the alternating full group
$\alpha $
of a countable group on the Cantor set, we show that the alternating full group
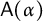 $\mathsf {A}(\alpha )$
is non-amenable if and only if the topological full group
$\mathsf {A}(\alpha )$
is non-amenable if and only if the topological full group
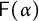 $\mathsf {F}(\alpha )$
is
$\mathsf {F}(\alpha )$
is
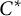 $C^*$
-simple. This implies, for instance, that the Elek–Monod example of non-amenable topological full group coming from a Cantor minimal
$C^*$
-simple. This implies, for instance, that the Elek–Monod example of non-amenable topological full group coming from a Cantor minimal
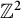 $\mathbb {Z}^2$
-system is
$\mathbb {Z}^2$
-system is
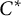 $C^*$
-simple.
$C^*$
-simple.
- Type
- Article
- Information
- Copyright
- © The Author(s), 2022. Published by Cambridge University Press on behalf of The Canadian Mathematical Society
Footnotes
This project has received funding from the European Research Council (ERC) under the European Union’s Horizon 2020 research and innovation program (Grant Agreement No. 817597).
References
- 1
- Cited by



