Hostname: page-component-cd9895bd7-7cvxr Total loading time: 0 Render date: 2024-12-26T16:08:01.403Z Has data issue: false hasContentIssue false
  • English
  • Français

The Densest Packing of Five Spheres in a Cube

Published online by Cambridge University Press:  20 November 2018

J. Schaer
J. Schaer*
Affiliation:
University of Alberta at Calgary
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

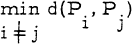The purpose of this paper is to locate five points Pi ( l ≤ i ≤ 5 ) in a closed unit cube C such that is as large as possible, where d(Pi, Pj) denotes the distance i j between Pi and Pj. We prove that this minimum distance cannot exceed 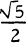(=m, say), and if it is equal to m, then the corresponding configuration is congruent to the set of points shown in fig. 1, namely P1 = A1 (0,0,0), P2 = A8 (1, 1, 1), P3 = B1 (0,1/2,1), P4 = B3 (1/2,1,0) and P5 = B5 (1, 0, 1/2).

Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 9 , Issue 3 , August 1966 , pp. 271 - 274
Copyright
Copyright © Canadian Mathematical Society 1966