Hostname: page-component-cd9895bd7-fscjk Total loading time: 0 Render date: 2024-12-29T12:00:36.320Z Has data issue: false hasContentIssue false
  • English
  • Français

Degree Kirchhoff Index of Bicyclic Graphs

Published online by Cambridge University Press:  20 November 2018

Zikai Tang  and
Hanyuan Deng
Zikai Tang
Affiliation:
College of Mathematics and Computer Science, Hunan Normal University, Changsha, Hunan 410081, P.R. China e-mail: [email protected] e-mail: [email protected]
Hanyuan Deng
Affiliation:
College of Mathematics and Computer Science, Hunan Normal University, Changsha, Hunan 410081, P.R. China e-mail: [email protected] e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

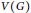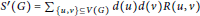Let $G$ be a connected graph with vertex set $V\left( G \right)$.The degree Kirchhoff index of $G$ is defined as ${{S}^{\prime }}\left( G \right)\,=\,\sum{_{\left\{ u,v \right\}\,\subseteq \,V\left( G \right)}d\left( u \right)d\left( v \right)R\left( u,\,v \right)}$, where 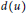$d\left( u \right)$ is the degree of vertex $u$, and 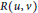$R\left( u,\,v \right)$ denotes the resistance distance between vertices $u$ and $v$. In this paper, we characterize the graphs having maximum and minimum degree Kirchhoff index among all $n$-vertex bicyclic graphs with exactly two cycles.

Keywords

05C1205C35degree Kirchhoff indexresistance distancebicyclic graphextremal graph
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 60 , Issue 1 , 01 March 2017 , pp. 197 - 205
Copyright
Copyright © Canadian Mathematical Society 2017

References

[1] Bonchev, D., Balaban, A. T., Liu, X., and Klein, D. J., Molecular cyclicity and centricity ofpolycyclic graphs: I. Cyclicity based on resistance distances or reciprocal distances. Int. J. Quantum Chem. 50(1994), 1–20.Google Scholar
[2] Chen, H. and Zhang, F., Resistance distance and the normalized Laplacian spectrum. Discrete Appl. Math. 155(2007), no. 5, 654–661. http://dx.doi.Org/10.101 6/j.dam.2006.09.008 Google Scholar
[3] Feng, L. H., Gutman, I., and Yu, G. H., Degree Kirchhoff index ofunicyclic graphs. MATCH Commun. Math. Comput. Chem. 63(2013), no. 3, 629–648.Google Scholar
[4] Feng, L. H., Liu, W. J., Yu, G. H., and Li, S. D., The degree Kirchhoff index of fully loaded unicyclic graphs and cacti. Utilitas Mathematica, to appear.Google Scholar
[5] Klein, D. J. and Randic, M., Resistance distance. J. Math. Chem. 12(1993), 81–95. http://dx.doi.Org/10.1007/BF01164627 Google Scholar
[6] Gutman, I., Selected properties of the Schultz molecular topological index. J. Chem. Inf. Comput. Sci. 34(1994), 1087–1089. http://dx.doi.Org/10.1021/ci00021a009 Google Scholar
[7] Palacios, J. L. and Renom, J. M., Another look at the degree Kirchhoff index. Int. J. Quantum Chem. 111(2011), 3453–3455. http://dx.doi.Org/10.1002/qua.22725 Google Scholar