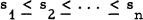Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Narayana, T.V
Mathsen, R.M
and
Sarangi, J
1971.
An algorithm for generating partitions and its applications.
Journal of Combinatorial Theory, Series A,
Vol. 11,
Issue. 1,
p.
54.
Avery, Peter
1980.
Condition for a tournament score sequence to be simple.
Journal of Graph Theory,
Vol. 4,
Issue. 2,
p.
157.
Winston, Kenneth J
and
Kleitman, Daniel J
1983.
On the asymptotic number of tournament score sequences.
Journal of Combinatorial Theory, Series A,
Vol. 35,
Issue. 2,
p.
208.
Takács, Lajos
1986.
Some asymptotic formulas for lattice paths.
Journal of Statistical Planning and Inference,
Vol. 14,
Issue. 1,
p.
123.
Takács, Lajos
1991.
A bernoulli excursion and its various applications.
Advances in Applied Probability,
Vol. 23,
Issue. 3,
p.
557.
Takács, Lajos
1991.
A bernoulli excursion and its various applications.
Advances in Applied Probability,
Vol. 23,
Issue. 3,
p.
557.
Epstein, Richard A.
2010.
The Theory of Gambling and Statistical Logic.
p.
287.
Tsirlin, A. M.
and
Akhremenkov, Al. A.
2019.
Probabilistic Analysis of Tournament Organization Systems.
Automatic Control and Computer Sciences,
Vol. 53,
Issue. 7,
p.
839.
Pozderac, Calvin
Speck, Steven
Feng, Xiaozhou
Huse, David A.
and
Skinner, Brian
2023.
Exact solution for the filling-induced thermalization transition in a one-dimensional fracton system.
Physical Review B,
Vol. 107,
Issue. 4,
Claesson, Anders
Dukes, Mark
Franklín, Atli
and
Stefánsson, Sigurður
2023.
Counting tournament score sequences.
p.
290.
Claesson, Anders
Dukes, Mark
Franklín, Atli
and
Stefánsson, Sigurður
2023.
Counting tournament score sequences.
Proceedings of the American Mathematical Society,