Hostname: page-component-cd9895bd7-q99xh Total loading time: 0 Render date: 2024-12-26T17:57:06.607Z Has data issue: false hasContentIssue false
  • English
  • Français

Compact Commutators of Rough Singular Integral Operators

Published online by Cambridge University Press:  20 November 2018

Jiecheng Chen  and
Guoen Hu
Jiecheng Chen
Affiliation:
Department of Mathematics, Zhejiang Normal University, Jinhua, 321004, P. R. China. e-mail: [email protected]
Guoen Hu
Affiliation:
Department of Applied Mathematics, Zhengzhou Information Science and Technology Institute, P. O. Box 1001-747, Zhengzhou 450002, P. R. China. e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

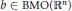Let $b\,\in \,\text{BMO}\left( {{\mathbb{R}}^{n}} \right)$ and 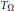${{T}_{\Omega }}$ be the singular integral operator with kernel 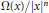$\Omega \left( x \right)/{{\left| x \right|}^{n}}$, where 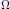$\Omega$ is homogeneous of degree zero, integrable, and has mean value zero on the unit sphere 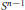${{S}^{n-1}}$. In this paper, using Fourier transform estimates and approximation to the operator 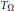${{T}_{\Omega }}$ by integral operators with smooth kernels, it is proved that if 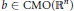$b\,\in \,\text{CMO}\left( {{\mathbb{R}}^{n}} \right)$ and 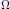$\Omega$ satisfies certain minimal size condition, then the commutator generated by $b$ and 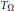${{T}_{\Omega }}$ is a compact operator on 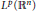${{L}^{p}}\left( {{\mathbb{R}}^{n}} \right)$ for appropriate index $p$. The associated maximal operator is also considered.

Keywords

42B20commutatorsingular integral operatorcompact operatormaximal operator
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 58 , Issue 1 , 01 March 2015 , pp. 19 - 29
Copyright
Copyright © Canadian Mathematical Society 2015

References

[1] Alvarez, J., Bagby, R., Kurtz, D., and Pérez, C., Weighted estimates for commutators of linear operators. Studia Math. 104 (1993), no. 2, 195–209. Google Scholar
[2] Bourdaud, G., Lanze de Cristoforis, M., and Sickel, W., Functional calculus on BMO and related spaces. J. Funct. Anal. 189 (2002), no. 2, 515–538. http://dx.doi.org/10.1006/jfan.2001.3847 Google Scholar
[3] Calderon, A. P. and Zygmund, A., On the existence of certain singular integrals. Acta Math. 88 (1952), 85–139. http://dx.doi.org/10.1007/BF02392130 Google Scholar
[4] Calderon, A. P. and Zygmund, A., On singular integrals. Amer. J. Math. 78 (1956), 289–309. http://dx.doi.org/10.2307/2372517 Google Scholar
[5] Chen, J. and Zhang, C., Boundedness of rough singular integral operators an the Triebel-Lizorkin spaces. J. Math. Anal. Appl. 337 (2008), no. 2, 1048–1052. http://dx.doi.org/10.1016/j.jmaa.2007.04.026 Google Scholar
[6] Chen, Y., Ding, Y., and Wang, X., Compactness of commutators for singular integrals on Morrey spaces. Canad. J. Math. 64 (2012), no. 2, 257–281. http://dx.doi.org/10.4153/CJM-2011-043-1 Google Scholar
[7] Coifman, R., Rochberg, R., and Weiss, G., Factorizaton theorems for Hardy spaces in several variables. Ann. of Math. 103 (1976), no. 3, 611–635. http://dx.doi.org/10.2307/1970954 Google Scholar
[8] Coifman, R. and Weiss, G., Extension of Hardy spaces and their use in analysis. Bull. Amer. Math. Soc. 83 (1977), no. 4, 569–645. http://dx.doi.org/10.1090/S0002-9904-1977-14325-5 Google Scholar
[9] Connett, W. C., Singular integrals near L1 In: Harmonic analysis in Euclidean spaces (Proc. Sympos. Pure Math.,Williams Coll.,Williamstown, Mass., 1978), Part 1, Proc. Sympos. Pure Math., 35, American Mathematical Society, Providence, RI, 1979, pp. 163–165..Google Scholar
[10] Duoandikoetxea, J., Weighted norm inequalities for homogeneous singular integrals. Trans. Amer. Math. Soc. 336 (1993), no. 2, 869–880. http://dx.doi.org/10.1090/S0002-9947-1993-1089418-5 Google Scholar
[11] Duoandikoetxea, J. and Rubio de Francia, J. L., Maximal and singular integral operators via Fourier transform estimates. Invent. Math. 84 (1986), no. 3, 541–561. http://dx.doi.org/10.1007/BF01388746 Google Scholar
[12] Fan, D. and Pan, Y., Singular integral operators with rough kernels supported by subvarieties. Amer. J. Math. 119 (1997), no. 4, 799–839. http://dx.doi.org/10.1353/ajm.1997.0024 Google Scholar
[13] Fan, D., Guo, K. ,and Pan, Y., A note on rough singular integral operators. Math. Inequal. Appl. 2 (1999), no. 1, 73–81. Google Scholar
[14] Grafakos, L., Estimates for maximal singular integrals. Colloq. Math. 96 (2003), no. 2, 167–177. http://dx.doi.org/10.4064/cm96-2-2 Google Scholar
[15] Grafakos, L., Classical Fourier analysis. Second ed., Graduate Texts in Mathematics, 249, Springer, New York, 2008.Google Scholar
[16] Grafakos, L. and Stefanov, A., Lp bounds for singular integrals and maximal singular integrals with rough kernels. Indiana Univ. Math. J. 47 (1998), no. 2, 455–469. Google Scholar
[17] Hu, G., Lp boundedness for the commutator of a homogeneous singular integral operator. Studia Math. 154 (2003), no. 1, 13–27. http://dx.doi.org/10.4064/sm154-1-2 Google Scholar
[18] Hu, G., Lp(Rn)boundedness for a class of g-functions and applications. Hokkaido Math. J. 32 (2003), no. 3, 497–521. http://dx.doi.org/10.14492/hokmj/1350659154 Google Scholar
[19] Hu, G., Sun, Q., and Wang, X., Lp(Rn) bounds for commutators of convolution operators. Colloq. Math. 93 (2002), no. 1, 11–20. http://dx.doi.org/10.4064/cm93-1-2 Google Scholar
[20] Ricci, F. and Weiss, G., A characterization of H1(S-1)In: Harmonic analysis in Euclidean spaces (Proc. Sympos. Pure Math.,Williams Coll.,Williamstown, Mass., 1978), Part 1, Proc. Sympos. Pure Math., 35, American Mathematical Society, Providence, RI, 289–294..Google Scholar
[21] Seeger, A., Singular integral operators with rough convolution kernels. J. Amer. Math. Soc. 9 (1996), no. 1, 95–105. http://dx.doi.org/10.1090/S0894-0347-96-00185-3 Google Scholar
[22] Uchiyama, A., On the compactness of operators of Hankel type. Tohoku Math. J. 30 (1978), no. 1, 163–171. http://dx.doi.org/10.2748/tmj/1178230105 Google Scholar
[23] Watson, D. K., Weighted estimates for singular integrals via Fourier transform estimates. Duke Math. J. 60 (1990), no. 2, 389–399. http://dx.doi.org/10.1215/S0012-7094-90-06015-6 Google Scholar
[24] Yosida, K., Function analysis. Reprint of the sixth (1980) ed., Classics in Mathematics, Springer-Verlag, Berlin, 1995.Google Scholar