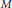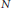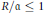Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Abbasi, Ahmad
Shekalgourabi, Hajar Roshan
and
Hassanzadeh-lelekaami, Dawood
2016.
ARTINIANNESS OF LOCAL COHOMOLOGY MODULES.
Honam Mathematical Journal,
Vol. 38,
Issue. 2,
p.
295.
Roshan-Shekalgourabi, H.
and
Hassanzadeh-Lelekaami, D.
2016.
On the generalized local cohomology of minimax modules.
Journal of Algebra and Its Applications,
Vol. 15,
Issue. 08,
p.
1650147.
Irani, Yavar
Bahmanpour, Kamal
and
Ghasemi, Ghader
2019.
Some homological properties of generalized local cohomology modules.
Journal of Algebra and Its Applications,
Vol. 18,
Issue. 12,
p.
1950238.
Divaani-Aazar, K.
Faridian, H.
and
Tousi, M.
2019.
Stable under specialization sets and cofiniteness.
Journal of Algebra and Its Applications,
Vol. 18,
Issue. 01,
p.
1950015.
Vahidi, Alireza
and
Papari-Zarei, Mahdieh
2021.
On the cofiniteness of generalized local cohomology modules with respect to the class of modules in dimension less than a fixed integer.
Communications in Algebra,
Vol. 49,
Issue. 8,
p.
3423.
Aghapournahr, Moharram
and
Hatamkhani, Marziye
2022.
On the stable under specialization sets and cofiniteness of local cohomology.
Communications in Algebra,
Vol. 50,
Issue. 8,
p.
3416.
Nam, Tran Tuan
and
Tri, Nguyen Minh
2024.
Non-vanishing and cofiniteness of generalized local cohomology modules.
Periodica Mathematica Hungarica,
Vol. 88,
Issue. 2,
p.
461.