A Characterization of Bergman Spaces on the Unit Ball of ℂⁿ. II

Abstract

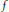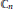It has been shown that a holomorphic function $f$ in the unit ball ${{\mathbb{B}}_{n}}$ of ${{\mathbb{C}}_{n}}$ belongs to the weighted Bergman space 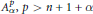$A_{\alpha }^{p},\,p\,>\,n\,+\,1\,+\alpha $, if and only if the function 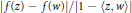$\left| f(z)\,-\,f(w) \right|/\left| 1\,-\,\left\langle z,\,w \right\rangle \right|$ is in ${{L}^{p}}({{\mathbb{B}}_{n}}\,\times \,{{\mathbb{B}}_{n}},\,d{{v}_{\beta }}\,\times \,d{{v}_{\beta }})$, where 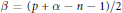$\beta \,=\,(p\,+\,\alpha \,-\,n\,-\,1)/2$ and 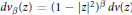$d{{v}_{\beta }}(z)\,=\,{{(1\,-\,{{\left| z \right|}^{2}})}^{\beta }}\,dv(z)$. In this paper we consider the range $0\,<\,p\,<\,n\,+\,1\,+\,\alpha $ and show that in this case, 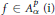$f\,\in \,A_{\alpha }^{p}\,(\text{i})$ (i) if and only if the function 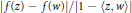$\left| f(z)\,-\,f(w) \right|/\left| 1\,-\,\left\langle z,\,w \right\rangle \right|$ is in 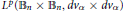${{L}^{p}}({{\mathbb{B}}_{n}}\,\times \,{{\mathbb{B}}_{n}},\,d{{v}_{\alpha }}\,\times \,d{{v}_{\alpha }})$, (ii) if and only if the function 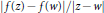$\left| f(z)\,-\,f(w) \right|/\left| z\,-\,w \right|$ is in 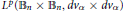${{L}^{p}}({{\mathbb{B}}_{n}}\,\times \,{{\mathbb{B}}_{n}},\,d{{v}_{\alpha }}\,\times \,d{{v}_{\alpha }})$. 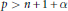We think the revealed difference in the weights for the double integrals between the cases $0\,<\,p\,<\,n\,+\,1\,+\,\alpha $ and $p\,>\,n\,+\,1\,+\,\alpha $ is particularly interesting.