Hostname: page-component-f554764f5-68cz6 Total loading time: 0 Render date: 2025-04-10T21:16:01.828Z Has data issue: false hasContentIssue false
  • English
  • Français

Asymptotic Approximation of an Integral Involving the Normal Distribution*

Published online by Cambridge University Press:  20 November 2018

J. P. McClure  and
R. Wong
J. P. McClure
Affiliation:
Department of Mathematics And Astronomy, University of ManitobaWinnipeg, CanadaR3T 2N2
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

An asymptotic approximation is obtained, as k → ∞, for the integral

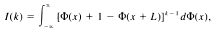
where Φ is the cumulative distribution function for a standard normal random variable, and L is a positive constant. The problem is motivated by a question in statistics, and an outline of'the application is given. Similar methods may be used to approximate other integrals involving the normal distribution.

Keywords

41A6062E20
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 29 , Issue 2 , 01 June 1986 , pp. 167 - 176
Copyright
Copyright © Canadian Mathematical Society 1986

References

1. Olver, F. W. J. Asymptotics and special functions, Academic Press, New York, 1974.Google Scholar
2. Tukey, J.W., Comparing individual means in the analysis of variance, Biometrics, 5 (1949), pp. 99–114.Google Scholar
3. Wong, R., A generalization of Watson's lemma, Ph.D. Thesis, University of Alberta, 1969.Google Scholar