Hostname: page-component-cd9895bd7-jkksz Total loading time: 0 Render date: 2024-12-26T17:48:07.493Z Has data issue: false hasContentIssue false
  • English
  • Français

Annihilators and Power Values of Generalized Skew Derivations on Lie Ideals

Published online by Cambridge University Press:  20 November 2018

Vincenzo De Filippis
Vincenzo De Filippis*
Affiliation:
Department of Mathematics and Computer Science, University of Messina, 98166, Messina, Italy e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let $R$ be a prime ring of characteristic diòerent from $2$, let 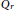${{Q}_{r}}$ be its right Martindale quotient ring, and let $C$ be its extended centroid. Suppose that $F$ is a generalized skew derivation of $R,\,L$ a non-central Lie ideal of 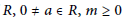$R,\,0\,\ne \,a\,\in \,R,\,m\,\ge \,0$ and 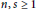$n,\,s\,\ge \,1$ fixed integers. If

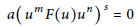$$a{{\left( {{u}^{m}}F\left( u \right){{u}^{n}} \right)}^{s}}\,=\,0$$

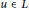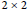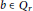for all $u\,\in \,L$, then either $R\,\subseteq \,{{M}_{2}}\left( C \right)$, the ring of $2\,\times \,2$ matrices over $C$, or $m\,=\,0$ and there exists $b\,\in \,{{Q}_{r}}$ such that 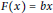$F\left( x \right)\,=\,bx$, for any 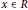$x\,\in \,R$, with 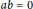$ab\,=\,0$.

Keywords

16W2516N60generalized skew derivationprime ring
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 59 , Issue 2 , 01 June 2016 , pp. 258 - 270
Copyright
Copyright © Canadian Mathematical Society 2016

References

[1] Beidar, K. I., Martindale, W. S. III, and Mikhalev, A. V., Rings with generalized identities. Monographs and Textbooks in Pure and Applied Mathematics, 196, Dekker, New York, 1996.Google Scholar
[2] Bergen, J. and Carini, L., A note on derivations with power central values on a Lie ideal.Pacific J. Math. 132(1988), no. 2, 209–213. http://dx.doi.org/10.2140/pjm.1988.132.209 Google Scholar
[3] Chang, C.-M. and Lin, Y.-C., Derivations on one-sided ideals of prime rings. Tamsui Oxf. J. Math. Sci. 17(2001), no. 2, 139–145.Google Scholar
[4] Chang, J.-C., On the identity h(x) = af(x) + g(x)b. Taiwanese J. Math. 7(2003), no. 1,103-113.Google Scholar
[5] Chang, J.-C., Generalized skew derivations with annihilating Engel conditions. Taiwanese J. Math. 12(2008), no. 7, 1641–1650.Google Scholar
[6] Chang, J.-C., Generalized skew derivations with nilpotent values on Lie ideals. Monatsh.Math. 161(2010), no. 2, 155–160. http://dx.doi.org/10.1007/s00605-009-0136-9 Google Scholar
[7] Chang, J.-C., Generalized skew derivations with power central values on Lie ideals. Comm. Algebra 39(2011), no. 6, 2241–2248.http://dx.doi.org/10.1080/00927872.2010.480957 Google Scholar
[8] Chang, J.-C., Generalized skew derivations with Engel conditions on Lie ideals. Bull. Inst. Math. Acad. Sin. (N.S.) 6(2011), no. 3, 305–320.Google Scholar
[9] Chen, H.-Y., Generalized derivations co centralizing polynomials.Comm. Algebra, 41(2013), no. 7, 2783–2798. http://dx.doi.org/10.1080/00927872.2012.663027 Google Scholar
[10] Cheng, H.-W. and Wei, R., Generalized skew derivations of rings.Adv. Math.(China) 35(2006), no. 1, 237–243.Google Scholar
[11] Chuang, C.-L., GPIs having coefficients in Utumi quotient rings. Proc. Amer. Math. Soc. 103(1988), no. 3, 723–728. http://dx.doi.org/10.1090/S0002-9939-1988-0947646-4 Google Scholar
[12] Chuang, C.-L., Differential identities with automorphisms and antiautomorphisms I. J. Algebra 149(1992), no. 2, 371–404. http://dx.doi.org/10.! 01 6/0021-8693(92)90023-F Google Scholar
[13] Chuang, C.-L., Differential identities with automorphisms and antiautomorphisms II. J. Algebra 160(1993), no. 1, 130–171. http://dx.doi.org/10.1006/jabr.1993.1181 Google Scholar
[14] Chuang, C.-L., Identities with skew derivations. J. Algebra, 224(2000), no. 2, 292–335. http://dx.doi.org/10.1006/jabr.1999.8052 Google Scholar
[15] Chuang, C.-L., Chou, M. -C., and Liu, C. -K., Skew derivations with annihilating Engel conditions. Publ. Math. Debrecen 68(2006), no. 1-2, 161–170.Google Scholar
[16] Chuang, C.-L. and Lee, T. -K., Identities with a single skew derivation. J. Algebra 288(2005), no. 1, 59–77. http://dx.doi.org/10.1016/j.jalgebra.2003.12.032 Google Scholar
[17] Dhara, B. and De Filippis, V., Notes on generalized derivations on Lie ideals in prime rings. Bull. Korean Math. Soc. 46(2009), no. 3, 599–605. http://dx.doi.org/10.4134/BKMS.2009.46.3.599 Google Scholar
[18] Dhara, B., De Filippis, V., and Scudo, G., Power values of generalized derivations with annihilator conditions in prime rings. Mediterr. J. Math. 10(2013), no. 1,123-135. http://dx.doi.org/10.1007/s00009-012-0185-5 Google Scholar
[19] Dhara, B. and Sharma, R. K., Derivations with annihilator conditions in prime rings.Publ. Math. Debrecen 71(2007), no. 1-2, 11–20.Google Scholar
[20] Di Vincenzo, O. M., On the n-th centralizer of a Lie ideal. Boll.Un. Mat. Ital. A 7(1989), no. 1, 77–85.Google Scholar
[21] Jacobson, N., Structure of rings. American Mathematical Society, Providence, RI, 1964.Google Scholar
[22] Herstein, I. N., Topics in ring theory. The University of Chicago Press, Chicago, IL, 1969.Google Scholar
[23] Herstein, I. N., Derivations of prime rings having power central values. In: Algebraists’ homage: paper in ring theory and related topics (New Haven, Conn, 1981), Contemp. Math., 13, American Mathematical Society, Providence, RI, 1982.Google Scholar
[24] Kharchenko, V. K., Generalized identities with automorphisms. Algebra i Logika 14(1975), 215–237, 241; Engl. Transi.: Algebra and Logic 14(1975), 132–148.Google Scholar
[25] Kosan, M. T. and Lee, T. -K., b-generalized derivations of semiprime rings having nilpotent values. J. Austral. Math. Soc. 96(2014), no. 3, 326–337. http://dx.doi.org/10.1017/S1446788713000670 Google Scholar
[26] Lanski, C. and Montgomery, S., Lie structure of prime rings of characteristic 2. Pacific J. Math. 42(1972), 117–135. http://dx.doi.org/10.2140/pjm.1972.42.117 Google Scholar
[27] Lee, T.-K., Generalized skew derivations characterized by acting on zero products. Pacific J. Math. 216(2004), no. 2, 293–301. http://dx.doi.org/10.2140/pjm.2004.216.293 Google Scholar
[28] Lee, T.-K. and Lin, J.-S., A result on derivations.Proc. Amer. Math. Soc. 124(1996), no. 6, 1687–1691. http://dx.doi.org/10.1090/S0002-9939-96-03234-0 Google Scholar
[29] Liu, K.-S., Differential identities and constants of algebraic automorphisms in prime rings.Ph.D. Thesis, National Taiwan University, 2006.Google Scholar
[30] Martindale, W. S. III, Prime rings satisfying a generalized polynomial identity. J. Algebra 12(1969), 576–584. http://dx.doi.org/! 0.101 6/0021-8693(69)90029-5 Google Scholar