Hostname: page-component-cd9895bd7-7cvxr Total loading time: 0 Render date: 2024-12-27T10:39:10.165Z Has data issue: false hasContentIssue false
  • English
  • Français

An Application of Separate Convergence for Continued Fractions to Orthogonal Polynomials

Published online by Cambridge University Press:  20 November 2018

William B. Jones ,
W. J. Thron  and
Nancy J. Wyshinski
William B. Jones
Affiliation:
Department of Mathematics University of Colorado Boulder, CO 80309-0426 U.S.A.
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

It is known that the n-th denominators Qn (α, β, z) of a real J-fraction

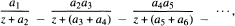
where

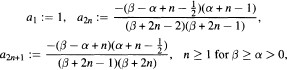
form an orthogonal polynomial sequence (OPS) with respect to a distribution function ψ(t) on ℝ. We use separate convergence results for continued fractions to prove the asymptotic formula

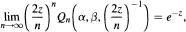
the convergence being uniform on compact subsets of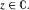

Keywords

30B7033A6534E05
Type
Research Article
Information
Canadian Mathematical Bulletin , Volume 35 , Issue 3 , 01 September 1992 , pp. 381 - 389
Copyright
Copyright © Canadian Mathematical Society 1992

References

1. Baltus, Christopher, and Jones, William B., Truncation Error Bounds for Modified Continued Fractions with Applications to Special Functions, Numer. Math. 55 (1989), 281–307.Google Scholar
2. Chihara, T. S., Introduction to Orthogonal Polynomials , (Mathematics and Its Applications Ser.), Gordon and Breach, New York (1978).Google Scholar
3. Henrici, P., Applied and Computational Complex Analysis , Vol. 2, Special Functions, Integral Transforms, Asymptotics and Continued Fractions, John Wiley and Sons, New York (1977).Google Scholar
4. Ismail, Mourad E.H. and Masson, David R., Two Families of Orthogonal Polynomials Related to Jacobi Polynomials, Rocky Mountain J. of Math. 21(1991), 359–375.Google Scholar
5. Jones, William B., Magnus, Arne, McCabe, John H., and Wyshinski, Nancy J., Asymptotic Properties of a Family of Orthogonal Polynomial Sequences , J. Comput. Appl. Math. 32 (1 & 2) (1990), 143–151.Google Scholar
6. Jones, William B. and Thron, W. J., Continued Fractions: Analytic Theory and Applications , Encyclopedia of Mathematics and its Applications, 11, Addison-Wesley Publishing Company, Reading, MA (1980), distributed now by Cambridge University Press, New York.Google Scholar
7. Njâstad, Olav, A survey of some results on separate convergence of continued fractions , Analytic Theory of Continued Fractions III, (Lisa Jacobsen (ed.)), Lecture Notes in Mathematics 1406, Springer-Verlag, New York (1989), 88–115.Google Scholar
8. Perron, Oskar, Die Lehre Von Den Kettenbriichen , Chelsea Publishing Company, New York (1929).Google Scholar
9. Sleszyriski, J., On the convergence of continued fractions, Zap. mat. otchiel. novoruss. obsch. estest. (Odessa), 8 (1888), 97–127.Google Scholar
10. Thron, W. J., Some results on separate convergence of continued fractions , Computational Methods and Function Theory, Proceedings Valparaiso (1989), Lecture Notes in Mathematics 1435, Springer-Verlag, New York (1990), 191–200.Google Scholar
11. Thron, W. J., Order and type of entire functions arising from separately convergent continued fractions , J. Comput. Appl. Math. 32 (1 & 2) (1990), 273–279.Google Scholar