Hostname: page-component-cd9895bd7-8ctnn Total loading time: 0 Render date: 2024-12-27T09:17:44.620Z Has data issue: false hasContentIssue false
  • English
  • Français

Almost Gorenstein rings arising from fiber products

Part of: Local rings and semilocal rings General theory of categories and functors

Published online by Cambridge University Press:  10 July 2020

Naoki Endo ,
Shiro Goto  and
Ryotaro Isobe
Naoki Endo*
Affiliation:
Department of Mathematics, Purdue University, West Lafayette, IN47907, USA URL: https://www.math.purdue.edu/nendo/
Shiro Goto
Affiliation:
Department of Mathematics, School of Science and Technology, Meiji University, 1-1-1 Higashi-mita, Tama-ku, Kawasaki214-8571, Japane-mail:[email protected]
Ryotaro Isobe
Affiliation:
Department of Mathematics and Informatics, Graduate School of Science and Engineering, Chiba University, 1-33 Yayoi-cho, Inage-ku, Chiba, 263-8522, Japane-mail:[email protected]
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

The purpose of this paper is, as part of the stratification of Cohen–Macaulay rings, to investigate the question of when the fiber products are almost Gorenstein rings. We show that the fiber product $R \times _T S$ of Cohen–Macaulay local rings R, S of the same dimension $d>0$ over a regular local ring T with 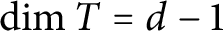$\dim T=d-1$ is an almost Gorenstein ring if and only if so are R and S. In addition, the other generalizations of Gorenstein properties are also explored.

Keywords

fiber productCohen–Macaulay ringGorenstein ringalmost Gorenstein ring

MSC classification

Primary: 13H10: Special types (Cohen-Macaulay, Gorenstein, Buchsbaum, etc.) 13H15: Multiplicity theory and related topics 18A30: Limits and colimits (products, sums, directed limits, pushouts, fiber products, equalizers, kernels, ends and coends, etc.)
Type
Article
Information
Canadian Mathematical Bulletin , Volume 64 , Issue 2 , June 2021 , pp. 383 - 400
Check for updates
Copyright
© Canadian Mathematical Society 2020

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

The first author was partially supported by JSPS Grant-in-Aid for Young Scientists 20K14299 and JSPS Overseas Research Fellowships. The second author was partially supported by JSPS Grant-in-Aid for Scientific Research (C) 16K05112. The third author was partially supported by Grant-in-Aid for JSPS Fellows 20J10517.

References

Ananthnarayan, H., Avramov, L. L., and Moore, W. F., Connected sums of Gorenstein local rings . J. Reine Angew. Math. 667(2012), 149–176. https://doi.org/10.1515/crelle.2011.132Google Scholar
Barucci, V. and Fröberg, R., One-dimensional almost Gorenstein rings . J. Algebra 188(1997), no. 2, 418–442. https://doi.org/10.1006/jabr.1996.6837 CrossRefGoogle Scholar
Brennan, J. P., Herzog, J., and Ulrich, B., Maximally generated maximal Cohen-Macaulay modules . Math. Scand. 61(1987), no. 2, 181–203. https://doi.org/10.7146/math.scand.a-12198 CrossRefGoogle Scholar
Celikbas, E., Celikbas, O., Goto, S., and Taniguchi, N., Generalized Gorenstein Arf rings . Ark. Mat. 57(2019), 35–53. https://doi.org/10.4310/ARKIV.2019.v57.n1.a3 CrossRefGoogle Scholar
Christensen, L. W., Striuli, J., and Veliche, O., Growth in the minimal injective resolution of a local ring . J. Lond. Math. Soc. (2) 81(2010), no. 1, 24–44. https://doi.org/10.1112/jlms/jdp058 CrossRefGoogle Scholar
Chau, T. D. M., Goto, S., Kumashiro, S., and Matsuoka, N., Sally modules of canonical ideals in dimension one and $2$ -AGL rings . J. Algebra 521(2019), 299–330. https://doi.org/10.1016/j.jalgebra.2018.11.023 CrossRefGoogle Scholar
D’Anna, M., A construction of Gorenstein rings . J. Algebra 306(2006), 507–519. https://doi.org/10.1016/j.jalgebra.2005.12.023 CrossRefGoogle Scholar
Ghezzi, L., Goto, S., Hong, J., and Vasconcelos, W. V., Invariants of Cohen-Macaulay rings associated to their canonical ideals . J. Algebra 489(2017), 506–528. https://doi.org/10.1016/j/jalgebra.2017.05.042 CrossRefGoogle Scholar
Goto, S., Isobe, R., and Kumashiro, S., Correspondence between trace ideals and birational extensions with application to the analysis of the Gorenstein property of rings . J. Pure Appl. Algebra 224(2020), 747–767. https://doi.org/10.1016/j.jpaa.2019.06.008 CrossRefGoogle Scholar
Goto, S., Kien, D. V., Matsuoka, N., and Truong, H. L., Pseudo-Frobenius numbers versus defining ideals in numerical semigroup rings . J. Algebra 508(2018), 1–15. https://doi.org/10.1016/j.jalgebra.2018.04.025 CrossRefGoogle Scholar
Goto, S. and Kumashiro, S., On generalized Gorenstein local rings. Preprint, 2019.Google Scholar
Goto, S., Matsuoka, N., and Phuong, T. T., Almost Gorenstein rings. J. Algebra 379(2013), 355–381. https://doi.org/10.1016/j.jalgebra.2013.01.025 CrossRefGoogle Scholar
Goto, S., Matsuoka, N., Taniguchi, N., and Yoshida, K.-i., The almost Gorenstein Rees algebras of parameters . J. Algebra 452(2016), 263–278. https://doi.org/10.1016/j.jalgebra.2015.12.022 CrossRefGoogle Scholar
Goto, S., Matsuoka, N., Taniguchi, N., and Yoshida, K.-i., The almost Gorenstein Rees algebras over two-dimensional regular local rings . J. Pure Appl. Algebra. 220(2016), 3425–3436. https://doi.org/10.1016/j.jpaa.2016.04.007 CrossRefGoogle Scholar
Goto, S., Matsuoka, N., Taniguchi, N., and Yoshida, K.-i., On the almost Gorenstein property in Rees algebras of contracted ideals . Kyoto J. Math. 59(2019), no. 4, 769–785. https://doi.org/10.1215/21562261-2018-0001 CrossRefGoogle Scholar
Goto, S., Matsuoka, N., Taniguchi, N., and Yoshida, K.-i., The almost Gorenstein Rees algebras of ${p}_g$ -ideals, good ideals, and powers of the maximal ideals . Michigan Math. J. 67(2018), 159–174. https://doi.org/1307/mmj/1516330972 CrossRefGoogle Scholar
Goto, S., Nishida, K., and Ozeki, K., Sally modules of rank one . Michigan Math. J. 57(2008), 359–381. https://doi.org/10.1307/mmj/1220879414 CrossRefGoogle Scholar
Goto, S., Rahimi, M., Taniguchi, N., and Truong, H. L., When are the Rees algebras of parameter ideals almost Gorenstein graded rings? Kyoto J. Math. 57(2017), no. 3, 655–666. https://doi.org/10.1215/21562261-2017-0010 CrossRefGoogle Scholar
Goto, S., Takahashi, R., and Taniguchi, N., Almost Gorenstein rings - towards a theory of higher dimension . J. Pure Appl. Algebra 219(2015), 2666–2712. https://doi.org/10.1016/j.jpaa.2014.09.022 CrossRefGoogle Scholar
Goto, S., Takahashi, R., and Taniguchi, N., Ulrich ideals and almost Gorenstein rings . Proc. Amer. Math. Soc. 144(2016), 2811–2823. https://doi.org/10.1090/proc/12970 CrossRefGoogle Scholar
Herzog, J., Hibi, T., and Stamate, D. I., The trace of the canonical module . Israel J. Math. 233(2019), 133–165. https://doi.org/10.1007/s11856-019-1898-y CrossRefGoogle Scholar
Herzog, J. and Kunz, E., Der kanonische Modul eines Cohen-Macaulay-Rings . Lecture Notes in Mathematics, 238, Springer-Verlag, Berlin-New York, 1971.Google Scholar
Higashitani, A., Almost Gorenstein homogeneous rings and their $h$ -vectors . J. Algebra 456(2016), 190–206. https://doi.org/10.1016/j.jalgebra.2016.02.023 CrossRefGoogle Scholar
Kobayashi, T., Syzygies of Cohen-Macaulay modules over one dimensional Cohen-Macaulay local rings. Preprint, 2017. arXiv:1710.02673 Google Scholar
Kobayashi, T. and Takahashi, R., Rings whose ideals are isomorphic to trace ideals . Math. Nachr. 292(2019), no. 10, 2252–2261. https://doi.org/10.1002/mana.201800309 CrossRefGoogle Scholar
Lindo, H., Trace ideals and centers of endomorphism rings of modules over commutative rings . J. Algebra 482(2017), 102–130. https://doi.org/10.1016/j/jalgebra.2016.10.026 CrossRefGoogle Scholar
Lindo, H. and Pande, N., Trace ideals and the Gorenstein property. Preprint, 2018. arXiv:1802.06491.Google Scholar
Matsuoka, N. and Murai, S., Uniformly Cohen-Macaulay simplicial complexes and almost Gorenstein* simplicial complexes . J. Algebra 455(2016), 14–31. https://doi.org/10.1016/j/jalgebra.2016.02.005 CrossRefGoogle Scholar
Miyazaki, M., Almost Gorenstein Hibi rings . J. Algebra 493(2018), 135–149. https://doi.org/10.1016/j.jalgebra.2017.09.033 CrossRefGoogle Scholar
Moore, W. F., Cohomology over fiber products of local rings . J. Algebra 321(2009), no. 3, 758–773. https://doi.org/10.1016/j.jalgebra.2008.10.015 CrossRefGoogle Scholar
Nasseh, S. and Sather-Wagstaff, S., Vanishing of $Ext$ and $Tor$ over fiber products . Proc. Amer. Math. Soc. 145(2017), no. 11, 4661–4674. https://doi.org/10.1090/proc/13633 Google Scholar
Nasseh, S., Sather-Wagstaff, S., Takahashi, R., and VandeBogert, K., Applications and homological properties of local rings with decomposable maximal ideals . J. Pure Appl. Algebra, 223(2019), no. 3, 1272–1287. https://doi.org/10.1016/j.jpaa.2018.06.006 CrossRefGoogle Scholar
Ogoma, T., Fiber products of Noetherian rings . In: Commutative algebra and combinatorics (Kyoto, 1985), Adv. Stud. Pure Math., 11, North-Holland, Amsterdam, 1987, pp. 173–182. https://doi.org/10.2969/aspm/01110173CrossRefGoogle Scholar
Shapiro, J., On a construction of Gorenstein rings proposed by M. D’Anna . J. Algebra 323(2010), 1155–1158. https://doi.org/10.1016/j.jalgebra.2009.12.003 CrossRefGoogle Scholar
Taniguchi, N., On the almost Gorenstein property of determinantal rings . Comm. Algebra 46(2018), 1165–1178. https://doi.org/10.1080/00927872.2017.1339066 CrossRefGoogle Scholar
Vasconcelos, W. V., Hilbert functions, analytic spread, and Koszul homology. In: Commutative algebra. Syzygies, multiplicities, and birational algebra (South Hadley, 1992), Contemp. Math., 159, Amer. Math. Soc., Providence, RI, 1994, pp. 410–422. https://doi.org/10.1090/conm/159/01520Google Scholar