Published online by Cambridge University Press: 20 November 2018
An important conjecture in the theory of Borel sets in non-separable metric spaces is whether any point-countable Borel-additive family in a complete metric space has a  $\sigma $-discrete refinement. We confirm the conjecture for point-countable
$\sigma $-discrete refinement. We confirm the conjecture for point-countable 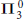 $\Pi _{3}^{0}$-additive families, thus generalizing results of R. W. Hansell and the first author. We apply this result to the existence of Borel measurable selectors for multivalued mappings of low Borel complexity, thus answering in the affirmative a particular version of a question of J. Kaniewski and R. Pol.
$\Pi _{3}^{0}$-additive families, thus generalizing results of R. W. Hansell and the first author. We apply this result to the existence of Borel measurable selectors for multivalued mappings of low Borel complexity, thus answering in the affirmative a particular version of a question of J. Kaniewski and R. Pol.