No CrossRef data available.
Article contents
Explicit symmetric DGLA models of 3-cells
Published online by Cambridge University Press: 01 July 2020
Abstract
We give explicit formulae for differential graded Lie algebra (DGLA) models of 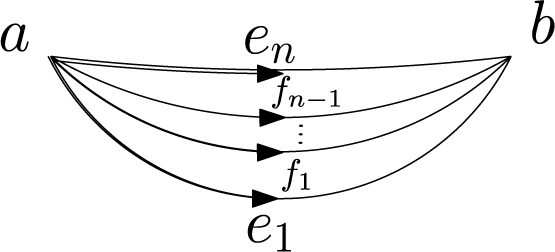 $3$
-cells. In particular, for a cube and an
$3$
-cells. In particular, for a cube and an 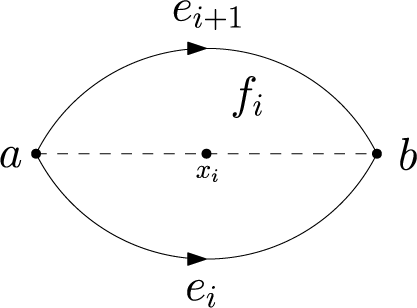 $n$
-faceted banana-shaped
$n$
-faceted banana-shaped  $3$
-cell with two vertices,
$3$
-cell with two vertices, 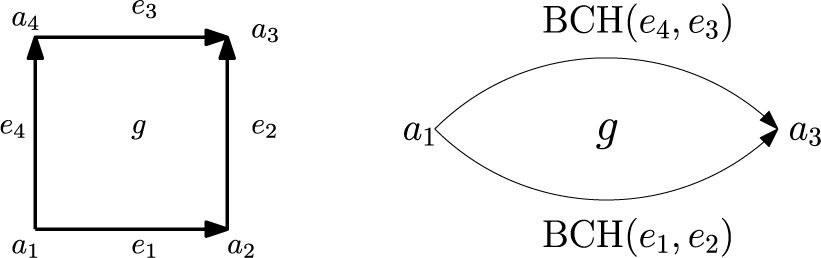 $n$
edges each joining those two vertices, and
$n$
edges each joining those two vertices, and 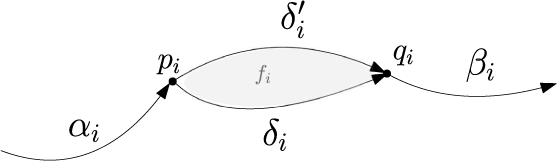 $n$
bi-gon
$n$
bi-gon 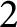 $2$
-cells, we construct a model symmetric under the geometric symmetries of the cell fixing two antipodal vertices. The cube model is to be used in forthcoming work for discrete analogues of differential geometry on cubulated manifolds.
$2$
-cells, we construct a model symmetric under the geometric symmetries of the cell fixing two antipodal vertices. The cube model is to be used in forthcoming work for discrete analogues of differential geometry on cubulated manifolds.
MSC classification
- Type
- Article
- Information
- Copyright
- © Canadian Mathematical Society 2020
Footnotes
This research was supported in part by Grant No. 2016219. from the United States-Israel Binational Science Foundation (BSF). Griniasty is grateful to the Azrieli Foundation for the award of an Azrieli Fellowship.



