Article contents
Unitary Representations of Generalized Symmetric Groups
Published online by Cambridge University Press: 20 November 2018
Extract
In this paper all representations are over the complex field K. The generalized symmetric group S(n, m) of order n!mn is isomorphic to the semi-direct product of the group of n × n diagonal matrices whose rath powers are the unit matrix by the group of all n × n permutation matrices over K. As a permutation group, S(n, m) consists of all permutations of the mn symbols {1, 2, …, mn} which commute with
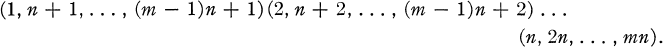
Obviously, S (1, m) is a cyclic group of order m, while S(n, 1) is the symmetric group of order n!. If ci = (i, n+ i, …, (m – 1)n+ i) and
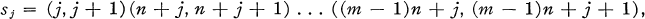
then {c1, c2, …, cn} generate a normal subgroup Q(n) of order mn and {s1, s2, …, sn…1} generate a subgroup S(n) isomorphic to S(n, 1).
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 1969
References
- 4
- Cited by


