Hostname: page-component-586b7cd67f-2plfb Total loading time: 0 Render date: 2024-11-20T18:36:50.269Z Has data issue: false hasContentIssue false
  • English
  • Français

Uniformly Continuous Functionals andM-Weakly Amenable Groups

Published online by Cambridge University Press:  20 November 2018

Brian Forrest  and
Tianxuan Miao
Brian Forrest
Affiliation:
Department of Pure Mathematics, University of Waterloo, WaterlooON N2L 3G1, e-mail: [email protected]
Tianxuan Miao
Affiliation:
Department of Mathematical Sciences, Lakehead University, Thunder Bay, ON P7B 5E1, e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let $G$ be a locally compact group. Let 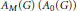${{A}_{M}}\left( G \right)\,\left( {{A}_{0}}\left( G \right) \right)$ denote the closure of 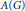$A\left( G \right)$, the Fourier algebra of $G$ in the space of bounded (completely bounded) multipliers of 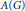$A\left( G \right)$. We call a locally compact group 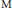$\text{M}$-weakly amenable if ${{A}_{M}}\left( G \right) $ has a bounded approximate identity. We will show that when $G$ is $\text{M}$-weakly amenable, the algebras ${{A}_{M}}\left( G \right) $ and ${{A}_{0}}\left( G \right)$ have properties that are characteristic of the Fourier algebra of an amenable group. Along the way we show that the sets of topologically invariant means associated with these algebras have the same cardinality as those of the Fourier algebra.

Keywords

43A0743A2246J1047L25Fourier algebramultipliersweakly amenableuniformly continuous functionals
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 65 , Issue 5 , 01 October 2013 , pp. 1005 - 1019
Copyright
Copyright © Canadian Mathematical Society 2013

References

[1] Bonsall, F. F. and Duncan, J., Complete normed algebras. Ergebnisse der Mathematik und ihrer Grenzgebiete, 80, Springer-Verlag, New York-Heidelberg, 1973.Google Scholar
[2] Cowling, M. and Haagerup, U., Completely bounded multipliers of the Fourier algebra of a simple Lie group of real rank one. Invent. Math. 96(1989), no. 3, 507–549. http://dx.doi.org/10.1007/BF01393695 Google Scholar
[3] De Cannière, J. and Haagerup, U., Multipliers of the Fourier algebras of some simple Lie groups and their discrete subgroups. Amer. J. Math. 107(1985), no. 2, 455–500. http://dx.doi.org/10.2307/2374423 Google Scholar
[4] Dunkl, C. and Ramirez, D., Weakly almost periodic functionals on the Fourier algebra. Trans. Amer. Math. Soc. 185(1973), 501–514. http://dx.doi.org/10.1090/S0002-9947-1973-0372531-2 Google Scholar
[5] Eymard, P., L’algèbre de Fourier d’un groupe localement compact. Bull. Soc. Math. France 92(1964), 181–236.Google Scholar
[6] Forrest, B. E., Completely bounded multipliers and ideals in A(G) vanishing on closed subgroups. In: Banach algebras and their applications, Contemp. Math., 363, American Mathematical Society, Providence, RI, 2004, pp. 89–94.Google Scholar
[7] Forrest, B. E., Some Banach algebras without discontinuous derivations. Proc. Amer. Math. Soc. 114(1992), no. 4, 965–970. http://dx.doi.org/10.1090/S0002-9939-1992-1068120-4 Google Scholar
[8] Forrest, B., Runde, V., and Spronk, N., Operator amenability of the Fourier algebra in the cb-multiplier norm. Canad. J. Math. 59(2007), no. 5, 966–980. http://dx.doi.org/10.4153/CJM-2007-041-9 Google Scholar
[9] Granirer, E. E., Weakly almost periodic and uniformly continuous functionals on the Fourier algebra of any locally compact group. Trans. Amer. Math. Soc. 189(1974), 371–382. http://dx.doi.org/10.1090/S0002-9947-1974-0336241-0 Google Scholar
[10] Haagerup, U. and Kraus, J., Approximation properties for group C*-algebras and group von Neumann algebras. Trans. Amer. Math. Soc. 344(1994), no. 2, 667–699.Google Scholar
[11] Hu, Z., On the set of topologically invariant means on the von Neumann algebra VN(G). Illinois J. Math. 39(1995), no. 3, 463–490.Google Scholar
[12] Lau, A. T., Uniformly continuous functionals on Banach algebras. Colloq. Math. 51(1987), 195–205.Google Scholar
[13] Lau, A. T., Uniformly continuous functionals on the Fourier algebra of any locally compact group. Trans. Amer. Math. Soc. 251(1979), 39–59. http://dx.doi.org/10.1090/S0002-9947-1979-0531968-4 Google Scholar
[14] Lau and, A. T. Wong, J. C. S., Weakly almost periodic elements in L∞(G) of a locally compact group. Proc. Amer. Math. Soc. 107(1989), no. 4, 1031–1036.Google Scholar
[15] Leptin, H., Sur l’algèbre de Fourier d’un groupe localement compact. C.R. Acad. Sci. Paris Sér A-B 266(1968), A1180–A1182.Google Scholar
[16] Losert, V., Properties of the Fourier algebra that are equivalent to amenability. Proc. Amer. Math. Soc. 92(1984), no. 3, 347–354. http://dx.doi.org/10.1090/S0002-9939-1984-0759651-8 Google Scholar
[17] Ruan, Z.-J., The operator amenability of A(G). Amer. J. Math. 117(1995), no. 6, 1449–1474. http://dx.doi.org/10.2307/2375026 Google Scholar
[18] Rudin, W., Functional analysis. Second Ed., International Series in Pure and Applied Mathematics, McGraw-Hill, Inc., New York, 1991.Google Scholar