Article contents
Unbounded Fredholm Modules and Spectral Flow
Published online by Cambridge University Press: 20 November 2018
Abstract
An odd unbounded (respectively,  $p$-summable) Fredholm module for a unital Banach
$p$-summable) Fredholm module for a unital Banach  $*$-algebra,
$*$-algebra,  $A$, is a pair
$A$, is a pair 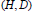 $(H,D)$ where
$(H,D)$ where  $A$ is represented on the Hilbert space,
$A$ is represented on the Hilbert space,  $H$, and
$H$, and 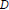 $D$ is an unbounded self-adjoint operator on
$D$ is an unbounded self-adjoint operator on  $H$ satisfying:
$H$ satisfying:
(1) 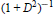 ${{(1+{{D}^{2}})}^{-1}}$ is compact (respectively, Trace
${{(1+{{D}^{2}})}^{-1}}$ is compact (respectively, Trace 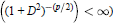 $\left( {{\left( 1+{{D}^{2}} \right)}^{-(p/2)}} \right)<\infty $, and
$\left( {{\left( 1+{{D}^{2}} \right)}^{-(p/2)}} \right)<\infty $, and
(2) 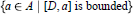 $\{a\in A|\,\,[D,a]\,\,\text{is}\,\,\text{bounded}\}\text{ }$ is a dense
$\{a\in A|\,\,[D,a]\,\,\text{is}\,\,\text{bounded}\}\text{ }$ is a dense  $*$- subalgebra of
$*$- subalgebra of  $A$.
$A$.
If  $u$
is a unitary in the dense
$u$
is a unitary in the dense  $*$-subalgebra mentioned in (2) then
$*$-subalgebra mentioned in (2) then
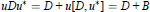 $$uD{{u}^{*}}=D+u[D,{{u}^{*}}]=D+B$$
$$uD{{u}^{*}}=D+u[D,{{u}^{*}}]=D+B$$
where  $B$ is a bounded self-adjoint operator. The path
$B$ is a bounded self-adjoint operator. The path
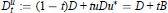 $$D_{t}^{u}:=(1-t)D+tuD{{u}^{*}}=D+tB$$
$$D_{t}^{u}:=(1-t)D+tuD{{u}^{*}}=D+tB$$
is a “continuous” path of unbounded self-adjoint “Fredholm” operators. More precisely, we show that
 $$F_{t}^{u}:=D_{t}^{u}{{\left( 1+{{\left( D_{t}^{u} \right)}^{2}} \right)}^{-\frac{1}{2}}}$$
$$F_{t}^{u}:=D_{t}^{u}{{\left( 1+{{\left( D_{t}^{u} \right)}^{2}} \right)}^{-\frac{1}{2}}}$$
is a norm-continuous path of (bounded) self-adjoint Fredholm operators. The spectral flow of this path 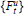 $\left\{ F_{t}^{u} \right\}$ (or
$\left\{ F_{t}^{u} \right\}$ (or  $\{D_{t}^{u}\}$) is roughly speaking the net number of eigenvalues that pass through 0 in the positive direction as
$\{D_{t}^{u}\}$) is roughly speaking the net number of eigenvalues that pass through 0 in the positive direction as  $t$ runs from 0 to 1. This integer,
$t$ runs from 0 to 1. This integer,
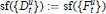 $$\text{sf}\left( \left\{ D_{t}^{u} \right\} \right):=\text{sf}\left( \left\{ F_{t}^{u} \right\} \right),$$
$$\text{sf}\left( \left\{ D_{t}^{u} \right\} \right):=\text{sf}\left( \left\{ F_{t}^{u} \right\} \right),$$
recovers the pairing of the 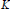 $K$-homology class
$K$-homology class 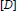 $[D]$ with the
$[D]$ with the  $K$-theory class
$K$-theory class 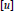 $[u]$.
$[u]$.
We use I.M. Singer's idea (as did E. Getzler in the  $\theta $-summable case) to consider the operator
$\theta $-summable case) to consider the operator  $B$ as a parameter in the Banach manifold,
$B$ as a parameter in the Banach manifold, 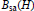 ${{B}_{\text{sa}}}\left( H \right)$, so that spectral flow can be exhibited as the integral of a closed 1-form on this manifold. Now, for
${{B}_{\text{sa}}}\left( H \right)$, so that spectral flow can be exhibited as the integral of a closed 1-form on this manifold. Now, for  $B$ in our manifold, any
$B$ in our manifold, any  $X\,\in \,{{T}_{B}}({{B}_{\text{sa}}}(H))$ is given by an
$X\,\in \,{{T}_{B}}({{B}_{\text{sa}}}(H))$ is given by an 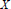 $X$ in
$X$ in 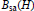 ${{B}_{\text{sa}}}(H)$ as the derivative at
${{B}_{\text{sa}}}(H)$ as the derivative at  $B$ along the curve
$B$ along the curve  $t\,\mapsto \,B\,+\,tX$ in the manifold. Then we show that for
$t\,\mapsto \,B\,+\,tX$ in the manifold. Then we show that for 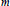 $m$ a sufficiently large half-integer:
$m$ a sufficiently large half-integer:
 $$\alpha (X)=\frac{1}{{{{\tilde{C}}}_{m}}}\text{Tr}\left( X{{\left( 1+{{\left( D+B \right)}^{2}} \right)}^{-m}} \right)$$
$$\alpha (X)=\frac{1}{{{{\tilde{C}}}_{m}}}\text{Tr}\left( X{{\left( 1+{{\left( D+B \right)}^{2}} \right)}^{-m}} \right)$$
is a closed 1-form. For any piecewise smooth path 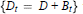 $\left\{ {{D}_{t}}=D+{{B}_{t}} \right\}$ with
$\left\{ {{D}_{t}}=D+{{B}_{t}} \right\}$ with 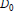 ${{D}_{0}}$ and
${{D}_{0}}$ and 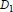 ${{D}_{1}}$unitarily equivalent we show that
${{D}_{1}}$unitarily equivalent we show that
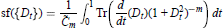 $$\text{sf}\left( \left\{ {{D}_{t}} \right\} \right)=\frac{1}{{{{\tilde{C}}}_{m}}}\int_{\text{0}}^{\text{1}}{\text{T}}\text{r}\left( \frac{d}{dt}\left( {{D}_{t}} \right){{\left( 1+D_{1}^{2} \right)}^{-m}} \right)dt$$
$$\text{sf}\left( \left\{ {{D}_{t}} \right\} \right)=\frac{1}{{{{\tilde{C}}}_{m}}}\int_{\text{0}}^{\text{1}}{\text{T}}\text{r}\left( \frac{d}{dt}\left( {{D}_{t}} \right){{\left( 1+D_{1}^{2} \right)}^{-m}} \right)dt$$
the integral of the 1-form  $\alpha $. If
$\alpha $. If 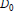 ${{D}_{0}}$ and
${{D}_{0}}$ and 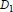 ${{D}_{1}}$ are not unitarily equivalent, wemust add a pair of correction terms to the right-hand side. We also prove a bounded finitely summable version of the form:
${{D}_{1}}$ are not unitarily equivalent, wemust add a pair of correction terms to the right-hand side. We also prove a bounded finitely summable version of the form:
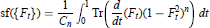 $$\text{sf}\left( \left\{ {{F}_{t}} \right\} \right)=\frac{1}{{{C}_{n}}}\int_{\text{0}}^{\text{1}}{\text{T}}\text{r}\left( \frac{d}{dt}\left( {{F}_{t}} \right){{\left( 1-{{F}_{t}}^{2} \right)}^{n}} \right)dt$$
$$\text{sf}\left( \left\{ {{F}_{t}} \right\} \right)=\frac{1}{{{C}_{n}}}\int_{\text{0}}^{\text{1}}{\text{T}}\text{r}\left( \frac{d}{dt}\left( {{F}_{t}} \right){{\left( 1-{{F}_{t}}^{2} \right)}^{n}} \right)dt$$
for 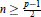 $n\ge \frac{p-1}{2}$ an integer. The unbounded case is proved by reducing to the bounded case via the map
$n\ge \frac{p-1}{2}$ an integer. The unbounded case is proved by reducing to the bounded case via the map 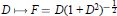 $D\mapsto F=D{{(1+{{D}^{2}})}^{-\frac{1}{2}}}$ We prove simultaneously a type II version of our results.
$D\mapsto F=D{{(1+{{D}^{2}})}^{-\frac{1}{2}}}$ We prove simultaneously a type II version of our results.
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 1998
References
- 51
- Cited by


