Hostname: page-component-586b7cd67f-gb8f7 Total loading time: 0 Render date: 2024-11-24T12:48:16.511Z Has data issue: false hasContentIssue false
  • English
  • Français

Transformations of n-Space which Preserve a Fixed Square-Distance

Published online by Cambridge University Press:  20 November 2018

J. A. Lester
J. A. Lester*
Affiliation:
University of Waterloo, Waterloo, Ontario
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

1. Introduction. Our interest here lies in the following theorem:

THEOREM 1. Assume there is defined on Rn (n ≧ 3) a “square-distance” of the form

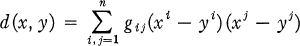
where (gij) is a given symmetric non-singular matrix over the reals and x = (x1, …, xn), y = (y1, …, yn) ∈ Rn. Assume further that f is a bijection ofRnwhich preserves a given fixed square-distance ρ, i.e. d(x, y) = ρ if and only if d(ƒ(x),ƒ(y)) = ρ. Then (unless ρ = 0 and (gij) is positive or negative definite) ƒ(x) = Lx + ƒ(0), where L is a linear bijection ofRnsatisfying d(Lx, Ly) = ±d(x, y) for all x, y ∈ Rn (the – sign is possible if and only if ρ = 0 and (gij) has signature 0).

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 31 , Issue 2 , 01 April 1979 , pp. 392 - 395
Copyright
Copyright © Canadian Mathematical Society 1979

References

1. Beckman, F. S. and Quarles, D. A., Jr., On isometries of Euclidean spaces, Proc. A.M.S. 4 (1953), 810–815.Google Scholar
2. Benz, W., Zur charakterisierung der Lorentz-transformationen, J. Geometry. 9 (1977), 29–37.Google Scholar
3. Borchers, H. J. and Hegerfeldt, G. C., The structure of space-time transformations, Comm. Math. Phys. 28 (1972), 259.Google Scholar
4. Schroder, E. M., Zur kennzeichnung der Lorentz-transformationen, Aequationes Math., to appear.Google Scholar
5. Lester, J. A., Cone preserving mappings for quadric cones over arbitrary fields, Can. J. Math. 29 (1977), 1247–1253.Google Scholar
6. Snapper, E., Troyer, R. J., Metric affine geometry (Academic Press, New York 1971).Google Scholar