1 Introduction
1.1 Systems of tangle equations
A system of tangle equations has the form

where
![]() $T_1\ne T_2$
are rational two-tangles and
$T_1\ne T_2$
are rational two-tangles and
![]() $L_1,L_2$
are links and X is an unknown two-tangle. (From now on, two-tangles will be referred to as “tangles.”) Such systems play an important role in the analysis of entanglement of DNA molecules (see Section 2.3). We study them in the settings of both unframed and framed links, by combining topological methods (surgery theory on double branched covers of links and tangles) and algebraic methods involving the Jones polynomial and the Kauffman bracket.
$L_1,L_2$
are links and X is an unknown two-tangle. (From now on, two-tangles will be referred to as “tangles.”) Such systems play an important role in the analysis of entanglement of DNA molecules (see Section 2.3). We study them in the settings of both unframed and framed links, by combining topological methods (surgery theory on double branched covers of links and tangles) and algebraic methods involving the Jones polynomial and the Kauffman bracket.
We prove foundational results in Sctions 2.2 and 2.3. In particular, we show that every tangle equation has infinitely many solutions when L is not the unlink of two components. We also exhibit systems (1.1) which have an arbitrarily large numbers of solutions.
The Kauffman bracket of a framed two-tangle T is
 $[T]=\begin {pmatrix} [T]_0\\ [T]_\infty \end {pmatrix},$
where
$[T]=\begin {pmatrix} [T]_0\\ [T]_\infty \end {pmatrix},$
where
![]() $[T]_0,[T]_\infty \in \mathbb Z[A^{{\pm 1}}]$
are determined by applying the Kauffman bracket skein relations reducing T to a linear combination
$[T]_0,[T]_\infty \in \mathbb Z[A^{{\pm 1}}]$
are determined by applying the Kauffman bracket skein relations reducing T to a linear combination

(cf. [Reference Eliahou, Kauffman and Thistlethwaite10, Reference Lawrence and Rosenstein27, Reference Tuzun and Sikora41]).
A rational solution of (1.1) is one given by a rational tangle. We prove the following.
Proposition (Proof in Section 8)
Every framed system (1.1) has at most one-framed rational solution.
We propose, more generally, the following.
Conjecture If a framed system (1.1) has a rational solution X, then it is unique (among all solutions X).
The Jones unknot conjecture, henceforth, called the Jones conjecture, states that the Jones polynomial distinguishes all nontrivial knots from the trivial one (see [Reference Jones, Arnold, Atiyah, Lax and Mazur19]). One of the main results of this paper relates the above two conjectures.
Theorem 1.3 (Proof in Section 9)
The Jones conjecture implies Conjecture 1.2.
A stronger version of this statement, formulated in Section 9, shows that because the Jones conjecture holds up to 24 crossings (see [Reference Tuzun and Sikora42]), Conjecture 1.2 holds for tangles up to
![]() $11$
crossings.
$11$
crossings.
The topological meaning of the Jones polynomial is rather obscure, despite much research devoted to it in the last decades. Hence, we find this purely topological consequence of the Jones conjecture intriguing. In particular, this result opens a door to disproving the Jones conjecture through purely topological methods.
We will show in Proposition 2.1 that there is no upper bound on the number of solutions of systems (1.1) (framed and unframed ones).
Conjecture 1.2 does not hold for unframed systems. However, every unframed system has at most two rational solutions (see [Reference Ernst and Sumners11]). Conjecture 1.2 suggests the following unframed version of it.
Conjecture If an unframed system (1.1) has a rational solution
![]() $X,$
then its every solution is rational.
$X,$
then its every solution is rational.
The importance of this conjecture stems from the fact that a counterexample to it may lift to a framed counterexample of Conjecture 1.2; thus, disproving the Jones conjecture. We will prove cases of this conjecture and connect it with the theory of strongly invertible knots in an upcoming work.
1.2 Approach through the surgery theory
Taking double branched covers of tangles and of links translates equations (1.1) into the language of surgery theory of three-manifolds. Apart from the Kauffman bracket, this is the second main source of methods utilized in this paper. In particular, we show the following.
Proposition (Proof in Section 3)
If
![]() $L_1=L_2$
is either a nontrivial rational knot or a nontrivial torus knot, then system (1.1) has no algebraic tangle (framed or unframed) solutions for any
$L_1=L_2$
is either a nontrivial rational knot or a nontrivial torus knot, then system (1.1) has no algebraic tangle (framed or unframed) solutions for any
![]() $T_1,T_2.$
$T_1,T_2.$
(This result settles, in particular, Conjecture 1.2 when
![]() $L_1=L_2$
is a nontrivial rational knot or a torus knot.) We will relate systems of tangle equations to cosmetic surgery conjecture in Section 3.
$L_1=L_2$
is a nontrivial rational knot or a torus knot.) We will relate systems of tangle equations to cosmetic surgery conjecture in Section 3.
1.3 Connection with q-rationals and slopes of essential surfaces
Theorem 1.6 (Proof in Section 7)
For any tangle T,
is well defined (i.e., not
![]() $0/0$
) and independent of the framing of T. Furthermore,
$0/0$
) and independent of the framing of T. Furthermore,
where
![]() $q=-A^4.$
$q=-A^4.$
We call
![]() $\{T\}_q$
the Kauffman bracket ratio of T or the KB ratio for short.
$\{T\}_q$
the Kauffman bracket ratio of T or the KB ratio for short.
Theorem 1.7 (Proof in Section 7)
For any
![]() $x\in \widehat {\mathbb Q}$
,
$x\in \widehat {\mathbb Q}$
,
![]() $\{\langle x\rangle \}_q$
is the q-rational of [Reference Morier-Genoud and Ovsienko33].
$\{\langle x\rangle \}_q$
is the q-rational of [Reference Morier-Genoud and Ovsienko33].
q-Rationals are q-deformations of rational numbers, in the sense that
![]() $\{\langle x\rangle \}_q$
evaluates to x at
$\{\langle x\rangle \}_q$
evaluates to x at
![]() $q=1.$
(They were independently discovered by us in the first version of this paper on arXiv.) Interestingly, much of the theory of continued fractions extends to these q-rational numbers. We believe that our approach to q-rational numbers provides a useful new geometric intuition.
$q=1.$
(They were independently discovered by us in the first version of this paper on arXiv.) Interestingly, much of the theory of continued fractions extends to these q-rational numbers. We believe that our approach to q-rational numbers provides a useful new geometric intuition.
Finally, we relate the Kauffman bracket ratios to slopes of dividing surfaces in tangle complements in Section 10. A surface S properly embedded in the complement of a tangle,
![]() $B^3-T$
, is meridionally incompressible if every two-disk
$B^3-T$
, is meridionally incompressible if every two-disk
![]() $D^2$
in
$D^2$
in
![]() $B^3$
with boundary in S intersecting T transversely precisely once can be deformed to a disk in
$B^3$
with boundary in S intersecting T transversely precisely once can be deformed to a disk in
![]() $S\cup T$
. A surface S is m-essential (for “meridionally essential”) if it is incompressible, meridionally incompressible and not boundary-parallel in
$S\cup T$
. A surface S is m-essential (for “meridionally essential”) if it is incompressible, meridionally incompressible and not boundary-parallel in
![]() $D^3 \smallsetminus T$
(see [Reference Ozawa34, Reference Ozawa35]).
$D^3 \smallsetminus T$
(see [Reference Ozawa34, Reference Ozawa35]).
We say that a loop
![]() $\gamma $
in
$\gamma $
in
![]() $S^2_4$
is dividing if it separates the punctures of
$S^2_4$
is dividing if it separates the punctures of
![]() $S^2_4$
into two groups of two. Such loops are classified by their slope
$S^2_4$
into two groups of two. Such loops are classified by their slope
![]() $s(\gamma )\in \widehat {\mathbb {Q}}={\mathbb {Q}}\cup \{\infty \}$
which can be defined by lifting
$s(\gamma )\in \widehat {\mathbb {Q}}={\mathbb {Q}}\cup \{\infty \}$
which can be defined by lifting
![]() $\gamma $
to the torus being the double cover of
$\gamma $
to the torus being the double cover of
![]() $S^2$
branched over the four ends of T (see Section 10).
$S^2$
branched over the four ends of T (see Section 10).
If the boundary of an m-essential surface contains a dividing loop
![]() $\gamma ,$
we call S dividing and we call
$\gamma ,$
we call S dividing and we call
![]() $s(\gamma )$
the slope
$s(\gamma )$
the slope
![]() $s(S)$
of S. (Then it is easy to see that all other dividing loops of
$s(S)$
of S. (Then it is easy to see that all other dividing loops of
![]() $\partial S$
must be parallel.) This definition is inspired by that in [Reference Ozawa35], where the slopes are defined for algebraic tangles.
$\partial S$
must be parallel.) This definition is inspired by that in [Reference Ozawa35], where the slopes are defined for algebraic tangles.
Conjecture Every dividing m-essential surface in the complement of any tangle T has slope
![]() $\{T\}_1$
.
$\{T\}_1$
.
For the above reason, we call
![]() $\{T\}_1\in \widehat {\mathbb {Q}}$
the algebraic slope of T. This conjecture is related to [Reference Ozawa35, Question 3.4] which asks more broadly if the slopes of such surfaces are determined by T.
$\{T\}_1\in \widehat {\mathbb {Q}}$
the algebraic slope of T. This conjecture is related to [Reference Ozawa35, Question 3.4] which asks more broadly if the slopes of such surfaces are determined by T.
Theorem 1.9 (Proof in Section 10)
Our algebraic slope coincides with Ozawa’s slope for algebraic tangles. In other words, the above conjecture holds for algebraic tangles.
2 Preliminaries
2.1 Rational and algebraic tangles and links
Throughout, two-tangles will be called tangles for brevity. They are proper tame embeddings of one-manifolds into
![]() $D^3=D^2\times (-1,1)$
with ends at NE, SE, SW, NW points of
$D^3=D^2\times (-1,1)$
with ends at NE, SE, SW, NW points of
![]() $\partial D^2,$
where
$\partial D^2,$
where
![]() $D^2$
is a compact disk identified with
$D^2$
is a compact disk identified with
![]() $D^2\times \{0\}.$
Tangles are considered up to isotopies fixing
$D^2\times \{0\}.$
Tangles are considered up to isotopies fixing
![]() $\partial D^2$
. A two-string tangle is one which consists of two strings only (no loops). The
$\partial D^2$
. A two-string tangle is one which consists of two strings only (no loops). The
![]() $\langle -1\rangle , \langle 0\rangle , \langle 1\rangle $
and
$\langle -1\rangle , \langle 0\rangle , \langle 1\rangle $
and
![]() $\langle \infty \rangle $
tangles and the tangle addition are depicted in Figure 1.
$\langle \infty \rangle $
tangles and the tangle addition are depicted in Figure 1.

Figure 1: The
![]() $-1, 0, 1$
and
$-1, 0, 1$
and
![]() $\infty $
tangles and the tangle addition
$\infty $
tangles and the tangle addition
![]() $T+T'$
. (We follow here Conway’s notation [Reference Conway and Leech7]. Kauffman’s and his collaborators’ papers use opposite signs, e.g., [Reference Kauffman, Lambropoulou and Calvo24].)
$T+T'$
. (We follow here Conway’s notation [Reference Conway and Leech7]. Kauffman’s and his collaborators’ papers use opposite signs, e.g., [Reference Kauffman, Lambropoulou and Calvo24].)
The result of adding n tangles
![]() $\langle 1\rangle $
(resp.
$\langle 1\rangle $
(resp.
![]() $\langle -1\rangle $
) together is denoted by
$\langle -1\rangle $
) together is denoted by
![]() $\langle n\rangle $
(resp.
$\langle n\rangle $
(resp.
![]() $\langle -n\rangle $
), for
$\langle -n\rangle $
), for
![]() $n=1,2,3,\ldots $
These tangles, together with
$n=1,2,3,\ldots $
These tangles, together with
![]() $\langle 0\rangle $
, are called integral.
$\langle 0\rangle $
, are called integral.
The mirror image
![]() $-T$
of T is obtained by switching all crossings of T. The tangle rotation
$-T$
of T is obtained by switching all crossings of T. The tangle rotation
![]() $R(T)$
is the
$R(T)$
is the
![]() $90^\circ $
clockwise rotation and the tangle inversion is the tangle rotation followed by the mirror image.
$90^\circ $
clockwise rotation and the tangle inversion is the tangle rotation followed by the mirror image.
All tangles obtained from the integral ones by the operations of addition and rotation are called algebraic. (This class is closed under the mirror image and inversion.) Among them are rational tangles defined as follows:
By
![]() $\langle a_n,..,a_1\rangle $
, we denote the tangle obtained from
$\langle a_n,..,a_1\rangle $
, we denote the tangle obtained from
![]() $\langle 0\rangle $
by adding
$\langle 0\rangle $
by adding
![]() $\langle a_1\rangle $
followed by the inversion, then by adding
$\langle a_1\rangle $
followed by the inversion, then by adding
![]() $\langle a_2\rangle $
followed by the inversion, and so on, until this construction is finished by adding
$\langle a_2\rangle $
followed by the inversion, and so on, until this construction is finished by adding
![]() $a_n$
at the end, as in Figure 2 (left). Tangles of this form, for
$a_n$
at the end, as in Figure 2 (left). Tangles of this form, for
![]() $a_1,\ldots ,a_n\in \mathbb Z$
, are called rational because as observed by Conway [Reference Conway and Leech7] (and proved in [Reference Burde and Zieschang6, Reference Kauffman, Lambropoulou and Calvo24, Reference Montesinos32])
$a_1,\ldots ,a_n\in \mathbb Z$
, are called rational because as observed by Conway [Reference Conway and Leech7] (and proved in [Reference Burde and Zieschang6, Reference Kauffman, Lambropoulou and Calvo24, Reference Montesinos32])
 $$ \begin{align*}\langle a_n,..,a_1\rangle \to a_n+\frac{1}{a_{n-1}+\frac{1}{\ddots +\frac{1}{a_1}}}\end{align*} $$
$$ \begin{align*}\langle a_n,..,a_1\rangle \to a_n+\frac{1}{a_{n-1}+\frac{1}{\ddots +\frac{1}{a_1}}}\end{align*} $$
defines a bijection between rational tangles (up to tangle isotopy) and
![]() $\widehat {\mathbb {Q}}={\mathbb {Q}}\cup \{\infty \}$
. Under this bijection, tangle inversion corresponds to the fraction inversion,
$\widehat {\mathbb {Q}}={\mathbb {Q}}\cup \{\infty \}$
. Under this bijection, tangle inversion corresponds to the fraction inversion,
![]() $x\to 1/x,$
and the mirror image operation corresponds to the negation,
$x\to 1/x,$
and the mirror image operation corresponds to the negation,
![]() $x\to -x.$
$x\to -x.$
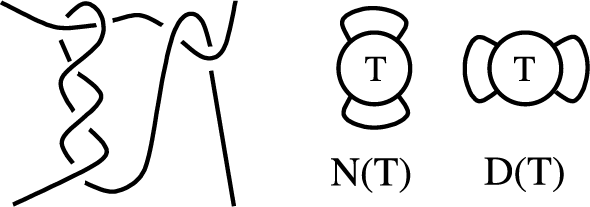
Figure 2: Rational tangle
![]() $\langle -2,-3,2\rangle $
, and the numerator, and the denominator closures.
$\langle -2,-3,2\rangle $
, and the numerator, and the denominator closures.
This association does not preserve addition.
By applying the numerator or the denominator closure (defined in Figure 2) to a rational tangle, we obtain a rational link, also referred to as a two-bridge link or four-plat.
2.2 Tangle equations
A tangle equation has the form
where a rational tangle T and a link L are given and the tangle X is unknown. Finding all solutions of (2.1) is usually exceedingly difficult.
Let us denote by B and D the three-balls in which X and T lie, and let S be the four-punctured sphere
![]() $S^2_4=\partial B-X=\partial D-T.$
For any
$S^2_4=\partial B-X=\partial D-T.$
For any
![]() $\phi $
in the mapping class group,
$\phi $
in the mapping class group,
![]() $MCG(S^2_4),$
the action of
$MCG(S^2_4),$
the action of
![]() $\phi $
on
$\phi $
on
![]() $S^2_4$
extends to maps of
$S^2_4$
extends to maps of
![]() $B-X$
and of
$B-X$
and of
![]() $D-T$
to
$D-T$
to
![]() $B-X'$
and
$B-X'$
and
![]() $D-T'$
, respectively, such that
$D-T'$
, respectively, such that
![]() $N(X+T)=N(X'+T')$
. In other words,
$N(X+T)=N(X'+T')$
. In other words,
![]() $MCG(S^2_4)$
acts on all pairs
$MCG(S^2_4)$
acts on all pairs
![]() $(X,T)$
while preserving
$(X,T)$
while preserving
![]() $N(X+T)$
. (The orbits of that action on tangles are the isotopy classes of tangles moving their endpoints around.) We call pairs
$N(X+T)$
. (The orbits of that action on tangles are the isotopy classes of tangles moving their endpoints around.) We call pairs
![]() $(X,T)$
and
$(X,T)$
and
![]() $\phi (X,T),$
for
$\phi (X,T),$
for
![]() $\phi \in MCG(S^2_4)$
equivalent. Since all rational tangles belong to a single orbit of
$\phi \in MCG(S^2_4)$
equivalent. Since all rational tangles belong to a single orbit of
![]() $MCG(S^2_4)$
, this equivalence defines a
$MCG(S^2_4)$
, this equivalence defines a
![]() $1$
–
$1$
–
![]() $1$
correspondences between solutions of tangle equations (2.1) for different T’s.
$1$
correspondences between solutions of tangle equations (2.1) for different T’s.
Recall that a tangle is two-string if it has no loops.
Proposition (1) If L is the unlink of two components, then Eq. (2.1) has infinitely many solutions but only one two-string one.
(2) If L is a knot or a nontrivial link then Eq. (2.1) has infinitely many two-string solutions.
Proof Since every tangle equation is equivalent to one with
![]() $T=\langle 0\rangle ,$
we assume that for the proof. Hence, clearly it has at least one solution, X. For L the unlink,
$T=\langle 0\rangle ,$
we assume that for the proof. Hence, clearly it has at least one solution, X. For L the unlink,
![]() $X=\langle 0\rangle $
.
$X=\langle 0\rangle $
.

Figure 3: A satellite of a long trefoil.
Assume that L is not the unlink now. For any long knot K, let
![]() $Sat_X(K)$
be its satellite with X as a companion tangle, as in Figure 3. Then
$Sat_X(K)$
be its satellite with X as a companion tangle, as in Figure 3. Then
![]() $N(Sat_X(K))=N(X)$
. Hence, if X is a solution of Eq. (2.1), then
$N(Sat_X(K))=N(X)$
. Hence, if X is a solution of Eq. (2.1), then
![]() $Sat_X(K)$
is a solution as well. Since
$Sat_X(K)$
is a solution as well. Since
![]() $Sat_X(K)$
and
$Sat_X(K)$
and
![]() $Sat_X(K')$
are non-isotopic for different K and
$Sat_X(K')$
are non-isotopic for different K and
![]() $K'$
(here, we assume that
$K'$
(here, we assume that
![]() $X\ne \langle 0\rangle $
), there are infinitely many different tangles of this form.
$X\ne \langle 0\rangle $
), there are infinitely many different tangles of this form.
For a nontrivial rational L [Reference Ernst and Sumners11, Theorem 2.2] shows infinitely many rational solutions to Eq. (2.1).
Finally, we remark that Proposition 2.1 does not hold if T is allowed to be nonrational. For example, Eq. (2.1) has no solutions for certain pretzel tangles T and L the unknot, since one can show that some pretzel tangles do not embed into the unknot. (That fact is an easy application of Proposition 5.1.)
2.3 Systems of tangle equations
A system of tangle equations has the form (1.1) or, equivalently,
 $$ \begin{align*}\begin{cases} N(X+T_1)=L_1,\\ N(X+T_2)=L_2, \end{cases}\end{align*} $$
$$ \begin{align*}\begin{cases} N(X+T_1)=L_1,\\ N(X+T_2)=L_2, \end{cases}\end{align*} $$
where
![]() $T_1\ne T_2$
.
$T_1\ne T_2$
.
Systems of tangle equations play a crucial role in the analysis of entanglement of DNA molecules. The reason for that is that certain enzymes (called recombinase) separate circular DNA substrate molecules into two tangles:
![]() $T_1$
, consisting of the part of the DNA molecule bound to the enzyme, and the other part, X, not bound to the enzyme. Then the enzyme replaces
$T_1$
, consisting of the part of the DNA molecule bound to the enzyme, and the other part, X, not bound to the enzyme. Then the enzyme replaces
![]() $T_1$
by a tangle
$T_1$
by a tangle
![]() $T_2$
. The substrate knot
$T_2$
. The substrate knot
![]() $L_1$
is controlled by the experiment. The link
$L_1$
is controlled by the experiment. The link
![]() $L_2$
is observable in the experiment. Since tangles
$L_2$
is observable in the experiment. Since tangles
![]() $T_1$
and
$T_1$
and
![]() $T_2$
are known, DNA recombination processes lead to systems (1.1), where X is the unknown (see, e.g., [Reference Arsuaga, Diao, Vazquez, Benham, Harvey, Olson, Sumners and Swigon1, Reference Buck and Mauricio5, Reference Darcy8, Reference Darcy and Scharein9, Reference Ernst and Sumners11, Reference Sumners38, Reference Sumners, Ernst, Cozzarelli and Spengler39, Reference Vetcher, Lushnikov, Navarra-Madsen, Scharein, Lyubchenko, Darcy and Levene43, Reference Yang44] for further discussion of such systems).
$T_2$
are known, DNA recombination processes lead to systems (1.1), where X is the unknown (see, e.g., [Reference Arsuaga, Diao, Vazquez, Benham, Harvey, Olson, Sumners and Swigon1, Reference Buck and Mauricio5, Reference Darcy8, Reference Darcy and Scharein9, Reference Ernst and Sumners11, Reference Sumners38, Reference Sumners, Ernst, Cozzarelli and Spengler39, Reference Vetcher, Lushnikov, Navarra-Madsen, Scharein, Lyubchenko, Darcy and Levene43, Reference Yang44] for further discussion of such systems).
The action
![]() $MCG(S^2_4)$
on tangles of the previous section extends onto an action on triples
$MCG(S^2_4)$
on tangles of the previous section extends onto an action on triples
![]() $(X,T_1,T_2)$
. By analogy, we call triples
$(X,T_1,T_2)$
. By analogy, we call triples
![]() $(X,T_1,T_2)$
and
$(X,T_1,T_2)$
and
![]() $\phi (X,T_1,T_2)$
equivalent for any
$\phi (X,T_1,T_2)$
equivalent for any
![]() $\phi \in MCG(S^2_4).$
This equivalence defines a
$\phi \in MCG(S^2_4).$
This equivalence defines a
![]() $1$
–
$1$
–
![]() $1$
correspondences between solutions of systems of tangle equations (1.1) for equivalent pairs
$1$
correspondences between solutions of systems of tangle equations (1.1) for equivalent pairs
![]() $(T_1,T_2)$
and
$(T_1,T_2)$
and
![]() $(T_1',T_2')$
.
$(T_1',T_2')$
.
Proposition For every pair
![]() $(T_1,T_2)$
not equivalent to
$(T_1,T_2)$
not equivalent to
![]() $(\langle 0 \rangle , \langle k\rangle )$
for
$(\langle 0 \rangle , \langle k\rangle )$
for
![]() $k\in \mathbb Z\cup \{\infty \}$
and for every
$k\in \mathbb Z\cup \{\infty \}$
and for every
![]() $n>0$
there are links
$n>0$
there are links
![]() $L_1$
and
$L_1$
and
![]() $L_2$
such that (1.1) has at least n solutions.
$L_2$
such that (1.1) has at least n solutions.
A Montesinos tangle is a sum of rational ones,
![]() $R_1+ \cdots +R_k$
. The numerator closure of a Montesinos tangle is a Montesinos link. In the above proposition, one can further assume that
$R_1+ \cdots +R_k$
. The numerator closure of a Montesinos tangle is a Montesinos link. In the above proposition, one can further assume that
![]() $L_1, L_2$
and the solutions X are Montesinos.
$L_1, L_2$
and the solutions X are Montesinos.
Proof of Proposition 2.3
By utilizing an equivalence, we can assume that equations (1.1) have form
for some
![]() $L_1,L_2$
and
$L_1,L_2$
and
![]() $T=\langle x_0\rangle ,$
for some
$T=\langle x_0\rangle ,$
for some
![]() $x_0\in \widehat {\mathbb {Q}} -{\mathbb {Z}}.$
Let
$x_0\in \widehat {\mathbb {Q}} -{\mathbb {Z}}.$
Let
for some
![]() $x_1\in \widehat {\mathbb {Q}},$
where there are
$x_1\in \widehat {\mathbb {Q}},$
where there are
![]() $i T$
’s on the left and
$i T$
’s on the left and
![]() $2n-i T$
’s on the right. By a cyclic symmetry,
$2n-i T$
’s on the right. By a cyclic symmetry,
![]() $N(X_i)$
coincide for
$N(X_i)$
coincide for
![]() $i=0, \ldots , n-1$
and also
$i=0, \ldots , n-1$
and also
![]() $N(X_i+T)$
coincide for
$N(X_i+T)$
coincide for
![]() $i=0, \ldots , n-1$
.
$i=0, \ldots , n-1$
.
We claim that choosing
![]() $x_1\in \widehat {\mathbb {Q}}$
so that
$x_1\in \widehat {\mathbb {Q}}$
so that
![]() $x_1\ne x_0$
mod
$x_1\ne x_0$
mod
![]() $1$
guarantees that all
$1$
guarantees that all
![]() $X_1,\ldots ,X_n$
are distinct. Indeed, the links
$X_1,\ldots ,X_n$
are distinct. Indeed, the links
![]() $N(X_i+\langle x_0\rangle )$
are Montesinos. By the classification of Montesinos links [Reference Bonahon4], they are all distinct if
$N(X_i+\langle x_0\rangle )$
are Montesinos. By the classification of Montesinos links [Reference Bonahon4], they are all distinct if
![]() $x_0\ne x_1$
mod
$x_0\ne x_1$
mod
![]() $1.$
$1.$
In the case of systems of tangle equations, the conditions for the existence and uniqueness or finiteness of solutions seem unknown in general. We propose the following.
Conjecture Every system (1.1) have finitely many solutions X only.
We are going to see later (e.g., in Section 8) that the uniqueness of solutions is easier to analyze in the context of framed tangles and links.
3 Surgery methods, cosmetic surgery conjecture
We denote by
![]() $\Sigma (T)$
the double cover of the three-ball
$\Sigma (T)$
the double cover of the three-ball
![]() $B^3$
branched along a tangle T in it. Similarly,
$B^3$
branched along a tangle T in it. Similarly,
![]() $\Sigma (L)$
denotes the double cover of
$\Sigma (L)$
denotes the double cover of
![]() $S^3$
branched along a link L. Since the double branched cover of a rational tangle T is a solid torus, every solution X of (1.1) defines a knot (the core of
$S^3$
branched along a link L. Since the double branched cover of a rational tangle T is a solid torus, every solution X of (1.1) defines a knot (the core of
![]() $\Sigma (T)$
) in
$\Sigma (T)$
) in
![]() $\Sigma (L).$
$\Sigma (L).$
As an application of surgery methods, we obtain Proposition 1.5 which we recall here.
Proposition 1.5 If
![]() $L_1=L_2$
is either a nontrivial rational knot or a nontrivial torus knot, then system (1.1) has no algebraic tangle (framed or unframed) solutions for any
$L_1=L_2$
is either a nontrivial rational knot or a nontrivial torus knot, then system (1.1) has no algebraic tangle (framed or unframed) solutions for any
![]() $T_1,T_2.$
$T_1,T_2.$
Proof Suppose that X is an algebraic tangle solution to
for
![]() $T_1\ne T_2.$
By the above discussion, there is a nontrivial surgery of
$T_1\ne T_2.$
By the above discussion, there is a nontrivial surgery of
![]() $\Sigma (L)$
yielding
$\Sigma (L)$
yielding
![]() $\Sigma (L)$
. If L is rational then
$\Sigma (L)$
. If L is rational then
![]() $\Sigma (L)$
is a lens space and if L is a torus knot then
$\Sigma (L)$
is a lens space and if L is a torus knot then
![]() $\Sigma (L)$
is Seifert with base the teo-sphere and three exceptional fibers (see, e.g., [Reference Jang and Paoluzzi18, Section 3.1]). Either way, it is irreducible and atoroidal. Furthermore,
$\Sigma (L)$
is Seifert with base the teo-sphere and three exceptional fibers (see, e.g., [Reference Jang and Paoluzzi18, Section 3.1]). Either way, it is irreducible and atoroidal. Furthermore,
![]() $\Sigma (X)$
is a graph manifold and, hence, non-hyperbolic. Hence, [Reference Matignon31, Corollary 1.4] implies that
$\Sigma (X)$
is a graph manifold and, hence, non-hyperbolic. Hence, [Reference Matignon31, Corollary 1.4] implies that
![]() $\Sigma (T_i)$
lies in a three-ball. Since
$\Sigma (T_i)$
lies in a three-ball. Since
![]() $\Sigma (L)\ne S^3,$
the boundary of that ball is incompressible in
$\Sigma (L)\ne S^3,$
the boundary of that ball is incompressible in
![]() $\Sigma (X),$
making it reducible. Since X is rational or prime, that is impossible by [Reference Lickorish29, Theorem 5].
$\Sigma (X),$
making it reducible. Since X is rational or prime, that is impossible by [Reference Lickorish29, Theorem 5].
As observed in the proof above, every solution of (3.1) yields a cosmetic surgery on
![]() $\Sigma (L)$
. Such surgeries are rare and are subject of the cosmetic surgery conjecture, (see [Reference Gordon15, Reference Kirby25]). A special case of that conjecture is the nugatory crossing conjecture, asserting that if a change of a sign of a crossing c in a knot diagram D results in an isotopic knot then c is nugatory, that is K has form below (see [Reference Balm, Friedl, Kalfagianni and Powell2, Reference Balm and Kalfagianni3, Reference Kalfagianni21, Reference Lidman and Moore30, Reference Torisu40]).
$\Sigma (L)$
. Such surgeries are rare and are subject of the cosmetic surgery conjecture, (see [Reference Gordon15, Reference Kirby25]). A special case of that conjecture is the nugatory crossing conjecture, asserting that if a change of a sign of a crossing c in a knot diagram D results in an isotopic knot then c is nugatory, that is K has form below (see [Reference Balm, Friedl, Kalfagianni and Powell2, Reference Balm and Kalfagianni3, Reference Kalfagianni21, Reference Lidman and Moore30, Reference Torisu40]).
We say that a tangle
![]() $X\subset B^3$
is unlinked if it is a two-string tangle whose components can be isotoped so that they do not intersect each other. (They can be all obtained by knotting the arcs of
$X\subset B^3$
is unlinked if it is a two-string tangle whose components can be isotoped so that they do not intersect each other. (They can be all obtained by knotting the arcs of
![]() $\langle 0\rangle $
or of
$\langle 0\rangle $
or of
![]() $\langle \infty \rangle $
.) The following is straightforward.
$\langle \infty \rangle $
.) The following is straightforward.
Proposition Nugatory crossing conjecture is equivalent to the following statement: If
![]() $N(X+\langle 1\rangle )=N(X+\langle -1\rangle )$
is a knot, then X is unlinked.
$N(X+\langle 1\rangle )=N(X+\langle -1\rangle )$
is a knot, then X is unlinked.
Proof (1)
![]() $\Rightarrow $
Suppose the nugatory crossing conjecture holds and
$\Rightarrow $
Suppose the nugatory crossing conjecture holds and
is a knot K. Then the crossing in
![]() $\langle \pm 1\rangle $
is nugatory in K. By the nugatory crossing conjecture, K consists of two arcs, each in a disjoint ball, as in Figure 4. Since these balls can be placed in the three-disk
$\langle \pm 1\rangle $
is nugatory in K. By the nugatory crossing conjecture, K consists of two arcs, each in a disjoint ball, as in Figure 4. Since these balls can be placed in the three-disk
![]() $B^3$
to form X, the statement follows.
$B^3$
to form X, the statement follows.

Figure 4: A nugatory crossing in knot. Disks denote one-tangles.
![]() $\Leftarrow $
Any knot with a nugatory crossing can be realized as
$\Leftarrow $
Any knot with a nugatory crossing can be realized as
![]() $N(X+\langle 1\rangle )$
where the nugatory crossing is in
$N(X+\langle 1\rangle )$
where the nugatory crossing is in
![]() $\langle 1\rangle .$
Since X is unlinked the statement follows.
$\langle 1\rangle .$
Since X is unlinked the statement follows.
It is worth noting that
![]() $N(X+\langle 1\rangle )=N(X+\langle -1\rangle )$
cannot be a link for a two-string X.
$N(X+\langle 1\rangle )=N(X+\langle -1\rangle )$
cannot be a link for a two-string X.
Finally, the following example shows that the assumption of nontriviality of
![]() $L_1=L_2$
in Proposition 1.5 is essential. It will be useful later.
$L_1=L_2$
in Proposition 1.5 is essential. It will be useful later.
Lemma 3.2
![]() $X=\langle \infty \rangle $
and
$X=\langle \infty \rangle $
and
![]() $\langle -1/2\rangle $
are the only solutions to
$\langle -1/2\rangle $
are the only solutions to
where U denotes the unknot.
Proof The numerator closure
![]() $N(\cdot )$
and the operation
$N(\cdot )$
and the operation
![]() $N(\cdot +\langle 1\rangle )$
, on the level of the double covers correspond to two different Dehn fillings of
$N(\cdot +\langle 1\rangle )$
, on the level of the double covers correspond to two different Dehn fillings of
![]() $\Sigma (X).$
Each of them yields the double cover of
$\Sigma (X).$
Each of them yields the double cover of
![]() $S^3$
branched along U, i.e.,
$S^3$
branched along U, i.e.,
![]() $S^3.$
By a theorem of Gordon and Luecke [Reference Gordon and Luecke16, Theorem 2],
$S^3.$
By a theorem of Gordon and Luecke [Reference Gordon and Luecke16, Theorem 2],
![]() $\Sigma (X)$
must be a solid torus and, hence, X is rational,
$\Sigma (X)$
must be a solid torus and, hence, X is rational,
![]() $X=\langle p/q\rangle .$
$X=\langle p/q\rangle .$
By a theorem of Schubert,
only if
![]() $p=1$
(see [Reference Kauffman, Lambropoulou and Calvo24, Reference Schubert37]). Since
$p=1$
(see [Reference Kauffman, Lambropoulou and Calvo24, Reference Schubert37]). Since
![]() $N(\langle \frac {1}{q}\rangle +\langle 1\rangle )=U$
, it is easy to see that q is either
$N(\langle \frac {1}{q}\rangle +\langle 1\rangle )=U$
, it is easy to see that q is either
![]() $0$
or
$0$
or
![]() $-1/2.$
$-1/2.$
4 Framed tangles and systems of equations
Framed links are tame embeddings of annuli
![]() $S^1\times I\cup \cdots \cup S^1\times I$
into
$S^1\times I\cup \cdots \cup S^1\times I$
into
![]() $\mathbb R^3$
, where I is an open interval. Similarly, framed tangles are proper tame embeddings
$\mathbb R^3$
, where I is an open interval. Similarly, framed tangles are proper tame embeddings
where
![]() $J_1, J_2$
are closed intervals and the end arcs
$J_1, J_2$
are closed intervals and the end arcs
![]() $\partial J_1\times I$
,
$\partial J_1\times I$
,
![]() $\partial J_2\times I$
lie disjointly in
$\partial J_2\times I$
lie disjointly in
![]() $\partial D^2=\partial (D^2\times \{0\})$
each containing a different point from among
$\partial D^2=\partial (D^2\times \{0\})$
each containing a different point from among
![]() $NE, SE, SW, NW$
in
$NE, SE, SW, NW$
in
![]() $\partial D^2$
. Clearly, every link diagram and tangle diagram defines a framed link or tangle with its framing parallel to the page. We require that every framed link and tangle can be represented in that way. (Hence, components with a half-twist framing are not allowed.)
$\partial D^2$
. Clearly, every link diagram and tangle diagram defines a framed link or tangle with its framing parallel to the page. We require that every framed link and tangle can be represented in that way. (Hence, components with a half-twist framing are not allowed.)

Figure 5: Balanced Reidemeister moves. (Diagrams have blackboard framing.)
For our purposes, it will be convenient to consider framed links and tangles up to balanced isotopy given by the balanced Reidemeister moves, presented in Figure 5. Note that this is a somewhat more flexible isotopy than the standard isotopy of framed links or tangles.
A framed rational tangle is a rational tangle with an arbitrary framing. Given a diagram D of a tangle or a link, we will denote by
![]() $D^n$
the framed diagram obtained from the page framing of D by adding
$D^n$
the framed diagram obtained from the page framing of D by adding
![]() $|n|$
positive or negative twists, depending on the sign of n. Note that location of these twists does not matter up to balanced Reidemeister moves.
$|n|$
positive or negative twists, depending on the sign of n. Note that location of these twists does not matter up to balanced Reidemeister moves.
Definitions of tangle addition and of numerator and denominator closures generalize immediately to framed tangles. Consequently, systems (1.1) can be considered in the context of framed tangles and links.
4.1 Systems of framed tangle equations
Framed links and tangles seem more appropriate for modeling DNA molecules which are double stranded. Additionally, we will see soon, that it sometimes easier to solve tangle equations in the framed setting and framed solutions inform on unframed ones.
Let us assume that
![]() $T_1, T_2$
are framed rational tangles now. As before, we assume that
$T_1, T_2$
are framed rational tangles now. As before, we assume that
![]() $T_1, T_2$
are unequal as unframed tangles. (Note that if
$T_1, T_2$
are unequal as unframed tangles. (Note that if
![]() $T_1$
and
$T_1$
and
![]() $T_2$
in (1.1) differ by framing twists only, then by adjusting the framing of
$T_2$
in (1.1) differ by framing twists only, then by adjusting the framing of
![]() $L_2$
, one can reduce (1.1) to a form in which
$L_2$
, one can reduce (1.1) to a form in which
![]() $T_1=T_2$
as framed tangles. In that form, (1.1) is either inconsistent or reduces to a single equation.)
$T_1=T_2$
as framed tangles. In that form, (1.1) is either inconsistent or reduces to a single equation.)
Note that Proposition 2.1 easily extends to framed equations.
Given an unframed system (1.1), its framing is a choice of a framing for
![]() $L_1,L_2,T_1,T_2.$
Let us consider the following framed version of system (3.2) which will be useful later:
$L_1,L_2,T_1,T_2.$
Let us consider the following framed version of system (3.2) which will be useful later:
for some
![]() $n,m\in \mathbb Z,$
where as defined above,
$n,m\in \mathbb Z,$
where as defined above,
![]() $U^n$
denotes the unknot with
$U^n$
denotes the unknot with
![]() $|n|$
twists of framing, positive or negative, depending on the sign of n.
$|n|$
twists of framing, positive or negative, depending on the sign of n.
Lemma 4.1
(1) If
![]() $m=n+1,$
then
$m=n+1,$
then
![]() $\langle \infty \rangle ^n$
is the only solution to (4.1).
$\langle \infty \rangle ^n$
is the only solution to (4.1).
(2) If
![]() $m=n-3,$
then
$m=n-3,$
then
![]() $\langle -1/2\rangle ^{n+2}$
is the only solution to (4.1).
$\langle -1/2\rangle ^{n+2}$
is the only solution to (4.1).
(3) If
![]() $m-n$
is neither
$m-n$
is neither
![]() $1$
or
$1$
or
![]() $-3,$
then (4.1) has no framed solution.
$-3,$
then (4.1) has no framed solution.
Proof Suppose that X satisfies the above equations. Then stripped of its framing, it is either
![]() $X=\langle \infty \rangle $
or
$X=\langle \infty \rangle $
or
![]() $\langle -1/2\rangle $
, by Lemma 3.2. Since
$\langle -1/2\rangle $
, by Lemma 3.2. Since

where the dashed square marks the tangle
![]() $\langle \infty \rangle ^f,$
for some
$\langle \infty \rangle ^f,$
for some
![]() $f\in \mathbb Z$
, the tangle
$f\in \mathbb Z$
, the tangle
![]() $X=\langle \infty \rangle $
lifts to a framed solution only iff
$X=\langle \infty \rangle $
lifts to a framed solution only iff
![]() $m-n=1$
and that solution is
$m-n=1$
and that solution is
![]() $\langle \infty \rangle ^f$
, where
$\langle \infty \rangle ^f$
, where
![]() $f=n$
. (For reader’s convenience, we marked the signs of crossings in the diagrams.) Similarly,
$f=n$
. (For reader’s convenience, we marked the signs of crossings in the diagrams.) Similarly,

shows that
![]() $X=\langle -1/2\rangle $
lifts to a framed solution only iff
$X=\langle -1/2\rangle $
lifts to a framed solution only iff
![]() $m-n=-3$
and that solution is
$m-n=-3$
and that solution is
![]() $\langle -1/2\rangle ^f$
for
$\langle -1/2\rangle ^f$
for
![]() $f=n-2$
.
$f=n-2$
.
For
![]() $m-n\ne 1, -3$
, these equations are contradictory.
$m-n\ne 1, -3$
, these equations are contradictory.
Note that every framed system (1.1) defines an unframed one and every framed solution descends to an unframed one. Lemmas 3.2 and 4.1 show that not every solution to the unframed system can be lifted to a framed one. In fact, there may be no framing of (1.1) for which all unframed solutions can be framed.
5 The Kauffman bracket of a tangle
The Kauffman bracket
![]() $[L]\in \mathbb Z[A^{\pm 1}]$
is an invariant of framed unoriented links L up to isotopy, satisfying the skein relations
$[L]\in \mathbb Z[A^{\pm 1}]$
is an invariant of framed unoriented links L up to isotopy, satisfying the skein relations

(with the blackboard framing), where
![]() $\delta =-A^2-A^{-2},$
normalized so that the bracket of the trivially framed unknot
$\delta =-A^2-A^{-2},$
normalized so that the bracket of the trivially framed unknot
![]() $U^0$
is
$U^0$
is
![]() $[U^0]=1.$
$[U^0]=1.$
By the above skein relations, each framed tangle can be expressed as

where
![]() $[T]_0,[T]_\infty \in \mathbb Z[A^{{\pm 1}}]$
are uniquely defined. Recall that we call the vector
$[T]_0,[T]_\infty \in \mathbb Z[A^{{\pm 1}}]$
are uniquely defined. Recall that we call the vector
 $[T]=\begin {pmatrix} [T]_0\\ [T]_\infty \end {pmatrix}$
the Kauffman bracket of
$[T]=\begin {pmatrix} [T]_0\\ [T]_\infty \end {pmatrix}$
the Kauffman bracket of
![]() $T.$
$T.$
Kauffman brackets of tangles provide a criterium for embedding them into links.
The KB determinant of T denoted by
![]() $det_{KB}(T)\in \mathbb Q[A^{\pm 1}]$
is
$det_{KB}(T)\in \mathbb Q[A^{\pm 1}]$
is
![]() $gcd([T]_0,[T]_\infty )$
in
$gcd([T]_0,[T]_\infty )$
in
![]() $\mathbb Q[A^{\pm 1}]$
. Note that it is well defined for unframed tangles up to a multiplicative factor of
$\mathbb Q[A^{\pm 1}]$
. Note that it is well defined for unframed tangles up to a multiplicative factor of
![]() $A^n$
, for
$A^n$
, for
![]() $n\in \mathbb Z$
.
$n\in \mathbb Z$
.
Proposition A necessary condition for a tangle T embedding into a link L is that
![]() $det_{KB}(T)$
divides
$det_{KB}(T)$
divides
![]() $[L]$
in
$[L]$
in
![]() $\mathbb Q[A^{\pm 1}]$
.
$\mathbb Q[A^{\pm 1}]$
.
This result generalizes the main result of [Reference Krebes26] which treats
![]() $A=e^{\pi i/4}$
case (see also [Reference Ruberman36]). (This idea was utilized for the trivial L in [Reference Tuzun and Sikora41].) More generally, a necessary condition is that
$A=e^{\pi i/4}$
case (see also [Reference Ruberman36]). (This idea was utilized for the trivial L in [Reference Tuzun and Sikora41].) More generally, a necessary condition is that
![]() $[L]\in \mathbb Z[A^{\pm 1}]$
lies in the ideal
$[L]\in \mathbb Z[A^{\pm 1}]$
lies in the ideal
![]() $\langle [T]_0,[T]_\infty \rangle \triangleleft \mathbb Z[A^{\pm 1}]$
.
$\langle [T]_0,[T]_\infty \rangle \triangleleft \mathbb Z[A^{\pm 1}]$
.
Proof of Proposition 5.1

and, therefore, a tangle T embeds into a link L only if
![]() $gcd([T]_0,[T]_\infty )$
divides
$gcd([T]_0,[T]_\infty )$
divides
![]() $[L]$
in
$[L]$
in
![]() $\mathbb Q[A^{\pm 1}].$
(Formally, one needs framed tangles and links for that, but since framing affects the bracket by a multiplicative factor of
$\mathbb Q[A^{\pm 1}].$
(Formally, one needs framed tangles and links for that, but since framing affects the bracket by a multiplicative factor of
![]() $(-A^3)^n$
which is a unit in
$(-A^3)^n$
which is a unit in
![]() $\mathbb Q[A^{\pm 1}]$
, that statement makes sense in the unframed setting as well.)
$\mathbb Q[A^{\pm 1}]$
, that statement makes sense in the unframed setting as well.)
Note that
![]() $[T]_0, [T]_\infty $
are integers for that
$[T]_0, [T]_\infty $
are integers for that
![]() $A=e^{\pi i/4}$
. The topological meaning of
$A=e^{\pi i/4}$
. The topological meaning of
![]() $gcd( [T]_0, [T]_\infty )$
for that A seems to be an interesting question. It appears to be related to the order of the torsion of the double branched cover of T, however, it is not always equal to it, as observed in [Reference Ruberman36].
$gcd( [T]_0, [T]_\infty )$
for that A seems to be an interesting question. It appears to be related to the order of the torsion of the double branched cover of T, however, it is not always equal to it, as observed in [Reference Ruberman36].
The following discussion will be useful later. We say that an unframed two-string tangle T is of type
![]() $0$
,
$0$
,
![]() $\pm 1$
, or
$\pm 1$
, or
![]() $\infty $
if it can be reduced to one of these rational tangles through crossing changes. Note that each tangle is of precisely one of these three types, depending on connections between its endpoints. Type
$\infty $
if it can be reduced to one of these rational tangles through crossing changes. Note that each tangle is of precisely one of these three types, depending on connections between its endpoints. Type
![]() $\pm 1$
has two subtypes:
$\pm 1$
has two subtypes:
![]() $+1$
and
$+1$
and
![]() $-1$
, depending on whether one can get from the original tangle T to tangle
$-1$
, depending on whether one can get from the original tangle T to tangle
![]() $+1$
in an even or odd number of crossing changes between different components. For the statement below, we will need to consider oriented tangles. The orientation will be considered up to the total orientation reversal, hence
$+1$
in an even or odd number of crossing changes between different components. For the statement below, we will need to consider oriented tangles. The orientation will be considered up to the total orientation reversal, hence

Every oriented tangle of type
![]() $\pm 1$
is either oriented vertically or horizontally depending on whether one can reduce it to the vertically or horizontally oriented tangles of Figure 6, by crossing changes which involve an even number of changes between different components.
$\pm 1$
is either oriented vertically or horizontally depending on whether one can reduce it to the vertically or horizontally oriented tangles of Figure 6, by crossing changes which involve an even number of changes between different components.

Figure 6:
![]() $1$
and
$1$
and
![]() $-1$
tangle types oriented vertically (on the left) and horizontally (on the right).
$-1$
tangle types oriented vertically (on the left) and horizontally (on the right).
If we denote the writhe of a framed oriented link L by
![]() $w(L),$
then
$w(L),$
then
![]() $(-A)^{-3w(L)}[L]$
is the Jones polynomial
$(-A)^{-3w(L)}[L]$
is the Jones polynomial
![]() $J(L)$
for
$J(L)$
for
![]() $t=A^{-4}.$
Since
$t=A^{-4}.$
Since
![]() $J(L)\in t^{(|L|-1)/2}\mathbb Z[t^{\pm 1}]$
, where
$J(L)\in t^{(|L|-1)/2}\mathbb Z[t^{\pm 1}]$
, where
![]() $|L|$
denotes the number of components of L, the bracket
$|L|$
denotes the number of components of L, the bracket
![]() $[L]$
can be written with all exponents of A congruent to
$[L]$
can be written with all exponents of A congruent to
We extend this property to Kauffman brackets of tangles.
Let
![]() $|T|$
denote the number of connected components of a tangle
$|T|$
denote the number of connected components of a tangle
![]() $T.$
$T.$
Proposition For any oriented tangle T, the bracket
![]() $[T]_\tau $
, for
$[T]_\tau $
, for
![]() $\tau $
is
$\tau $
is
![]() $0$
or
$0$
or
![]() $\infty $
, can be written with all exponents of A congruent to
$\infty $
, can be written with all exponents of A congruent to
where
![]() $\delta _\tau =0$
or
$\delta _\tau =0$
or
![]() $2$
depending on whether
$2$
depending on whether
![]() $\tau $
is
$\tau $
is
![]() $0$
or
$0$
or
![]() $\infty $
and
$\infty $
and
![]() $\eta (T)=0$
for T of type
$\eta (T)=0$
for T of type
![]() $\pm 1$
vertically oriented (see Figure 6) and for type
$\pm 1$
vertically oriented (see Figure 6) and for type
![]() $0$
. Otherwise,
$0$
. Otherwise,
![]() $\eta (T)=1$
; that is for type
$\eta (T)=1$
; that is for type
![]() $\pm 1$
oriented horizontally and for T of type
$\pm 1$
oriented horizontally and for T of type
![]() $\infty $
.
$\infty $
.
Proof We prove it for all oriented tangle diagrams D by induction on the crossing number
![]() $c(D)$
of D. The statement is obvious for
$c(D)$
of D. The statement is obvious for
![]() $c(D)=0.$
Assume that the statement holds for all diagrams with
$c(D)=0.$
Assume that the statement holds for all diagrams with
![]() $c(D)\leq C$
. We are going to prove the statement for diagrams D with
$c(D)\leq C$
. We are going to prove the statement for diagrams D with
![]() $C+1$
crossings by the induction on the undescendedness
$C+1$
crossings by the induction on the undescendedness
![]() $ud(D)$
, of D, which measures the failure of being a descending diagram.
$ud(D)$
, of D, which measures the failure of being a descending diagram.
It is defined as follows: We will refer to the connected components of the tangle represented by D as the components of D for simplicity. Let us also orient and order all these components and let us choose a base point on each loop component of D away from crossings. Next, let us follow all components of D according to their order, and according to their orientations, starting with their beginning (if they are arcs) or their base points (if they are loops). A crossing of D is improper if its underpass is transversed before its overpass is. (The improperness of a crossing will depend on the above choices.) The undescendedness,
![]() $ud(D)$
, of D is the minimum of the numbers of improper crossings in D over all choices of basepoints and choices of orientations and orderings of the components of the tangle represented by D. In particular,
$ud(D)$
, of D is the minimum of the numbers of improper crossings in D over all choices of basepoints and choices of orientations and orderings of the components of the tangle represented by D. In particular,
If
![]() $ud(D)=0$
then D is isotopic to
$ud(D)=0$
then D is isotopic to
![]() $\langle 0\rangle , \langle \pm 1\rangle $
or
$\langle 0\rangle , \langle \pm 1\rangle $
or
![]() $\langle \infty \rangle $
depending on the type of the tangle represented by D, with possibly some additional unlinked trivial loop components. It is easy to see that the statement follows in this case. Assume that the statement holds for all diagrams D with
$\langle \infty \rangle $
depending on the type of the tangle represented by D, with possibly some additional unlinked trivial loop components. It is easy to see that the statement follows in this case. Assume that the statement holds for all diagrams D with
![]() $c(D)=C+1$
and
$c(D)=C+1$
and
![]() $ud(D)\leq u$
. Let D be a diagram with
$ud(D)\leq u$
. Let D be a diagram with
![]() $c(D)=C+1$
and
$c(D)=C+1$
and
![]() $ud(D)= u+1,$
and let v be one of the improper crossings counted in
$ud(D)= u+1,$
and let v be one of the improper crossings counted in
![]() $ud(D)$
(according to a certain choice of base points, orientations, and of ordering). Assume that this crossing has sign
$ud(D)$
(according to a certain choice of base points, orientations, and of ordering). Assume that this crossing has sign
![]() $\varepsilon =\pm $
. Then
$\varepsilon =\pm $
. Then
where
![]() $D_\varepsilon $
is D, and
$D_\varepsilon $
is D, and
![]() $D_{-\varepsilon }$
is D with the crossing v reversed and
$D_{-\varepsilon }$
is D with the crossing v reversed and
![]() $D_0$
is D with v smoothed out. Consequently,
$D_0$
is D with v smoothed out. Consequently,
Since
and
by the inductive assumption, the exponents of
![]() $A^{-2\varepsilon }[D_{-\varepsilon }]_\tau $
are
$A^{-2\varepsilon }[D_{-\varepsilon }]_\tau $
are
which equals
Similarly, the exponents of
![]() $A^{-\varepsilon }(A^2-A^{-2})[D_0]_\tau $
are
$A^{-\varepsilon }(A^2-A^{-2})[D_0]_\tau $
are
which equals
To complete the argument, let us assume first that v is a single component crossing. Then
![]() $D,D_{-\varepsilon }, D_0$
have the same type and
$D,D_{-\varepsilon }, D_0$
have the same type and
Hence, the quantities in (5.1) and (5.2) coincide and imply that the exponents of
![]() $[D]_\tau $
are
$[D]_\tau $
are
as claimed in the statement, thus completing the proof of the inductive step in the single-component crossing case.
If v is a two-component crossing, then
![]() $D,D_{-\varepsilon }$
have the same type and
$D,D_{-\varepsilon }$
have the same type and
A two-component crossing smoothing transforms tangle types and orientations in one of the following three ways:

(Since these are tangle types, no crossing signs are indicated.)
Consequently,
![]() $\eta (D)\ne \eta (D_0).$
Hence, the quantities in (5.1) and (5.2) coincide and imply that the the exponents of
$\eta (D)\ne \eta (D_0).$
Hence, the quantities in (5.1) and (5.2) coincide and imply that the the exponents of
![]() $[D]_\tau $
are
$[D]_\tau $
are
as claimed in the statement, thus completing the proof of the inductive step in the two-component crossing case.
6 The KB ratio of a tangle
Let
It is well defined, because
![]() $[T]_\infty $
and
$[T]_\infty $
and
![]() $[T]_0$
cannot both vanish. (This follows, for example, from Proposition 5.1 and the fact that
$[T]_0$
cannot both vanish. (This follows, for example, from Proposition 5.1 and the fact that
![]() $[N(T)]\ne 0,$
because
$[N(T)]\ne 0,$
because
![]() $[N(T)]=(-2)^{|N(T)|}$
for
$[N(T)]=(-2)^{|N(T)|}$
for
![]() $A=\pm 1$
.)
$A=\pm 1$
.)
Note that
![]() $Q(T)$
is preserved by the first Reidemeister move and, hence, it is an invariant of unframed tangles.
$Q(T)$
is preserved by the first Reidemeister move and, hence, it is an invariant of unframed tangles.
Let
Proposition 5.2 implies
thus proving Theorem 1.6. We call it the Kauffman bracket ratio, or the KB-ratio of T, for short. KB-ratios will play an important role in the remainder of the paper.
Proposition For any tangle
![]() $T,$
$T,$
(1)
![]() $\{-T\}_q=-q^{-1}\{ T\}_{q^{-1}},$
where the subscript on the right-hand side indicates q substituted by
$\{-T\}_q=-q^{-1}\{ T\}_{q^{-1}},$
where the subscript on the right-hand side indicates q substituted by
![]() $q^{-1}$
. (Recall that
$q^{-1}$
. (Recall that
![]() $-T$
is the mirror image of T.)
$-T$
is the mirror image of T.)
(2)
![]() $\{ R(T)\}_q= -q^{-1}/\{T \}_q$
, where R denotes the rotation operation, as before.
$\{ R(T)\}_q= -q^{-1}/\{T \}_q$
, where R denotes the rotation operation, as before.
Additionally, for any
![]() $T'$
,
$T'$
,
(3)
![]() $\{T+T' \}_q=\{T\}_q+\{T' \}_q+(q-1)\{T\}_q\{T' \}_q.$
$\{T+T' \}_q=\{T\}_q+\{T' \}_q+(q-1)\{T\}_q\{T' \}_q.$
Proof (1)
![]() $\{-T\}_q=A^{-2}Q(-T)=A^{-2}Q(T)_{A^{-1}}=A^{-4}\{T\}_{q^{-1}}.$
$\{-T\}_q=A^{-2}Q(-T)=A^{-2}Q(T)_{A^{-1}}=A^{-4}\{T\}_{q^{-1}}.$
(2) Since
![]() $Q(R(T))=1/Q(T)$
,
$Q(R(T))=1/Q(T)$
,
(3) By the following identity

and, hence,
7 q-Deformed rationals
Quantum integers,
 $$ \begin{align*}[n]_q=\begin{cases} \frac{1-q^n}{1-q}, & \text{for } n\ne 0,\cr 0, & \text{for } n=0,\cr \end{cases}\end{align*} $$
$$ \begin{align*}[n]_q=\begin{cases} \frac{1-q^n}{1-q}, & \text{for } n\ne 0,\cr 0, & \text{for } n=0,\cr \end{cases}\end{align*} $$
for
![]() $n\in \mathbb Z$
, appear already in the 1808 work of Gauss on binomial coefficients [Reference Gauss14, Reference Jackson17]. They are at the foundation of quantum calculus (see [Reference Kac and Cheung20]), and are indispensable in quantum algebra and in quantum topology (see, e.g., [Reference Kassel23]).
$n\in \mathbb Z$
, appear already in the 1808 work of Gauss on binomial coefficients [Reference Gauss14, Reference Jackson17]. They are at the foundation of quantum calculus (see [Reference Kac and Cheung20]), and are indispensable in quantum algebra and in quantum topology (see, e.g., [Reference Kassel23]).
Quantum integers were extended in [Reference Morier-Genoud and Ovsienko33] to “q-deformed rationals”
![]() $[x]_q$
for
$[x]_q$
for
![]() $x\in \mathbb Q.$
They satisfy the following identities:
$x\in \mathbb Q.$
They satisfy the following identities:
(cf. [Reference Leclere and Morier-Genoud28]).
They can be defined also through our bracket
![]() $\{\cdot \}_q$
and, in fact, in this way, they have been discovered independently by us in the first version of this paper on arXiv.
$\{\cdot \}_q$
and, in fact, in this way, they have been discovered independently by us in the first version of this paper on arXiv.
Note that the first two identities of Eq. (7.1) are satisfied by
![]() $\{\cdot \}_q$
by Proposition 6.1. The last one is satisfied as well: According to Conway’s correspondence
$\{\cdot \}_q$
by Proposition 6.1. The last one is satisfied as well: According to Conway’s correspondence
![]() ${\langle 1/x\rangle =R(-\langle x\rangle )}$
and, hence,
${\langle 1/x\rangle =R(-\langle x\rangle )}$
and, hence,
By the theory of continued fractions, the above identities determine the values of
![]() $[x]_q$
and of
$[x]_q$
and of
![]() $\{x\}_q$
for all
$\{x\}_q$
for all
![]() $x\in \mathbb Q.$
(In fact, the formulas for
$x\in \mathbb Q.$
(In fact, the formulas for
![]() $x+1$
and
$x+1$
and
![]() $-1/x$
are sufficient.) This implies the following statement from the Introduction.
$-1/x$
are sufficient.) This implies the following statement from the Introduction.
Theorem 1.7
For every
![]() $x\in \widehat {\mathbb {Q}},$
$x\in \widehat {\mathbb {Q}},$
![]() $[x]_q=\{\langle x\rangle \}_q$
.
$[x]_q=\{\langle x\rangle \}_q$
.
Let
![]() $\{\langle x\rangle \}_1$
denote
$\{\langle x\rangle \}_1$
denote
![]() $\{\langle x\rangle \}_q$
evaluated at
$\{\langle x\rangle \}_q$
evaluated at
![]() $q=1.$
By the above theorem and Eq. (7.1), we have the following.
$q=1.$
By the above theorem and Eq. (7.1), we have the following.
Corollary 7.1 ([Reference Morier-Genoud and Ovsienko33])
For every
![]() $x\in \widehat {\mathbb {Q}}, \{\langle x\rangle \}_1=x$
.
$x\in \widehat {\mathbb {Q}}, \{\langle x\rangle \}_1=x$
.
Corollary 7.2 The Kauffman bracket distinguishes all framed tangles among rational ones.
Proof Any two framed rational tangles
![]() $T,T'$
with
$T,T'$
with
![]() $\{T\}_q=\{T'\}_q$
must represent the same rational number and, hence, may differ by framing only. However, if
$\{T\}_q=\{T'\}_q$
must represent the same rational number and, hence, may differ by framing only. However, if
![]() $T'$
is obtained from T by adding n twists to it (where negative n means
$T'$
is obtained from T by adding n twists to it (where negative n means
![]() $|n|$
negative twists) then
$|n|$
negative twists) then
![]() $[T']= (-A)^{3n}[T].$
Hence,
$[T']= (-A)^{3n}[T].$
Hence,
![]() $n=0.$
$n=0.$
8 The uniqueness of the KB of a solution of a system of tangle equations
Theorem 8.1 For any system of framed tangle equations (1.1),
![]() $[X]$
is unique.
$[X]$
is unique.
Proof Let X be a solution of framed (1.1). Then

and analogously for
![]() $T_2.$
Hence,
$T_2.$
Hence,
 $$ \begin{align*}\left(\begin{matrix} [N(X+T_1)]\\ [N(X+T_2)] \end{matrix}\right)=B\cdot \left(\begin{matrix} [X]_0\\ [X]_\infty \end{matrix}\right),\end{align*} $$
$$ \begin{align*}\left(\begin{matrix} [N(X+T_1)]\\ [N(X+T_2)] \end{matrix}\right)=B\cdot \left(\begin{matrix} [X]_0\\ [X]_\infty \end{matrix}\right),\end{align*} $$
where
 $$ \begin{align*}B=\left(\begin{matrix} [T_1]_0\delta + [T_1]_\infty & [T_1]_0 + [T_1]_\infty\delta\\ [T_2]_0\delta + [T_2]_\infty & [T_2]_0 + [T_2]_\infty\delta\\ \end{matrix}\right).\end{align*} $$
$$ \begin{align*}B=\left(\begin{matrix} [T_1]_0\delta + [T_1]_\infty & [T_1]_0 + [T_1]_\infty\delta\\ [T_2]_0\delta + [T_2]_\infty & [T_2]_0 + [T_2]_\infty\delta\\ \end{matrix}\right).\end{align*} $$
Note that
 $$ \begin{align*}\det B=(\delta^2-1)\cdot \det \left(\begin{matrix} [T_1]_0 & [T_1]_\infty\\ [T_2]_0 & [T_2]_\infty\end{matrix}\right)\end{align*} $$
$$ \begin{align*}\det B=(\delta^2-1)\cdot \det \left(\begin{matrix} [T_1]_0 & [T_1]_\infty\\ [T_2]_0 & [T_2]_\infty\end{matrix}\right)\end{align*} $$
is nonzero, even for
![]() $q=1$
by Corollary 7.1.
$q=1$
by Corollary 7.1.
Given a system of equations (1.1), let
 $$ \begin{align*}q_\mu=\frac{\det \left(\begin{matrix} [L_1] & [T_1]_0+\mu [T_1]_\infty\\ [L_2] & [T_2]_0+\mu [T_2]_\infty\end{matrix}\right)} {(\delta-\mu)\cdot \det \left(\begin{matrix} [T_1]_0 & [T_1]_\infty\\ [T_2]_0 & [T_2]_\infty\end{matrix}\right)}\ \text{for}\ \mu=\pm 1.\end{align*} $$
$$ \begin{align*}q_\mu=\frac{\det \left(\begin{matrix} [L_1] & [T_1]_0+\mu [T_1]_\infty\\ [L_2] & [T_2]_0+\mu [T_2]_\infty\end{matrix}\right)} {(\delta-\mu)\cdot \det \left(\begin{matrix} [T_1]_0 & [T_1]_\infty\\ [T_2]_0 & [T_2]_\infty\end{matrix}\right)}\ \text{for}\ \mu=\pm 1.\end{align*} $$
Then, by Cramer’s rule, one can verify that
 $$ \begin{align*}[X]_0=\det \left(\begin{matrix} [L_1]& [T_1]_0+[T_1]_\infty \delta\\ [L_2]& [T_2]_0+ [T_2]_\infty\delta\end{matrix}\right)/\det B= \frac{1}{2}(q_1-q_{-1})\end{align*} $$
$$ \begin{align*}[X]_0=\det \left(\begin{matrix} [L_1]& [T_1]_0+[T_1]_\infty \delta\\ [L_2]& [T_2]_0+ [T_2]_\infty\delta\end{matrix}\right)/\det B= \frac{1}{2}(q_1-q_{-1})\end{align*} $$
and
 $$ \begin{align*}[X]_\infty=\det \left(\begin{matrix} [T_1]_0\delta+[T_1]_\infty & [L_1]\\ [T_2]_0\delta+ [T_2]_\infty & [L_2]\end{matrix}\right)/\det B= \frac{1}{2}(q_{1}+q_{-1}).\\[-40pt]\end{align*} $$
$$ \begin{align*}[X]_\infty=\det \left(\begin{matrix} [T_1]_0\delta+[T_1]_\infty & [L_1]\\ [T_2]_0\delta+ [T_2]_\infty & [L_2]\end{matrix}\right)/\det B= \frac{1}{2}(q_{1}+q_{-1}).\\[-40pt]\end{align*} $$
The above equations provide necessary algebraic conditions for the existence of a framed solution of (1.1). In particular, we have the following.
Corollary 8.2 A necessary condition for the existence of a framed solution to (1.1) is that
and that
![]() $A^{-2}p_\infty /p_0\in {\mathbb {Q}}(A^4).$
$A^{-2}p_\infty /p_0\in {\mathbb {Q}}(A^4).$
Note that Theorem 8.1 together with Corollary 7.2 implies Proposition 1.1 stating that every framed system (1.1) has at most one-framed rational solution.
9 Jones conjecture for tangles
Recall that
![]() $U^n$
denotes the unknot with framing
$U^n$
denotes the unknot with framing
![]() $n\in {\mathbb {Z}}$
.
$n\in {\mathbb {Z}}$
.
Lemma 9.1 (Kauffman bracket version of Jones conjecture)
The Jones conjecture (JC) is equivalent to its Kauffman bracket version (KBJC): if
![]() $[K]=r\cdot A^k$
, for some
$[K]=r\cdot A^k$
, for some
![]() $r,k\in {\mathbb {Z}}$
, then
$r,k\in {\mathbb {Z}}$
, then
![]() $K=U^n$
for some
$K=U^n$
for some
![]() $n\in {\mathbb {Z}}.$
$n\in {\mathbb {Z}}.$
Proof KBJC
![]() $\Rightarrow $
JC: Suppose that the Jones polynomial of K is
$\Rightarrow $
JC: Suppose that the Jones polynomial of K is
![]() $J(K)=1$
for some knot K. Then K with some framing has its Kauffman bracket equal to
$J(K)=1$
for some knot K. Then K with some framing has its Kauffman bracket equal to
![]() $(-A^3)^n$
for some
$(-A^3)^n$
for some
![]() $n\in {\mathbb {Z}}.$
By KBJC,
$n\in {\mathbb {Z}}.$
By KBJC,
![]() $K=U^n$
. Hence, K is trivial as an unframed knot.
$K=U^n$
. Hence, K is trivial as an unframed knot.
JC
![]() $\Rightarrow $
KBJC: Suppose that
$\Rightarrow $
KBJC: Suppose that
![]() $[K]=r\cdot A^k$
, for some
$[K]=r\cdot A^k$
, for some
![]() $r,k\in {\mathbb {Z}}$
. Then
$r,k\in {\mathbb {Z}}$
. Then
![]() $J(K)=r (-A)^{-3w(K)}A^k.$
By [Reference Ganzell13, Corollary 3],
$J(K)=r (-A)^{-3w(K)}A^k.$
By [Reference Ganzell13, Corollary 3],
![]() $J(K)=1$
. Hence, K is (unframed) trivial, by the Jones conjecture.
$J(K)=1$
. Hence, K is (unframed) trivial, by the Jones conjecture.
Now, we can formulate three versions of the Jones conjecture for tangles.
Theorem 9.2 The Jones conjecture is equivalent to each of the following statements:
(a) For any framed tangle T, if
 $[T]=\begin {pmatrix} 1\\ 0\end {pmatrix}$
then
$[T]=\begin {pmatrix} 1\\ 0\end {pmatrix}$
then
![]() $T=\langle 0\rangle ^0.$
$T=\langle 0\rangle ^0.$
(b) If
 $[T]=\begin {pmatrix} r\cdot A^{n}\\ 0\end {pmatrix}$
for some
$[T]=\begin {pmatrix} r\cdot A^{n}\\ 0\end {pmatrix}$
for some
![]() $r,n\in {\mathbb {Z,}}$
then
$r,n\in {\mathbb {Z,}}$
then
![]() $T=\langle 0\rangle $
as an unframed tangle.
$T=\langle 0\rangle $
as an unframed tangle.
(c) If
![]() $[T']=[T]$
and T is rational, then
$[T']=[T]$
and T is rational, then
![]() $T'=T$
as framed tangles.
$T'=T$
as framed tangles.
It is worth noting that statement (c) above does not hold for rational knots. That is, there are examples of distinct rational knots with coinciding Jones polynomials [Reference Kanenobu22].
Proof of Theorem 9.2
JC implies (a): Assume the Jones conjecture holds and that
![]() $[T]=(1,0).$
(For convenience, in this proof we will write all vertical vectors horizontally.) Then
$[T]=(1,0).$
(For convenience, in this proof we will write all vertical vectors horizontally.) Then
![]() $[R(T)]=(0,1)$
and it is easy to check that
$[R(T)]=(0,1)$
and it is easy to check that
By Lemma 9.1,
Now, by Lemma 4.1,
![]() $R(T)=\langle \infty \rangle $
and, hence,
$R(T)=\langle \infty \rangle $
and, hence,
![]() $T=\langle 0\rangle .$
$T=\langle 0\rangle .$
(a) implies (c): Suppose that T is rational and
![]() $[T']=[T]$
. Then T can be transformed
$[T']=[T]$
. Then T can be transformed
by the operations of rotation,
![]() $R(\cdot ),$
of addition of one,
$R(\cdot ),$
of addition of one,
![]() $P(T)= T +\langle 1\rangle ,$
its inverse,
$P(T)= T +\langle 1\rangle ,$
its inverse,
![]() $P^{-1}(T).$
and adding a positive or negative kink,
$P^{-1}(T).$
and adding a positive or negative kink,
![]() $F^{\pm 1}(T)$
, (for framing changes). Let us apply the same operations to
$F^{\pm 1}(T)$
, (for framing changes). Let us apply the same operations to
![]() $T':$
$T':$
Since

the operations R and P induce linear transformations of the Kauffman brackets:
 $$ \begin{align*}[R(T)] = \left(\begin{matrix} 0 &1\\ 1 & 0\end{matrix}\right)[T],\quad [P(T)] = [T+\langle 1\rangle]=\left(\begin{matrix} A^{-1} & 0\\ A & -A^3\end{matrix}\right)\cdot [T].\end{align*} $$
$$ \begin{align*}[R(T)] = \left(\begin{matrix} 0 &1\\ 1 & 0\end{matrix}\right)[T],\quad [P(T)] = [T+\langle 1\rangle]=\left(\begin{matrix} A^{-1} & 0\\ A & -A^3\end{matrix}\right)\cdot [T].\end{align*} $$
Since
![]() $R,P$
and F are invertible,
$R,P$
and F are invertible,
![]() $[T_i]=[T_i']$
for every i and, hence,
$[T_i]=[T_i']$
for every i and, hence,
![]() $[T"]=(1,0).$
By (a),
$[T"]=(1,0).$
By (a),
![]() $T_k'=\langle 0\rangle $
, implying that
$T_k'=\langle 0\rangle $
, implying that
![]() $T'=T.$
$T'=T.$
(c) implies (b): Assume that
![]() $[T]=(r\cdot A^n,0)$
, for some
$[T]=(r\cdot A^n,0)$
, for some
![]() $r,n\in {\mathbb {Z}}.$
Then
$r,n\in {\mathbb {Z}}.$
Then
![]() $[D(T)]=r\cdot A^n$
and by [Reference Ganzell13, Corollary 3] (as in the proof of Lemma 9.1),
$[D(T)]=r\cdot A^n$
and by [Reference Ganzell13, Corollary 3] (as in the proof of Lemma 9.1),
![]() $J(D(T))=1$
. That implies that
$J(D(T))=1$
. That implies that
![]() $[T]=((-A^3)^k,0)$
has the bracket of
$[T]=((-A^3)^k,0)$
has the bracket of
![]() $\langle 0\rangle $
with some framing
$\langle 0\rangle $
with some framing
![]() $k\in {\mathbb {Z}}.$
Hence, by (c),
$k\in {\mathbb {Z}}.$
Hence, by (c),
![]() $T=\langle 0\rangle $
, as unframed tangle.
$T=\langle 0\rangle $
, as unframed tangle.
(b) implies (a): Suppose
![]() $[T]=(1,0).$
Then by (b),
$[T]=(1,0).$
Then by (b),
![]() $T=\langle 0\rangle $
as an unframed tangle. Since
$T=\langle 0\rangle $
as an unframed tangle. Since
![]() $[T]=(1,0)$
, the framing of T must be trivial.
$[T]=(1,0)$
, the framing of T must be trivial.
(a) implies JC: Let K be a knot with trivial Jones polynomial. Let us frame it so that
![]() $[K]=1.$
Let
$[K]=1.$
Let
![]() $K\# \langle 0\rangle $
be the connected sum of K with the lower strand of
$K\# \langle 0\rangle $
be the connected sum of K with the lower strand of
![]() $\langle 0\rangle .$
Then
$\langle 0\rangle .$
Then
![]() $[K\# \langle 0\rangle ]=(1,0)$
and
$[K\# \langle 0\rangle ]=(1,0)$
and
![]() $K\#\langle 0\rangle =\langle 0\rangle $
as unframed tangles, by (b) (which we proved is implied by (a)), implying that K is trivial.
$K\#\langle 0\rangle =\langle 0\rangle $
as unframed tangles, by (b) (which we proved is implied by (a)), implying that K is trivial.
Theorem 9.2 can be further refined to consider its statements up to a certain numbers of crossings. The crossing number,
![]() $c(T)$
of a framed or unframed tangle T is the minimal number of crossings in its unframed isotopy class. Then we can strengthen the implication JC
$c(T)$
of a framed or unframed tangle T is the minimal number of crossings in its unframed isotopy class. Then we can strengthen the implication JC
![]() $\Rightarrow $
(c) above as follows.
$\Rightarrow $
(c) above as follows.
Proposition Assume that the Jones conjecture holds for knots up to N crossings. If
![]() $[T']=[T],$
and
$[T']=[T],$
and
![]() $c(T)+c(T')<N$
and T is rational then
$c(T)+c(T')<N$
and T is rational then
![]() $T'=T$
(as framed tangles).
$T'=T$
(as framed tangles).
Proof follows the above proof for
![]() $JC \Rightarrow (a) \Rightarrow (c).$
Note that the tangle
$JC \Rightarrow (a) \Rightarrow (c).$
Note that the tangle
![]() $T"$
in Eq. (9.1) has at most
$T"$
in Eq. (9.1) has at most
![]() $c(T)+c(T')$
crossings. Now, observe that the Jones conjecture up to N crossings implies statement (a) up to
$c(T)+c(T')$
crossings. Now, observe that the Jones conjecture up to N crossings implies statement (a) up to
![]() $N-1$
crossings.
$N-1$
crossings.
10 Slopes of tangles
Recall that we call
![]() $\{T\}_1\in \widehat {\mathbb {Q}}$
the algebraic slope of T and that we postulate in Conjecture 1.8 that if an m-essential surface in the complement of T has a slope, that slope is
$\{T\}_1\in \widehat {\mathbb {Q}}$
the algebraic slope of T and that we postulate in Conjecture 1.8 that if an m-essential surface in the complement of T has a slope, that slope is
![]() $\{T\}_1.$
In this section, we define the slope of a surface and we prove our conjecture for algebraic tangles.
$\{T\}_1.$
In this section, we define the slope of a surface and we prove our conjecture for algebraic tangles.
Let us denote by
![]() $\cal T$
the double cover of the boundary
$\cal T$
the double cover of the boundary
![]() $S^2$
of the three-ball containing T, branched around
$S^2$
of the three-ball containing T, branched around
![]() $T\cap S^2$
, and let
$T\cap S^2$
, and let
![]() $\theta : \cal T\to \cal T$
be the Deck transformation of that covering. The arc
$\theta : \cal T\to \cal T$
be the Deck transformation of that covering. The arc
![]() $\mu $
of the denominator closure arc connecting SW and NW and its image
$\mu $
of the denominator closure arc connecting SW and NW and its image
![]() $\theta (\mu )$
under
$\theta (\mu )$
under
![]() $\theta $
form a loop which we call the meridian of
$\theta $
form a loop which we call the meridian of
![]() $\cal T$
, cf. Figure 7. (By considering the numerator arc SE–NE, we get a parallel loop in
$\cal T$
, cf. Figure 7. (By considering the numerator arc SE–NE, we get a parallel loop in
![]() $\cal T.$
) Similarly, the arc SW–SE of the numerator closure together with its
$\cal T.$
) Similarly, the arc SW–SE of the numerator closure together with its
![]() $\theta $
-image forms a loop which we call the longitude. We orient the meridian and longitude as in Figure 7.
$\theta $
-image forms a loop which we call the longitude. We orient the meridian and longitude as in Figure 7.

Figure 7: The double cover of a sphere
![]() $\partial B^3$
branched along the endpoints of a tangle in
$\partial B^3$
branched along the endpoints of a tangle in
![]() $B^3$
.
$B^3$
.
Recall that a loop
![]() $\gamma $
in
$\gamma $
in
![]() $S^2_4$
is dividing if it separates the punctures of
$S^2_4$
is dividing if it separates the punctures of
![]() $S^2_4$
into two groups of two. Its slope
$S^2_4$
into two groups of two. Its slope
![]() $s(\gamma )$
is the slope of its lift to
$s(\gamma )$
is the slope of its lift to
![]() $\cal T$
in the longitude–meridian basis of
$\cal T$
in the longitude–meridian basis of
![]() $\cal T,$
so that the slopes of the longitude and the meridian in
$\cal T,$
so that the slopes of the longitude and the meridian in
![]() $\cal T$
are of the slope
$\cal T$
are of the slope
![]() $0$
and
$0$
and
![]() $\infty $
, respectively. It is well defined by [Reference Farb and Margalit12, Proposition 2.6].
$\infty $
, respectively. It is well defined by [Reference Farb and Margalit12, Proposition 2.6].
As before, consider an m-essential surface S properly embedded in
![]() $D^3 \smallsetminus T$
. Recall that if its boundary contains a dividing loop
$D^3 \smallsetminus T$
. Recall that if its boundary contains a dividing loop
![]() $\gamma $
we say that S is dividing and has slope
$\gamma $
we say that S is dividing and has slope
![]() $s(\gamma )$
. (Then it is easy to see that all other dividing loops of
$s(\gamma )$
. (Then it is easy to see that all other dividing loops of
![]() $\partial S$
must be parallel.) This definition is inspired by that in [Reference Ozawa35], where the slopes are defined for algebraic tangles.
$\partial S$
must be parallel.) This definition is inspired by that in [Reference Ozawa35], where the slopes are defined for algebraic tangles.
For example,
![]() $T=\langle 0\rangle $
has a horizontal disk
$T=\langle 0\rangle $
has a horizontal disk
![]() $S=D^2$
in its complement which is m-essential. Since
$S=D^2$
in its complement which is m-essential. Since
![]() $\partial D^2$
lifts to the longitude in
$\partial D^2$
lifts to the longitude in
![]() $\cal T$
, the slope of
$\cal T$
, the slope of
![]() $\langle 0\rangle $
is
$\langle 0\rangle $
is
![]() $0$
. One can construct any rational tangle from
$0$
. One can construct any rational tangle from
![]() $\langle 0\rangle $
by the operations of rotation and addition of
$\langle 0\rangle $
by the operations of rotation and addition of
![]() $\pm 1$
which modify S accordingly. Consequently, it is easy to see that the slope of any rational tangle
$\pm 1$
which modify S accordingly. Consequently, it is easy to see that the slope of any rational tangle
![]() $\langle x\rangle $
is
$\langle x\rangle $
is
![]() $x.$
$x.$
Ozawa proves that for every algebraic tangle T, every m-essential surface in
![]() $B^3-T$
is dividing and its slope depends on T only (see [Reference Ozawa35]). This is the slope of T,
$B^3-T$
is dividing and its slope depends on T only (see [Reference Ozawa35]). This is the slope of T,
![]() $s(T)\in \widehat {\mathbb {Q}}.$
$s(T)\in \widehat {\mathbb {Q}}.$
Recall Theorem 1.9.
Theorem 1.9
For every algebraic tangle T,
![]() $\{T\}_1=s(T).$
$\{T\}_1=s(T).$
Proof By [Reference Ozawa35, Proposition 2.4],
for algebraic tangles. Furthermore,
![]() $s(\langle n\rangle )= n$
, for
$s(\langle n\rangle )= n$
, for
![]() $n\in {\mathbb {Z}}$
. Since
$n\in {\mathbb {Z}}$
. Since
![]() $\{\cdot \}_1$
satisfies the same properties by Proposition 6.1 and since these properties determine the values of
$\{\cdot \}_1$
satisfies the same properties by Proposition 6.1 and since these properties determine the values of
![]() $s(T)$
and of
$s(T)$
and of
![]() $\{T\}_1$
for algebraic tangles, the statement follows.
$\{T\}_1$
for algebraic tangles, the statement follows.
The following provides an interpretation of the algebraic slope in terms of algebraic topology.
Proposition
![]() $|\{T\}_1|= \det (N(T))/\det (D(T))$
where
$|\{T\}_1|= \det (N(T))/\det (D(T))$
where
![]() $\det $
denotes the link determinant.
$\det $
denotes the link determinant.
Proof The determinant of
![]() $N(T)$
is
$N(T)$
is
where
![]() $A=e^{\pi i/4}$
,
$A=e^{\pi i/4}$
,
![]() $w(N(T))$
is the writhe of
$w(N(T))$
is the writhe of
![]() $N(T)$
(with some orientation) and
$N(T)$
(with some orientation) and

Taking an analogous formula for
![]() $[D(T)],$
we have
$[D(T)],$
we have
Acknowledgment
The author thanks V. Ovsienko and M. Ozawa for helpful discussions.























