Article contents
Summability Methods on Matrix Spaces
Published online by Cambridge University Press: 20 November 2018
Extract
The matrix spaces under consideration are the four main types of irreducible bounded symmetric domains given by Cartan (5). Let z = (zjk) be a matrix of complex numbers, z' its transpose, z* its conjugate transpose and I = I(n) the identity matrix of order n. Then the first three types are defined by
(1)
where z is an n by m matrix (n ≤ m), a symmetric or a skew-symmetric matrix of order n (16). The fourth type is the set of complex spheres satisfying
(2)
where z is an n by 1 matrix. It is known that each of these domains possesses a distinguished boundary B which in the first three cases is given by
(3)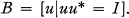
(In the case of skew symmetric matrices the distinguished boundary is given by (2) only if n is even.)
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 1961
References
- 1
- Cited by


