Hostname: page-component-586b7cd67f-g8jcs Total loading time: 0 Render date: 2024-11-27T19:59:58.516Z Has data issue: false hasContentIssue false
  • English
  • Français

Sum of Hermitian Matrices with Given Eigenvalues: Inertia, Rank, and Multiple Eigenvalues

Part of: Basic linear algebra

Published online by Cambridge University Press:  20 November 2018

Chi-Kwong Li  and
Yiu-Tung Poon
Chi-Kwong Li
Affiliation:
Department of Mathematics, College of William and Mary, Williamsburg, VA 23185, USA and University of Hong Kong, e-mail: [email protected]
Yiu-Tung Poon
Affiliation:
Department of Mathematics, Iowa State University, Ames, IA 50011, USA, e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

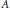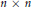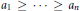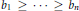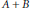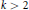Let $A$ and $B$ be $n\,\times \,n$ complex Hermitian (or real symmetric) matrices with eigenvalues ${{a}_{1}}\,\ge \,\cdots \,\ge \,{{a}_{n}}$ and ${{b}_{1}}\,\ge \,\cdots \,\ge \,{{b}_{n}}$. All possible inertia values, ranks, and multiple eigenvalues of $A\,+\,B$ are determined. Extension of the results to the sum of $k$ matrices with $k\,>\,2$ and connections of the results to other subjects such as algebraic combinatorics are also discussed.

Keywords

complex Hermitian matricesreal symmetric matricesinertiarankmultiple eigenvalues

MSC classification

Secondary: 15A42: Inequalities involving eigenvalues and eigenvectors
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 62 , Issue 1 , 01 February 2010 , pp. 109 - 132
Copyright
Copyright © Canadian Mathematical Society 2010

References

[1] H., Bercovici, Li, W. S. and D., Timotin, The Horn conjecture for sums of compact selfadjoint operators. http://arXiv.org/abs/0709.1088.Google Scholar
[2] A. S., Buch, Eigenvalues of Hermitian matrices with positive sum of bounded rank. Linear Algebra Appl. 418(2006), no. 2-3, 480-488. doi:10.1016/j.laa.2006.02.024Google Scholar
[3] M. D., Choi and P. Y., Wu, Convex combinations of projections. Linear Algebra Appl. 136(1990), 25-42. doi:10.1016/0024-3795(90)90019-9Google Scholar
[4] M. D., Choi and P. Y., Wu, Finite-rank perturbations of positive operators and isometries. Studia Math. 173(2006), no. 1, 73-79. doi:10.4064/sm173-1-5Google Scholar
[5] J., Day, W., So, and R. C., Thompson, The spectrum of a Hermitian matrix sum. Linear Algebra Appl. 280(1998), no. 2-3, 289-332. doi:10.1016/S0024-3795(98)10019-8Google Scholar
[6] K., Fan and G., Pall, Imbedding conditions for Hermitian and normal matrices. Canad. J. Math. 9(1957), 298-304.Google Scholar
[7] W., Fulton, Eigenvalues, invariant factors, highest weights, and Schubert calculus. Bull. Amer.Math. Soc. 37(2000), no. 3, 209-249. doi:10.1090/S0273-0979-00-00865-XGoogle Scholar
[8] A., Horn, Eigenvalues of sums of Hermitian matrices. Pacific J. Math 12(1962), 225-241.Google Scholar
[9] A. A., Klyachko, Stable bundles, representation theory and Hermitian operators. Selecta Math. 4(1998), no. 2, 419-445. doi:10.1007/s000290050037Google Scholar
[10] A., Knutson and T., Tao, The honeycomb model of GLn(c) tensor products. I. Proof of the saturation conjecture. J. Amer. Math. Soc. 12(1999), no. 4, 1055-1090. doi:10.1090/S0894-0347-99-00299-4Google Scholar
[11] R. C., Thompson and L. J., Freede, On the eigenvalues of sums of Hermitian matrices. Linear Algebra and Appl. 4(1971), 369-376. doi:10.1016/0024-3795(71)90007-3Google Scholar
[12] R. C., Thompson and L. J., Freede, On the eigenvalues of sums of Hermitian matrices. II. Aequationes Math 5(1970), 103-115. doi:10.1007/BF01819276Google Scholar
[13] Weyl, H., Das asymtotische Verteilungsgesetz der Eigenwerte lineare partieller Differentialgleichungen. Math. Ann. 71(1912), no. 4, 441-479 doi:10.1007/BF01456804Google Scholar