Hostname: page-component-cd9895bd7-gvvz8 Total loading time: 0 Render date: 2024-12-25T07:06:09.708Z Has data issue: false hasContentIssue false
  • English
  • Français

Some Obstacles to Duality in Topological Algebra

Published online by Cambridge University Press:  20 November 2018

Paul Bankston
Paul Bankston*
Affiliation:
Marquette University, Milwaukee, Wisconsin
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

0. Introduction. Functors 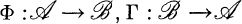form an equivalence of categories (see [8,]) if Γ(Φ(A)) ≅ A and Φ (Γ(B)) ≅ B naturally for all objects A from and B from . Letting 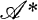denote the opposite of we say that and are dual if there is an equivalence between 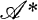and .

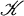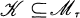Let τ be a similarity type of finitary operation symbols. We let Lτ denote the first order language (with equality) using nonlogical symbols from τ, and consider the class of all algebras of type τ as a category by declaring the morphisms to be all homomorphisms in the usual sense (i.e., those functions preserving the atomic sentences of Lτ). If is a class in (i.e., and 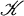is closed under isomorphism), we view 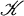as a full subcategory of , and we define the order of 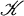to be the number of symbols occurring in τ.

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 34 , Issue 1 , 01 February 1982 , pp. 80 - 90
Copyright
Copyright © Canadian Mathematical Society 1982

References

1. Andréka, H., Makai, E. Jr., Mârki, L. and Németi, T., Reduced products in categories, Contributions to General Algebra (Proc. Klangenfurt Conf., 1978), 25–45.Google Scholar
2. Banaschewski, B. and Bruns, G., Categorical characterization of the MacNeille completion, Archiv der Math. 18 (1967), 369–377.Google Scholar
3. Bankston, P., Obstacles to duality between classes of relational structures (to appear).Google Scholar
4. Bankston, P., Reduced coproducts in the category of compact Hausdorff spaces, (to appear).Google Scholar
5. Chang, C. C. and Keisler, H. J., Model theory (North-Holland, Amsterdam, 1973).Google Scholar
6. Day, A. and Higgs, D., A finiteness condition in categories with ultrapowers, Math. Report 9-73, Lakehead University, (1973).Google Scholar
7. Gillman, L. and Jerison, M., Rings of continuous functions (Van Nostrand, Princeton, 1960).Google Scholar
8. Herrlich, H. and Strecker, G., Category theory (Allyn & Bacon, Boston, 1973).Google Scholar
9. Hewitt, E. and Ross, K. A., Abstract harmonic analysis I (Springer-Verlag, Berlin, 1963).Google Scholar
10. Hofmann, K. H., Mislove, M. and Stralka, A., The Pontryagin duality of compact 0-dimensional semilattices and its applications (Springer-Verlag, Berlin, 1974).Google Scholar
11. Kreisel, G. and Krivine, J. L., Elements of mathematical logic (North Holland, Amsterdam, 1967).Google Scholar
12. Okhuma, T., Ultrapowers in categories, Yokahama Math. J. 14 (1966), 17–37.Google Scholar
13. Pierce, R. S., A note on complete Boolean algebras, Proc. A.M.S. 9 (1958), 892–896.Google Scholar
14. Walker, R. C., The Stone-Cech compactification (Springer-Verlag, Berlin, 1974).Google Scholar