Hostname: page-component-cd9895bd7-jkksz Total loading time: 0 Render date: 2024-12-26T15:57:13.988Z Has data issue: false hasContentIssue false
  • English
  • Français

Simplicial Complexes and Open Subsets of Non-Separable LF-Spaces

Published online by Cambridge University Press:  20 November 2018

Kotaro Mine  and
Katsuro Sakai
Kotaro Mine
Affiliation:
Institute of Mathematics, University of Tsukuba, Tsukuba, 305-8571, Japan email: [email protected]@sakura.cc.tsukuba.ac.jp
Katsuro Sakai
Affiliation:
Institute of Mathematics, University of Tsukuba, Tsukuba, 305-8571, Japan email: [email protected]@sakura.cc.tsukuba.ac.jp
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let 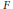$F$ be a non-separable 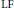$\text{LF}$-space homeomorphic to the direct sum 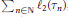${{\sum }_{n\in \text{N}}}\,{{\ell }_{2}}\left( {{\tau }_{n}} \right)$, where 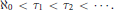${{\aleph }_{0}}<{{\tau }_{1}}<{{\tau }_{2}}<\cdot \cdot \cdot $. It is proved that every open subset 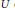$U$ of 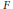$F$ is homeomorphic to the product $\left| K \right|\,\times \,F$ for some locally finite-dimensional simplicial complex 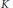$K$ such that every vertex 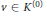$v\,\in \,{{K}^{\left( 0 \right)}}$ has the star 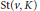$\text{St}\left( v,\,K \right)$ with card $\text{St}{{\left( v,K \right)}^{\left( 0 \right)}}<\tau =\sup {{\tau }_{n}}$ (and card 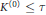${{K}^{\left( 0 \right)}}\le \tau $), and, conversely, if 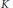$K$ is such a simplicial complex, then the product $\left| K \right|\,\times \,F$ can be embedded in 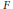$F$ as an open set, where 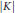$\left| K \right|$ is the polyhedron of 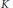$K$ with the metric topology.

Keywords

57N2046A1346T0557N1757Q0557Q40LF-spaceopen setsimplicial complexmetric topologylocally finite-dimensionalstarsmall box productANR, ℓ2(𝒯)ℓ2(𝒯)-manifoldopen embedding Σi∊ℕ𝓵2(τi).
Type
Research Article
Information
Canadian Journal of Mathematics , Volume 63 , Issue 2 , 01 April 2011 , pp. 436 - 459
Copyright
Copyright © Canadian Mathematical Society 2012

References

[1] Anderson, R. D. and McCharen, J. D., On extending homeomorphisms to Fréchet manifolds. Proc. Amer. Math. Soc. 25(1970), 283–289.Google Scholar
[2] Glaser, L. C., Geometrical combinatorial topology. I. Van Nostrand Reinhold Mathematical Studies, 27, Van Nostrand Reinhold Co., London, 1970.Google Scholar
[3] Heisey, R.E., Stability, classification, open embeddings, and triangulation of R1-manifolds. In: Proceeding of the International Conference on Geometric Topology, Polish Scientific Publishers, Warsaw, 1980, pp. 193–196.Google Scholar
[4] Heisey, R.E., Manifolds modelled on the direct limit of lines. Pacific J. Math. 102(1982), no. 1, 47–54.Google Scholar
[5] Henderson, D.W., Infinite-dimensional manifolds are open subsets of Hilbert space. Topology 9(1970), 25–33. doi:10.1016/0040-9383(70)90046-7Google Scholar
[6] Henderson, D.W., Corrections and extensions of two papers about infinite-dimensional manifolds. General Topology and Appl. 1(1971), 321–327. doi:10.1016/0016-660X(71)90004-3Google Scholar
[7] Henderson, D.W., Z-sets in ANR’s. Trans. Amer. Math. Soc. 213(1975), 205–216.Google Scholar
[8] Henderson, D.W. and Schori, R. M., Topological classification of infinite dimensional manifolds by homotopy type. Bull. Amer. Math. Soc. 76(1970), 121–124. doi:10.1090/S0002-9904-1970-12392-8Google Scholar
[9] Hu, S.-T., Theory of retracts. Wayne State University Press, Detroit, MI, 1965.Google Scholar
[10] Mankiewicz, P., On topological, Lipschitz, and uniform classification of LF-spaces. Studia Math. 52(1974), 109–142.Google Scholar
[11] Mine, K. and Sakai, K., Open subsets of LF-spaces. Bull. Pol. Acad. Sci. Math. 56(2008), no. 1, 25–37.Google Scholar
[12] Mine, K. and Sakai, K., Subdivision of simplicial complexes preserving the metric topology. Canad. Math. Bull., to appear.Google Scholar
[13] Narici, L. and Beckenstein, E., Topological vector spaces. Monographs and Textbooks in Pure and Applied Mathematics, 95, Marcel Dekker, Inc., New York, 1985. doi:10.4064/ba56-1-4Google Scholar
[14] Sakai, K., Embeddings of infinite-dimensional pairs and remarks on stability and deficiency. J. Math. Soc. 29(1977), no. 2, 261–280. doi:10.2969/jmsj/02920261Google Scholar
[15] Sakai, K., An embedding theorem of infinite-dimensional manifold pairs in the model space. Fund. Math. 100(1978), 83–87.Google Scholar
[16] Sakai, K., Boundaries and complements of infinite-dimensional manifolds in the model space. Topology Appl. 15(1983), no. 1, 79–91. doi:10.1016/0166-8641(83)90050-0Google Scholar
[17] Sakai, K., On R1-manifolds and Q1-manifolds. Topology Appl. 18(1984), no. 1, 69–79. doi:10.1016/0166-8641(84)90032-4Google Scholar
[18] Toruńczyk, H., Absolute retracts as factors of normed linear spaces. Fund. Math. 86(1974), 53–67.Google Scholar
[19] Toruńczyk, H., Characterizing Hilbert space topology. Fund. Math. 111(1981), no. 3, 247–262.Google Scholar
[20] Toruńczyk, H., A correction of two papers concerning Hilbert manifolds: “Concerning locally homotopy negligible sets and characterization of l2-manifolds” [Fund. Math. 101(1978), no. 2, 93–110] and “Characterizing Hilbert space topology” [ibid. 111(1981), no. 3, 247–262]. Fund. Math. 125(1985), no. 1, 89–93.Google Scholar
[21] Wilansky, A., Modern methods in topological vector spaces. McGraw-Hill, New York, 1978.Google Scholar