Article contents
Simple Algebras that Generalize the Jordan Algebra M38
Published online by Cambridge University Press: 20 November 2018
Extract
In this paper we discuss a generalization of the split exceptional Jordan algebra M38(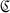 ) of the 3 X 3 hermitian matrices with elements in the split Cayley-Dickson algebra
) of the 3 X 3 hermitian matrices with elements in the split Cayley-Dickson algebra 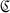 (1). The generalization consists of replacing
(1). The generalization consists of replacing 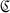 by the non-commutative Jordan algebra
by the non-commutative Jordan algebra 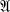 ≡
≡ 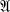 (A, ƒ, s, t) discussed in (2; 3) and forming the set of 3 X 3 hermitian matrices M3m(
(A, ƒ, s, t) discussed in (2; 3) and forming the set of 3 X 3 hermitian matrices M3m(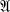 ) ≡ M with elements in the m-dimensional algebra
) ≡ M with elements in the m-dimensional algebra 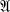 . With the usual definition of multiplication X · Y = ½(XY + YX), M becomes a commutative algebra and we have the following theorem, which shows how the structure of M is reflected by that of
. With the usual definition of multiplication X · Y = ½(XY + YX), M becomes a commutative algebra and we have the following theorem, which shows how the structure of M is reflected by that of 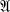 .
.
- Type
- Research Article
- Information
- Copyright
- Copyright © Canadian Mathematical Society 1966
References
- 1
- Cited by


