Hostname: page-component-cd9895bd7-7cvxr Total loading time: 0 Render date: 2024-12-26T16:26:12.343Z Has data issue: false hasContentIssue false
  • English
  • Français

A Semigroup Approach to Linear Algebraic Groups III. Buildings

Published online by Cambridge University Press:  20 November 2018

Mohan S. Putcha
Mohan S. Putcha*
Affiliation:
North Carolina State University, Raleigh, North Carolina
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Introduction. Let K be an algebraically closed field, G = SL(3, K) the group of 3 × 3 matrices over K of determinant 1. Let 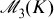denote the monoid of all 3 × 3 matrices over K. If e is an idempotent in 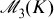, then

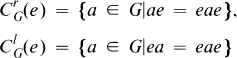
are opposite parabolic subgroups of G in the usual sense [1], [28]. However the map

does not exhaust all pairs of opposite parabolic subgroups of G. Now consider the representation ϕ:G → SL(6, K) given by

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 38 , Issue 3 , 01 June 1986 , pp. 751 - 768
Copyright
Copyright © Canadian Mathematical Society 1986

References

1. Borel, A. and Tits, J., Groupes reductifs, Publ. Math. I.H.E.S. 27 (1965), 55–150.Google Scholar
2. Clifford, A. H. and Preston, G. B., The algebraic theory of semigroups, vol. 1, Math. Survey, No. 7 (Amer. Math. Soc, Providence, R.I., 1962).Google Scholar
3. Humphreys, J. E., Linear algebraic groups (Springer-Verlag, 1981).Google Scholar
4. McAlister, D. B., Rees matrix covers for locally inverse semigroups, Trans. Amer. Math. Soc. 277 (1983), 727–738.Google Scholar
5. Meakin, J., The free local semilattice on a set, Journal of Pure and Applied Algebra 27 (1983), 263–275.Google Scholar
6. Meakin, J. and Pastijn, F., The structure of pseudo-semilattices, Algebra Universalis 13 (1981), 335–373.Google Scholar
7. Nambooripad, K. S. S., Structure of regular semigroups I, Mem. Amer. Math. Soc. 224 (1979).Google Scholar
8. Nambooripad, K. S. S., Pseudo-semilattices and biordered sets I, Simon Stevin 55 (1981), 103–110.Google Scholar
9. Nambooripad, K. S. S., Pseudo-semilattices and biordered sets II, Simon Stevin 56 (1982), 143–160.Google Scholar
10. Nambooripad, K. S. S., Pseudo-semilattices and biordered sets III, Simon Stevin 56 (1982), 239–256.Google Scholar
11. Pastijn, F., The structure of pseudo-inverse semigroups, Trans. Amer. Math. Soc. 273 (1982), 631–655.Google Scholar
12. Putcha, M. S., Green's relations on a connected algebraic monoid, Linear and Multilinear Algebra 12 (1982), 205–214.Google Scholar
13. Putcha, M. S., Connected algebraic monoids, Trans. Amer. Math. Soc. 272 (1982), 693–709.Google Scholar
14. Putcha, M. S., The -class structure of connected algebraic monoids, Journal of Algebra 73 (1981), 601–612.CrossRefGoogle Scholar
15. Putcha, M. S., A semigroup approach to linear algebraic groups, Journal of Algebra 80 (1983), 164–185.Google Scholar
16. Putcha, M. S., Reductive groups and regular semigroups, Semigroup Forum 30 (1984), 253–261.Google Scholar
17. Putcha, M. S., Idempotent cross-sections of -classes, Semigroup Forum 26 (1983), 103–109.CrossRefGoogle Scholar
18. Putcha, M. S., Determinant functions on algebraic monoids, Communications in Algebra 11 (1983), 695–710.Google Scholar
19. Putcha, M. S., A semigroup approach to linear algebraic groups II. Roots, Journal of Pure and Applied Algebra 39 (1986), 153–163.Google Scholar
20. Putcha, M. S., Regular linear algebraic monoids, Trans. Amer. Math. Soc. 290 (1985), 615–626.Google Scholar
21. Renner, L., Reductive monoids are von-Neumann regular, Journal of Algebra 93 (1985), 237–245.Google Scholar
22. Renner, L., Classification of semisimple rank one monoids, Trans. Amer. Math. Soc. 287 (1985), 457–473.Google Scholar
23. Renner, L., Classification of semisimple algebraic monoids, Trans. Amer. Math. Soc. 292 (1985), 193–224.Google Scholar
24. Renner, L., Analogue of the Bruhat decomposition for algebraic monoids, to appear.Google Scholar
25. Renner, L., Private communication.Google Scholar
26. Schein, B. M., Pseudo-semilattices and pseudo-lattices, Izv. Vyss. Ucebn, Zaved. Mat. 2 (117) (1972), 81–94; English transi, in Amer. Math. Soc. Transi. (2) 119, (1983).Google Scholar
27. Suzuki, M., Group theory I (Springer-Verlag, 1982).CrossRefGoogle Scholar
28. Tits, J., Buildings of spherical type and finite B-N pairs, Lecture Notes in Math. 386 (Berlin, Springer-Verlag, 1974).Google Scholar
29. Zalcstein, Y., Locally testable semigroups, Semigroup Forum 5 (1973), 216–227.Google Scholar