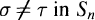Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
de Luca, Aldo
and
Varricchio, Stefeno
1990.
A note on ω-permutable semigroups.
Semigroup Forum,
Vol. 40,
Issue. 1,
p.
153.
Justin, J
and
Pirillo, G
1991.
Shirshov's theorem and ω-permutability of semigroups.
Advances in Mathematics,
Vol. 87,
Issue. 2,
p.
151.
Curzio, Mario
Longobardi, Patrizia
Maj, Mercede
and
Rhemtulla, Akbar
1992.
Groups with many rewritable products.
Proceedings of the American Mathematical Society,
Vol. 115,
Issue. 4,
p.
931.
Justin, Jacques
and
Pirillo, Giuseppe
1992.
Intracommutativity properties for groups and semigroups.
Journal of Algebra,
Vol. 153,
Issue. 2,
p.
424.
Longobardi, Patrizia
and
Maj, Mercede
1993.
Some remarks onP n-sequenceable groups.
Archiv der Mathematik,
Vol. 60,
Issue. 1,
p.
15.
Kelarev, A.V.
and
Shumyatsky, P.V.
1995.
Soluble and linear repetitive groups.
Bulletin of the Australian Mathematical Society,
Vol. 52,
Issue. 2,
p.
253.
de Luca, Aldo
and
Varricchio, Stefano
1997.
Handbook of Formal Languages.
p.
747.