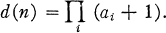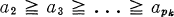Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Nicolas, J. L.
1975.
Répartition Modulo 1.
Vol. 475,
Issue. ,
p.
113.
Robin, Guy
1980.
Sur un probleme d'optimisation en nombres entiers.
Mathematische Operationsforschung und Statistik. Series Optimization,
Vol. 11,
Issue. 3,
p.
403.
Robin, G.
1983.
Méthodes d'optimisation pour un problème de théorie des nombres.
RAIRO. Informatique théorique,
Vol. 17,
Issue. 3,
p.
239.
Nicolas, J. L.
and
Robin, G.
1983.
Majorations Explicites Pour le Nombre de Diviseurs de N.
Canadian Mathematical Bulletin,
Vol. 26,
Issue. 4,
p.
485.
Erdős, P.
Nicolas, J.-L.
and
Sárközy, A.
1998.
Analytic and Elementary Number Theory.
Vol. 1,
Issue. ,
p.
225.
Nicolas, Jean Louis
2005.
Some Open Questions.
The Ramanujan Journal,
Vol. 9,
Issue. 1-2,
p.
251.
Brown, Ron
2006.
The minimal number with a given number of divisors.
Journal of Number Theory,
Vol. 116,
Issue. 1,
p.
150.
Narkiewicz, Władysław
2012.
Rational Number Theory in the 20th Century.
p.
13.
Andrews, George E.
and
Berndt, Bruce C.
2012.
Ramanujan's Lost Notebook.
p.
359.
Murty, M. Ram
and
Murty, V. Kumar
2013.
The Mathematical Legacy of Srinivasa Ramanujan.
p.
135.
Zhang, Zhenxiang
2014.
Estimating the counts of Carmichael and Williams numbers with small multiple seeds.
Mathematics of Computation,
Vol. 84,
Issue. 291,
p.
309.
Berman, Rachel N.
and
Roth, Ron M.
2020.
On the Number of Factorizations of Polynomials over Finite Fields.
p.
1.
Berman, Rachel N.
and
Roth, Ron M.
2021.
On the number of factorizations of polynomials over finite fields.
Journal of Combinatorial Theory, Series A,
Vol. 182,
Issue. ,
p.
105462.
Nicolas, Jean-Louis
2022.
Highly composite numbers and the Riemann hypothesis.
The Ramanujan Journal,
Vol. 57,
Issue. 2,
p.
507.