Hostname: page-component-cd9895bd7-q99xh Total loading time: 0 Render date: 2024-12-26T18:59:29.580Z Has data issue: false hasContentIssue false
  • English
  • Français

Ratio Limit Theorems

Published online by Cambridge University Press:  20 November 2018

A. G. Mucci
A. G. Mucci*
Affiliation:
University of Maryland, College Park, Maryland
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let be an adapted sequence of integrable random variables on the probability space 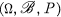. Let us set .The following result can be immediately derived from Brown [2]:

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 28 , Issue 2 , 01 April 1976 , pp. 403 - 407
Copyright
Copyright © Canadian Mathematical Society 1976

References

1. Breiman, L., Optimal gambling systems for favorable games, Fourth Berkeley Symposium on Probability and Mathematical Statistics, Vol. 1 (1961), 65–78.Google Scholar
2. Brown, B. M., A conditional setting for some theorems associated with the strong law, Z. Wahrscheinlichkeitstheorie 19 (1971), 274–280.Google Scholar
3. Dubins, L. E. and Freedman, D. A., A sharper form of the Borel-Cantelli lemma and the strong law, Annals of Mathematical Statistics 36 (1965), 800–807.Google Scholar
4. Freedman, D. A., Another note of the Borel-Cantelli lemma and the strong law with the Poisson approximation as a by-product, Annals of Probability 1 (1973), 910–925.Google Scholar
5. Stout, W. F., Almost sure convergence (Academic Press, 1974).Google Scholar