Hostname: page-component-586b7cd67f-t8hqh Total loading time: 0 Render date: 2024-11-28T17:55:09.731Z Has data issue: false hasContentIssue false
  • English
  • Français

Quasi-Multipliers and Embeddings of Hilbert C*-Bimodules

Published online by Cambridge University Press:  20 November 2018

Lawrence G. Brown ,
James A. Mingo  and
Nien-Tsu Shen
Lawrence G. Brown
Affiliation:
Department of Mathematics, Purdue University, West Lafayette, Indiana 47907, U.S.A., e-mail: [email protected]
James A. Mingo
Affiliation:
Department of Mathematics, Queen's University Kingston, Ontario K7L 3N6, e-mail: [email protected]
Nien-Tsu Shen
Affiliation:
MicroModule Systems 10500-A Ridgeview Court Cupertino, California 95014-0736, U.S.A., e-mail: [email protected]
Rights & Permissions [Opens in a new window]

Abstract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

This paper considers Hilbert C*-bimodules, a slight generalization of imprimitivity bimodules which were introduced by Rieffel [20]. Brown, Green, and Rieffel [7] showed that every imprimitivity bimodule X can be embedded into a certain C*-algebra L, called the linking algebra of X. We consider arbitrary embeddings of Hilbert C*-bimodules into C*-algebras; i.e. we describe the relative position of two arbitrary hereditary C*-algebras of a C*-algebra, in an analogy with Dixmier's description [10] of the relative position of two subspaces of a Hilbert space.

The main result of this paper (Theorem 4.3) is taken from the doctoral dissertation of the third author [22], although the proof here follows a different approach. In Section 1 we set out the definitions and basic properties (mostly folklore) of Hilbert C*-bimodules. In Section 2 we show how every quasi-multiplier gives rise to an embedding of a bimodule. In Section 3 we show that , the enveloping C*-algebra of the C*-algebraA with its product perturbed by a positive quasi-multiplier 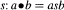, is isomorphic to the closure 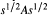(Proposition 3.1). Section 4 contains the main theorem (4.3), and in Section 5 we explain the analogy with the relative position of two subspaces of a Hilbert spaces and present some complements.

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 46 , Issue 6 , 01 December 1994 , pp. 1150 - 1174
Copyright
Copyright © Canadian Mathematical Society 1994

References

1. Akemann, C. and Pedersen, G. K., Complications of semi-continuity in C*-algebra theory, Duke Math. J. 40(1973), 785–795.Google Scholar
2. Blackadar, B., K-theoryfor Operator Algebras, Springer-Verlag, New York, 1986.Google Scholar
3. Blackadar, B. and Handelman, D., Dimension functions and traces on C*-algebras, J. Funct. Analysis 45(1982),297–340.Google Scholar
4. Brown, L. G., Stable Isomorphism of Hereditary Subalgebras of C*-algebras, Pacific J. Math. 71(1977), 335–348.Google Scholar
5. Brown, L. G., Close hereditary C*-subalgebras and the structure of quasi-multipliers, M.S.R.I. (1211-85), 1985, preprint.Google Scholar
6. Brown, L. G., Semicontinuity and Multipliers of C*-algebras, Canad. J. Math. XL(1988), 865–988.Google Scholar
7. Brown, L. G., Green, P., and Rieffel, M. A., Stable Isomorphism and Strong Morita Equivalence of C*-algebras, Pacific J. Math. 71(1977), 349–363.Google Scholar
8. Choi, M.-D. and Effros, E. G., Injectivity and Operator Spaces, J. Funct. Analysis 24(1977), 156–209.Google Scholar
9. Davis, C. and Kahane, W. M., The rotation of eigenvalues by a perturbation III, SIAM J. Numer. Anal. 7(1970), 1–46.Google Scholar
10. Dixmier, J., Position relative de deux variétés linéaires fermées dans un espace de Hilbert, Rev. Sci. 86(1948), 387–399.Google Scholar
11. Dixmier, J. , C*-algebras, North Holland, Amsterdam, 1977.Google Scholar
12. Halmos, P., Two Subspaces, Trans. Amer. Math. Soc. 144(1969), 381–389.Google Scholar
13. Kadison, R. V. and Ringrose, J., Fundamentals of the Theory of Operator Algebras, vol. II, Academic Press, Orlando, 1986.Google Scholar
14. Kasparov, G. G., Hilbert C*-modules; Theorems of Stinespring and Voiculescu, J. Operator Theory 4(1980), 133–150.Google Scholar
15. Krein, M. G., Krasnosel'skiĭ, M. A., and Mil'Dman, D.P., Defect Numbers of linear operators in Banach space and some geometrical problems, Sb. Trudov Inst. Mat. Akad. Nauk Ukr.SSR 11(1948), 97–112, (in Russian).Google Scholar
16. Paschke, W., Inner Product Modules over B*-algebras, Trans. Amer. Math. Soc. 182(1973), 443–468.Google Scholar
17. Pedersen, G. K., C*-algebras and Their Automorphisms, Academic Press, London, 1979.Google Scholar
18. Pedersen, G. K., Measure theory for C*-algebras II, Math. Scand. 22(1968), 63–74.Google Scholar
19. Raeburn, I. and Sinclair, A., The C*-algebra generated by two projections, Math. Scand. 65(1989), 278–290.Google Scholar
20. Rieffel, M. A., Induced Representations of C*-algebras, Adv. in Math. 13(1974), 176–257.Google Scholar
21. Sakai, S., C*-algebras and W*-algebras, Springer-Verlag, Berlin-Heidelberg-New York, 1971.Google Scholar
22. Shen, N.-T., Embeddings of Hilbert Bimodules, Doctoral dissertation, Purdue University, 1982.Google Scholar