Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Anstee, R.P.
1983.
The network flows approach for matrices with given row and column sums.
Discrete Mathematics,
Vol. 44,
Issue. 2,
p.
125.
Nosov, V. A.
Sachkov, V. N.
and
Tarakanov, V. E.
1985.
Combinatorial analysis (nonnegative matrices, algorithmic problems).
Journal of Soviet Mathematics,
Vol. 29,
Issue. 1,
p.
1051.
Marola, Giovanni
1988.
An algorithm for finding the number of (0, 1, 2,…, l)-matrices with prefixed row sum and column sum vectors.
International Journal of Computer Mathematics,
Vol. 23,
Issue. 2,
p.
125.
Michael, T.S.
1991.
The structure matrix and a generalization of Ryser's maximum term rank formula.
Linear Algebra and its Applications,
Vol. 145,
Issue. ,
p.
21.
Chen, William Y.C
1992.
Integral matrices with given row and column sums.
Journal of Combinatorial Theory, Series A,
Vol. 61,
Issue. 2,
p.
153.
Michael, T.S.
1993.
The structure matrix of the class of r-multigraphs with a prescribed degree sequence.
Linear Algebra and its Applications,
Vol. 183,
Issue. ,
p.
155.
Aharoni, R.
Herman, G.T.
and
Kuba, A.
1997.
Binary vectors partially determined by linear equation systems.
Discrete Mathematics,
Vol. 171,
Issue. 1-3,
p.
1.
Qian, Jianguo
1999.
On the upper bound of the diameter of interchange graphs.
Discrete Mathematics,
Vol. 195,
Issue. 1-3,
p.
277.
Kuba, Attila
and
Herman, Gabor T.
1999.
Discrete Tomography.
p.
3.
Kaneko, Akira
and
Nagahama, Rina
2006.
Discrete Geometry for Computer Imagery.
Vol. 4245,
Issue. ,
p.
110.
Brualdi, R.A.
and
Dahl, G.
2007.
Advances in Discrete Tomography and Its Applications.
p.
113.
Miller, Jeffrey W.
2013.
Reduced criteria for degree sequences.
Discrete Mathematics,
Vol. 313,
Issue. 4,
p.
550.
Lari, Isabella
Ricca, Federica
and
Scozzari, Andrea
2014.
Bidimensional allocation of seats via zero-one matrices with given line sums.
Annals of Operations Research,
Vol. 215,
Issue. 1,
p.
165.
Chen, Wei
Mo, Yanfang
Qiu, Li
and
Varaiya, Pravin
2016.
Constrained (0,1)-matrix completion with a staircase of fixed zeros.
Linear Algebra and its Applications,
Vol. 510,
Issue. ,
p.
171.
Burstein, David
and
Rubin, Jonathan
2017.
Sufficient Conditions for Graphicality of Bidegree Sequences.
SIAM Journal on Discrete Mathematics,
Vol. 31,
Issue. 1,
p.
50.
Blondin Massé, Alexandre
de Carufel, Julien
Goupil, Alain
Lapointe, Mélodie
Nadeau, Émile
and
Vandomme, Élise
2018.
Leaf realization problem, caterpillar graphs and prefix normal words.
Theoretical Computer Science,
Vol. 732,
Issue. ,
p.
1.
Means, Shawn A.
Bläsche, Christian
Laing, Carlo R.
and
Berthouze, Luc
2020.
A permutation method for network assembly.
PLOS ONE,
Vol. 15,
Issue. 10,
p.
e0240888.
Bar-Noy, Amotz
Choudhary, Keerti
Peleg, David
and
Rawitz, Dror
2020.
Efficiently Realizing Interval Sequences.
SIAM Journal on Discrete Mathematics,
Vol. 34,
Issue. 4,
p.
2318.
Bar-Noy, Amotz
Böhnlein, Toni
Peleg, David
Perry, Mor
and
Rawitz, Dror
2021.
Combinatorial Algorithms.
Vol. 12757,
Issue. ,
p.
3.
Laing, Carlo R.
Bläsche, Christian
and
Means, Shawn
2021.
Dynamics of Structured Networks of Winfree Oscillators.
Frontiers in Systems Neuroscience,
Vol. 15,
Issue. ,
 to be the set of m × n (0, 1)-matrices with ith row sum ri and jth column sum sj for 1 ≦ i ≦ m and 1 ≦ j ≦ n.
to be the set of m × n (0, 1)-matrices with ith row sum ri and jth column sum sj for 1 ≦ i ≦ m and 1 ≦ j ≦ n. to be nonempty [9 , 14]. From R, we form an m × n (0, 1)-matrix Ā as follows. The ith row sum of Ā is ri and the 1‘s are as far to the left as possible. Let
to be nonempty [9 , 14]. From R, we form an m × n (0, 1)-matrix Ā as follows. The ith row sum of Ā is ri and the 1‘s are as far to the left as possible. Let 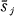 be the jth column sum of A. We define the sequence
be the jth column sum of A. We define the sequence  to be the conjugate of the sequence (ri).
to be the conjugate of the sequence (ri).

