Power Residues of Fourier Coefficients of Modular Forms

Abstract

Let 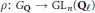$\rho :\,{{G}_{Q}}\,\to \,\text{G}{{\text{L}}_{n}}\left( {{Q}_{\ell }} \right)$ be a motivic $\ell $-adic Galois representation. For fixed 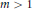$m\,>\,1$ we initiate an investigation of the density of the set of primes 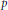$p$ such that the trace of the image of an arithmetic Frobenius at 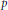$p$ under $\rho $ is an 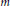$m$-th power residue modulo 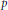$p$. Based on numerical investigations with modular forms we conjecture (with Ramakrishna) that this density equals $1/m$ whenever the image of $\rho $ is open. We further conjecture that for such $\rho $ the set of these primes 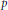$p$ is independent of any set defined by Cebatorev-style Galois-theoretic conditions (in an appropriate sense). We then compute these densities for certain 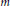$m$ in the complementary case of modular forms of 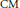$\text{CM}$-type with rational Fourier coefficients; our proofs are a combination of the Cebatorev density theorem (which does apply in the 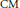$\text{CM}$ case) and reciprocity laws applied to Hecke characters. We also discuss a potential application (suggested by Ramakrishna) to computing inertial degrees at 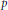$p$ in abelian extensions of imaginary quadratic fields unramified away from 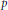$p$.