Hostname: page-component-cd9895bd7-p9bg8 Total loading time: 0 Render date: 2024-12-25T06:25:53.506Z Has data issue: false hasContentIssue false
  • English
  • Français

Polynomial Approximation and Growth of Generalized Axisymmetrig Potentials

Published online by Cambridge University Press:  20 November 2018

Peter A. McCoy
Peter A. McCoy*
Affiliation:
United States Naval Academy, Annapolis, Maryland 21402
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Generalized axisymmetric potentials Fα (GASP) are regular solutions to the generalized axisymmetric potential equation

(1.1)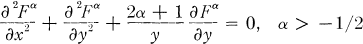

in some neighborhood Ω of the origin where they are subject to the initial data

(1.2)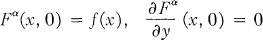

along the singular line y = 0. In Ω, these potentials may be uniquely expanded in terms of the complete set of normalized ultraspherical polynomials

(1.3)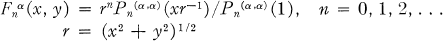

defined from the symmetric Jacobi polynomials Pn(α, α)(ξ) of degree n with parameter α as Fourier series

(1.4)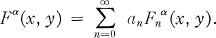

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 31 , Issue 1 , 01 February 1979 , pp. 49 - 59
Copyright
Copyright © Canadian Mathematical Society 1979

References

1. Askey, R., Orthogonal polynomials and special functions, Regional Conference Series in Applied Math., SLAM, Philadelphia, 1975.Google Scholar
2. Bergman, S., Integral operators in theory of linear partial differential equations, Ergebnisse der Math und Grenzebiete, Heft 23 (Springer-Verlag, New York, 1961).Google Scholar
3. Bernstein, S. N., Leçon sur les propriétés extrémales et la meilleure approximation des jonctions analytiques d'une variable réelle (Gauthier-Villars, Paris, 1926).Google Scholar
4. Dienes, P., The taylor series (Dover Publications, New York, 1957).Google Scholar
5. Fryant, A. J., Contributions to axisymmetric potential theory, Ph.D. Thesis, University of Wisconsin, Milwaukee, June 1975.Google Scholar
6. Fryant, A. J., Growth and complete sequences of generalized axisymmetric potentials, J. Approx. Theor. 19 (1977), 361–370.Google Scholar
7. Gilbert, R. P., Function theoretic methods in partial differential equations, Math, in Science and Engineering, vol. 54 (Academic Press, New York, 1969).Google Scholar
8. Gilbert, R. P., Constructive methods for elliptic equations, Lecture Notes in Mathematics, vol. 365 (Springer-Verlag, New York, 1974).Google Scholar
9. Gilbert, R. P., Some inequalities for generalized axially symmetric potentials with entire and meromorphic associates, Duke J. Math. 32 (1965), 239–246.Google Scholar
10. Grenander, U. and Szego, G., Toeplitz forms and their applications, California Monographs in Math. Science (U. of Calif. Press, Berkeley and Los Angeles, 1958).Google Scholar
11. Hille, E., Analytic function theory, vol. 2 (Blaisdell, Waltham, Mass., 1962).Google Scholar
12. Levin, B. Ja., Distribution of zeros of entire functions, Trans, of Math. Monographs, vol. 5 (Amer. Math. Soc, Providence, 1964).Google Scholar
13. Marden, M., Value distribution of harmonic polynomials in several real variables, Trans. Amer. Math. Soc. 159 (1971), 137–154.Google Scholar
14. Marden, M., Axisymmetric harmonic interpolation polynomials in RN, Trans. Amer. Math. Soc. 196 (1974), 385–402.Google Scholar
15. Marden, M., Geometry of polynomials, 2nd ed., Math. Surveys, No. 3 (Amer. Math. Soc, Providence, R.I., 1966).Google Scholar
16. McCoy, P. A., On the zeros of generalized axisymmetric potentials, Proc. Amer. Math. Soc. 61 (1976), 54–58.Google Scholar
17. McCoy, P. A., Extremal properties of real axially symmetric harmonic functions in Ez, Proc. Amer. Math Soc. 67 (1977), 248–252.Google Scholar
18. Meinardus, G., Approximation of functions: theory and numerical methods, Springer Tracts in Natural Philosophy, vol. 13 (Springer-Verlag, New York, 1967).Google Scholar
19. Muckenhoupt, B. and Stein, E. M., Classical expansions and their relation to conjugate harmonic functions, Trans. Amer. Math. Soc. 118 (1965), 17–91.Google Scholar
20. Rivlin, T. J., The Chebyshev polynomials (John Wiley and Sons, New York, 1974).Google Scholar
21. Tsuji, M., Potential theory in modern function theory (Maruzen Co., Ltd., Tokyo, 1959).Google Scholar
22. Varga, R. S., On an extension of a result of S. N. Bernstein, J. Approx. Theory (1968), 176–179.Google Scholar
23. Whittaker, E. T., and Watson, G. N., A course of modern analysis (Cambridge Univ. Press, 1969).Google Scholar