Hostname: page-component-586b7cd67f-dlnhk Total loading time: 0 Render date: 2024-11-24T06:16:27.788Z Has data issue: false hasContentIssue false
  • English
  • Français

Permanent Preservers on the Space of Doubly Stochastic Matrices

Published online by Cambridge University Press:  20 November 2018

B. N. Moyls ,
Marvin Marcus  and
Henryk Minc
B. N. Moyls
Affiliation:
University of British Columbia
Marvin Marcus
Affiliation:
University of British Columbia
Henryk Minc
Affiliation:
University of British Columbia
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

Let Mn be the linear space of n-square matrices with real elements. For a matrix X = (xij) ∈ Mn the permanent is defined by

where σ runs over all permutations of 1, 2, …, n. In (2) Marcus and May determine the nature of all linear transformations T of Mn into itself such that per T(X) = per X for all X ∈ Mn. For such a permanent preserver T, and for n < 3, there exist permutation matrices P, Q, and diagonal matrices D, L in Mn, such that per DL = 1 and either

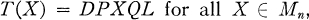
or

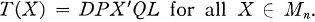
Here X′ denotes the transpose of X. In the case n = 2, a different type of transformation is also possible.

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 14 , 1962 , pp. 190 - 194
Copyright
Copyright © Canadian Mathematical Society 1962

References

1. Marcus, Marvin, Some properties and applications of doubly stochastic matrices, Amer. Math. Monthly, 67 (1960), 215–221.Google Scholar
2. Marcus, Marvin and May, F. C., The permanent function, Can. J. Math., 14 (1962) 177–190.Google Scholar
3. Marcus, Marvin and Newman, Morris, On the minimum of the permanent of a doubly stochastic matrix, Duke Math. J., 26 (1959), 61–72.Google Scholar