Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by Crossref.
Naito, Manabu
and
Yoshida, Norio
1978.
Oscillation theorems for semilinear elliptic differential operators.
Proceedings of the Royal Society of Edinburgh: Section A Mathematics,
Vol. 82,
Issue. 1-2,
p.
135.
Swanson, C. A.
1979.
Semilinear Second Order Elliptic Oscillation.
Canadian Mathematical Bulletin,
Vol. 22,
Issue. 2,
p.
139.
Wong, Pui-Kei
1979.
Sturmian theory of second-order elliptic differential equations and inequalities.
Journal of Mathematical Analysis and Applications,
Vol. 72,
Issue. 2,
p.
551.
Müller‐pfeiffer, E.
1979.
Kriterien für die Oszillation von elliptischen Differentialgleichungen höherer Ordnung.
Mathematische Nachrichten,
Vol. 90,
Issue. 1,
p.
239.
Etgen, Garret J.
and
Lewis, Roger T.
1980.
The Oscillation of Elliptic Systems.
Mathematische Nachrichten,
Vol. 94,
Issue. 1,
p.
43.
Ahlbrandt, Calvin D.
Hinton, Don B.
and
Lewis, Roger T.
1983.
Ordinary Differential Equations and Operators.
Vol. 1032,
Issue. ,
p.
1.


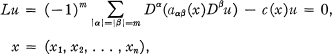

 where each α
where each α 