Hostname: page-component-cd9895bd7-7cvxr Total loading time: 0 Render date: 2024-12-25T07:04:50.642Z Has data issue: false hasContentIssue false
  • English
  • Français

Opérations En K-Théorie Algébrique

Published online by Cambridge University Press:  20 November 2018

Christophe Soulé
Christophe Soulé*
Affiliation:
Université Paris VII, Paris, France
Rights & Permissions [Opens in a new window]

Extract

Core share and HTML view are not available for this content. However, as you have access to this content, a full PDF is available via the ‘Save PDF’ action button.

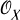C'est pour étendre le théorème de Riemann-Roch à un morphisme projectif arbitraire que Grothendieck a introduit le groupe K(X) (noté aujourd'hui K0(X)), construit à l'aide des -modules localement libres sur un schéma X[14]. La somme directe et le produit tensoriel de modules font de K0(X) un anneau, et les opérations de puissances extérieures lui fournissent une structure supplémentaire, que Grothendieck appelle λ-anneau. Un λ-anneau est muni d'une filtration décroissante, la γ-filtration, et un des principaux résultats de Grothendieck est que, si X est lisse sur un corps, le groupe

est isomorphe, à la torsion près, au groupe de Chow CH1(X) des cycles de codimension i sur X, modulo l'équivalence linéaire.

Type
Research Article
Information
Canadian Journal of Mathematics , Volume 37 , Issue 3 , 01 June 1985 , pp. 488 - 550
Copyright
Copyright © Canadian Mathematical Society 1985

References

Bibliographie

1. Anderson, D. W., Relationship among K-theories, Lee. Notes in Maths. 341 (1972), 57–72.Google Scholar
2. Atiyah, M. and Tall, D. O., Group representations, λ-rings and J-homomorphism, Topology 5 (1969), 253–297.Google Scholar
3. Bass, H., Algebraic K-theory (Benjamin, New York, 1968).Google Scholar
4. Baum, P., Fulton, W. and MacPherson, R., Riemann-Roch for singular varieties, Publ. Math. IHES 45 (1975), 101–145.Google Scholar
5. Beilinson, A., Régulateurs supérieurs et valeurs de fonction L (en russe), prépublication, (1983).Google Scholar
6. Bloch, S. and Ogus, A., Gersten's conjecture and the homology of schemes, Ann. Sci. Ec. Norm. Sup. 7 (1974), 181–202.Google Scholar
7. Bousfield, A. K. and Kan, D. M., Homotopy limits, completions and localizations, Lee. Notes in Maths. 304 (1975).Google Scholar
8. Brown, K. S., Abstract homotopy theory and generalized sheaf cohomology, Trans. Amer. Math. Soc. 186 (1974), 419–458.Google Scholar
9. Brown, K. S., and Gersten, S. M., Algebraic K-theory as generalized cohomology, Lee. Notes in Maths. 341 (1973), 266–292.Google Scholar
10. Dayton, B. and Weibel, C., A spectral sequence for the K-theory of affine glued schemes, Lee. Notes in Maths. 854 (1980), 24–92.Google Scholar
11. Fulton, W., Rational equivalence on singular varieties, Publ. Math. IHES 45 (1975), 147–167.Google Scholar
12. Gillet, H., Riemann-Roch theorems for higher algebraic K-theory, Advanees in Maths. 40 (1981), 203–289.Google Scholar
13. Gillet, H. et Soulé, C., en préparation.Google Scholar
14. Grothendieck, A. et al., Théorie des intersections et théorème de Riemann-Roch, Lee. Notes in Maths. 225, SGA 6 (1971).Google Scholar
15. Hiller, H., λ-rings and algebraic K-theory, Journal of Pure and Applied Algebra 20 (1981), 241–266.Google Scholar
16. Kratzer, C., λ-structure en K-théorie algébrique, Comm. Math. Helv. 55 (1970), 233–254.Google Scholar
17. Kratzer, C., Opérations d'Adams et représentations de groupes, Ensnt. Math II 6 (1980), 141–154.Google Scholar
18. Landsburg, S., Relative cycles and algebraic K-theory, prépublication (1983).Google Scholar
19. Loday, J-L., K-théorie algébrique et représentations de groupes, Ann. Sci. Ec. Norm. Sup. 9 (1976), 309–377.Google Scholar
20. Manin, Y., Lectures on the K-functor in algebraic geometry, Russ. Math. Surveys 24 (1969), 1–89.Google Scholar
21. Merkurjev, A. S. et Suslin, A. A., K-cohomologie des variétés de Severi-Brauer et symboles des restes normiques (en russe), Izv. Akad. Nauk SSSR 46 (1982), 1011–1046.Google Scholar
22. Milnor, J., Introduction to algebraic K-theory, Annals of Maths. Studies 72 (1971).Google Scholar
23. Milnor, J. and Stasheff, J., Characteristic classes, Annals of Maths. Studies 76 (1974).Google Scholar
24. Milnor, J. and Moore, J., On the structure of Hopf algebras, Ann. Math. 81 (1965), 211–264.Google Scholar
25. Quillen, D., Higher algebraic K-theory I, Lee. Notes in Maths. 341 (1973), 85–147.CrossRefGoogle Scholar
26. Quillen, D., Homotopical algebra, Lee. Notes in Maths. 43 (1967).CrossRefGoogle Scholar
27. Serre, J.-P., Groupes de Grothendieck des schémas en groupes déployés, Publ. Math. IHES 34 (1968), 37–52.Google Scholar
28. Schechtman, V. V., Riemann-Roch's theorem in algebraic K-theory, Dep. VINITI, (1979).Google Scholar
29. Soulé, C., Operations on étale K-theory. Applications, Lee. Notes in Maths 966 (1982), 271–303.Google Scholar
30. Soulé, C., Groupes de Chow et K-théorie de variétés sur un corps fini, Math. Ann. 268 (1984), 317–345.Google Scholar
31. Soulé, C., K-théorie et zéros aux points entiers de fonctions zêta, Proc. Congrès Int. des Math., Varsovie (1982).Google Scholar
32. Stienstra, J., Cartier-Dieudonné theory for the Chow groups, prépublication, Leiden (1983).Google Scholar
33. Suslin, A. A., Stability in algebraic K-theory, Lee. Notes in Maths 966 (1982), 304–333.CrossRefGoogle Scholar
34. Suslin, A. A., Mennicke symbols and their applications in the K-theory of fields, Lee. Notes in Maths. 966 (1982), 334–356.Google Scholar
35. Suslin, A. A., Homology of GLn, characteristic classes and Milnor K-theory, Lee. Notes in Maths. 1046 (1984), 357–375.Google Scholar
36. Weibel, C., KV-theory of categories, Transactions AMS. 267 (1981), 621–635.Google Scholar