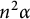Hostname: page-component-586b7cd67f-tf8b9 Total loading time: 0 Render date: 2024-11-24T05:48:31.789Z Has data issue: false hasContentIssue false
  • English
  • Français

On the triple correlations of fractional parts of $n^2\alpha $

Part of: Probabilistic theory: distribution modulo $1$; metric theory of algorithms Exponential sums and character sums

Published online by Cambridge University Press:  04 May 2021

Niclas Technau  and
Aled Walker
Niclas Technau
Affiliation:
University of Wisconsin-Madison, Madison, USA e-mail: [email protected]
Aled Walker*
Affiliation:
Trinity College, Cambridge, UK
*
e-mail: [email protected]
Get access Rights & Permissions [Opens in a new window]

Abstract

For fixed $\alpha \in [0,1]$ , consider the set 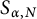$S_{\alpha ,N}$ of dilated squares 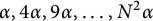$\alpha , 4\alpha , 9\alpha , \dots , N^2\alpha \, $ modulo 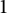$1$ . Rudnick and Sarnak conjectured that, for Lebesgue, almost all such 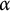$\alpha $ the gap-distribution of 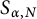$S_{\alpha ,N}$ is consistent with the Poisson model (in the limit as N tends to infinity). 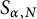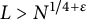In this paper, we prove a new estimate for the triple correlations associated with this problem, establishing an asymptotic expression for the third moment of the number of elements of $S_{\alpha ,N}$ in a random interval of length $L/N$ , provided that $L> N^{1/4+\varepsilon }$ . 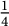The threshold of $\tfrac {1}{4}$ is substantially smaller than the threshold of 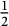$\tfrac {1}{2}$ (which is the threshold that would be given by a naïve discrepancy estimate).

Unlike the theory of pair correlations, rather little is known about triple correlations of the dilations $(\alpha a_n \, \text {mod } 1)_{n=1}^{\infty } $ for a nonlacunary sequence 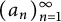$(a_n)_{n=1}^{\infty } $ of increasing integers. This is partially due to the fact that the second moment of the triple correlation function is difficult to control, and thus standard techniques involving variance bounds are not applicable. We circumvent this impasse by using an argument inspired by works of Rudnick, Sarnak, and Zaharescu, and Heath-Brown, which connects the triple correlation function to some modular counting problems.

In Appendix B, we comment on the relationship between discrepancy and correlation functions, answering a question of Steinerberger.

Keywords

Triple correlationsuniform distributiondiscrepancy

MSC classification

Primary: 11K06: General theory of distribution modulo $1$ 11K60: Diophantine approximation
Secondary: 11L05: Gauss and Kloosterman sums; generalizations
Type
Article
Information
Canadian Journal of Mathematics , Volume 74 , Issue 5 , October 2022 , pp. 1347 - 1384
Check for updates
Copyright
© Canadian Mathematical Society 2021

Access options

Get access to the full version of this content by using one of the access options below. (Log in options will check for institutional or personal access. Content may require purchase if you do not have access.)

Footnotes

While the work toward this paper was being carried out, NT was supported by the European Research Council (ERC) under the European Union’s Horizon 2020 research and innovation programme (Grant agreement No. 786758) and by the Austrian Science Fund (FWF): project J-4464. A.W. is supported by a Junior Research Fellowship from Trinity College Cambridge, and was also supported by a Post-Doctoral Fellowship at the Centre de Recherches Mathématiques.

References

Aistleitner, C. and Larcher, G., Metric results on the discrepancy of sequences ${\left({a}_n\alpha \right)}_{n\ge 1}$ modulo one for integer sequences ${\left({a}_n\right)}_{n\ge 1}$ of polynomial growth . Mathematika 62(2016), no. 2, 478–491.CrossRefGoogle Scholar
Aistleitner, C., Larcher, G., and Lewko, M., Additive energy and the Hausdorff dimension of the exceptional set in metric pair correlation problems. Israel J. Math. 222(2017), no. 1, 463–485.CrossRefGoogle Scholar
Beck, J., Probabilistic Diophantine approximation, I. Kronecker sequences. Ann. of Math. (2) 140(1994), no. 2, 449–502.CrossRefGoogle Scholar
Berry, M. V. and Tabor, M., Level clustering in the regular spectrum. Proc. Roy. Soc. Lond. A Math. Phy. Sci. 356(1977), no. 1686, 375–394.Google Scholar
Boca, F. and Zaharescu, A., Pair correlation of values of rational functions (mod p). Duke Math. J. 105(2000), no. 2, 267–307.Google Scholar
Chung, K.-L., An estimate concerning the Kolmogoroff limit distribution. Trans. Amer. Math. Soc. 67(1949), no. 1, 36–50.Google Scholar
El-Baz, D., Marklof, J., and Vinogradov, I., The two-point correlation function of the fractional parts of $\sqrt{n}$ is Poisson . Proc. Amer. Math. Soc. 143(2015), no. 7, 2815–2828.Google Scholar
Elkies, N. and McMullen, C., Gaps in $\sqrt{n}$ mod 1 and ergodic theory . Duke Math. J. 123(2004), no. 1, 95–139.CrossRefGoogle Scholar
Erdős, P. and Koksma, J., On the uniform distribution modulo 1 of sequences (f(n, ϑ)). Nederl. Akad. Wetensch. Proc. 52(1949), 851–854.Google Scholar
Eskin, A., Margulis, G., and Mozes, S., Quadratic forms of signature (2, 2) and eigenvalue spacings on rectangular 2-tori. Ann. of Math. (2) 161(2005), no. 2, 679–725.CrossRefGoogle Scholar
Harman, G., Metric number theory . London Mathematical Society Monographs New Series, 18, Clarendon Press, Oxford, UK, 1998.Google Scholar
Heath-Brown, D. R., Pair correlation for fractional parts of αn 2 . Math. Proc. Cambridge Philos. Soc. 148(2010), no. 3, 385–407.CrossRefGoogle Scholar
Iwaniec, H. and Kowalski, E., Analytic number theory . Vol. 53, American Mathematical Society, Providence, RI, 2004.Google Scholar
Khintchine, A., Über einen Satz der Wahrscheinlichkeitsrechnung. Fund. Math. 6(1924), no. 1, 9–20.CrossRefGoogle Scholar
Kuipers, L. and Niederreiter, H., Uniform distribution of sequences . Courier Corporation, North Chelmsford, MA, 2012.Google Scholar
Lutsko, C., Long-range correlations of sequence modulo 1. Preprint, 2020. arXiv:2007.09292 Google Scholar
Marklof, J., The Berry–Tabor conjecture . In: Casacuberta, C., Miró-Roig, R. M., Verdera, J., and Xambó-Descamps, S. (eds.), European Congress of Mathematics, Springer, New York, 2001, pp. 421–427.CrossRefGoogle Scholar
Marklof, J., Pair correlation densities of inhomogeneous quadratic forms. Ann. of Math. (2) 158(2003), no. 2, 419–471.Google Scholar
Montgomery, H., Ten lectures on the interface between analytic number theory and harmonic analysis. CBMS Regional Conference Series in Mathematics, 84, American Mathematical Society, Providence, RI, 1994.CrossRefGoogle Scholar
Rudnick, Z., Quantum chaos? Notices Amer. Math. Soc. 55(2008), no. 1, 32–34.Google Scholar
Rudnick, Z., A metric theory of minimal gaps. Mathematika 64(2018), no. 3, 628–636.CrossRefGoogle Scholar
Rudnick, Z. and Kurlberg, P., The distribution of spacings between quadratic residues. Duke J. Math. 100(1999), 211–242.Google Scholar
Rudnick, Z. and Sarnak, P., The pair correlation function of fractional parts of polynomials. Comm. Math. Phys. 194(1998), no. 1, 61–70.CrossRefGoogle Scholar
Rudnick, Z., Sarnak, P., and Zaharescu, A., The distribution of spacings between the fractional parts of n 2 α . Invent. Math. 145(2001), no. 1, 37–57.CrossRefGoogle Scholar
Rudnick, Z. and Zaharescu, A., The distribution of spacings between fractional parts of lacunary sequences. Forum Math. 14(2002), no. 5, 691–712.CrossRefGoogle Scholar
Sarnak, P., Values at integers of binary quadratic forms . In: Harmonic analysis and number theory (Montreal, PQ, 1996), CMS Conference Proceedings, 21, American Mathematical Society, Providence, RI, 1997, 181–203.Google Scholar
Steinerberger, S., Poissonian pair correlation and discrepancy. Indag. Math. 29(2018), no. 5, 1167–1178.CrossRefGoogle Scholar
Weiß, C. and Skill, T., Sequences with almost Poissonian pair correlations. Preprint, 2021. arXiv:1905.02760 Google Scholar
Weyl, H., Über die gleichverteilung von zahlen mod eins. Math. Ann. 77(1916), no. 3, 313–352.CrossRefGoogle Scholar
Zaharescu, A., Correlation of fractional parts of n 2 α . Forum Math. 15(2003), no. 1, 1–21.Google Scholar