Crossref Citations
This article has been cited by the following publications. This list is generated based on data provided by
Crossref.
Cheong, Daewoong
Koh, Doowon
and
Pham, Thang
2021.
Несимметричная оценка суммы множеств расстояний.
Труды Математического института имени В. А. Стеклова,
Vol. 314,
Issue. ,
p.
290.
Cheong, Daewoong
Koh, Doowon
and
Pham, Thang
2021.
An Asymmetric Bound for Sum of Distance Sets.
Proceedings of the Steklov Institute of Mathematics,
Vol. 314,
Issue. 1,
p.
279.
Koh, Doowon
and
Pham, Thang
2022.
A point-sphere incidence bound in odd dimensions and applications.
Comptes Rendus. Mathématique,
Vol. 360,
Issue. G6,
p.
687.
Koh, Doowon
and
Lee, Sujin
2022.
Averages and maximal averages over Product j-varieties in finite fields.
Finite Fields and Their Applications,
Vol. 79,
Issue. ,
p.
101984.
Pham, Minh-Quy
2023.
On the k-resultant modulus set problem on varieties over finite fields.
International Journal of Number Theory,
Vol. 19,
Issue. 03,
p.
569.
Koh, Doowon
2023.
Restriction estimates for the flat disks over finite fields.
Journal of Mathematical Analysis and Applications,
Vol. 518,
Issue. 2,
p.
126714.
Chang, Che-Jui
Mohammadi, Ali
Pham, Thang
and
Shen, Chun-Yen
2024.
Product of Sets on Varieties in Finite Fields.
Journal of Fourier Analysis and Applications,
Vol. 30,
Issue. 2,
Pham, Thang
2024.
Group action and L2-norm estimates of geometric problems.
Journal of Mathematical Analysis and Applications,
Vol. 531,
Issue. 1,
p.
127748.
Koh, Doowon
and
Pham, Thang
2025.
A spherical extension theorem and applications in positive characteristic.
Finite Fields and Their Applications,
Vol. 101,
Issue. ,
p.
102515.
 $L^{2}\to L^{r}$
extension conjecture for the zero radius sphere over finite fields. We also obtain the sharp
$L^{2}\to L^{r}$
extension conjecture for the zero radius sphere over finite fields. We also obtain the sharp 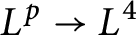 $L^{p}\to L^{4}$
extension estimate for non-zero radii spheres over finite fields, which improves the previous result of the first and second authors significantly.
$L^{p}\to L^{4}$
extension estimate for non-zero radii spheres over finite fields, which improves the previous result of the first and second authors significantly.